This is extremely similar to Srednicki Chapter 5 where he includes this picture (but sadly no completion relation similar to your text): 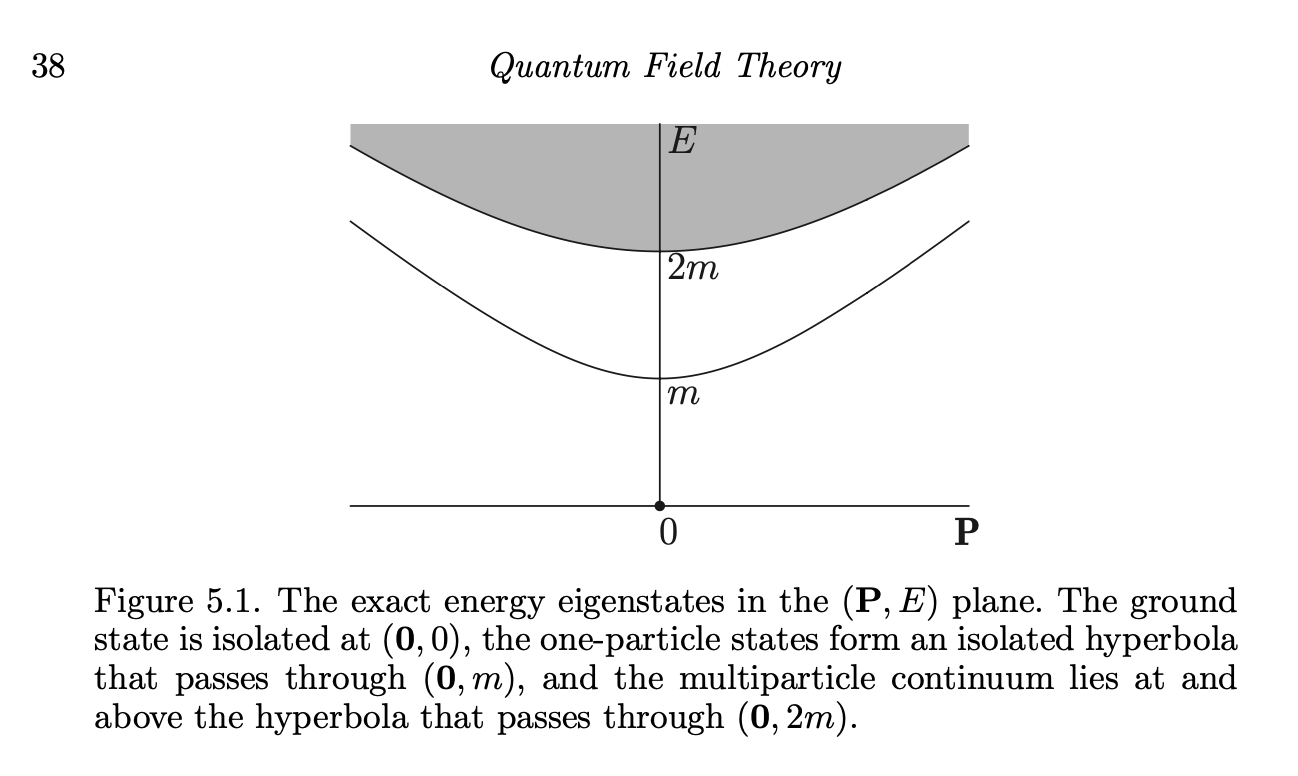
This picture shows the entire set of eigenstates where there is a single vacuum state, a line of one particle states and then a multi-particle continuum. Thus my understanding of your formula is that $|\lambda_p\rangle$ does not necessarily represent a single particle state. In fact, in private correspondence with the author, he suggested I think about the states in this context with one label for momentum and another label $n$ that captures everything else; this is $\lambda$ here. Therefore, the $\lambda$ sum represents summing over every possible $0$ momentum state and the integral represents summing over every boosted (by ${\bf p}$) counterpart for each $|\lambda_{p=0}\rangle$ state. This way we are capturing the entire basis for the Hilbert space so this is a fine completeness relation.
