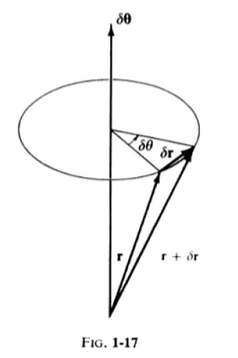
Hello fellow physicists,
I was trying to understand some behavior on rotating objects, specifically about the formula $\vec{v} = \vec{\omega} \times \vec{r}$.
The Book (Marion, J. B. (1965). Classical Dynamics: Of Particles and Systems (pp. 40-41). Academic Press Inc.) explains it pretty well, starting from the equation:
$\delta \vec{r} = \delta \vec{ \theta} \times \vec{r} \quad (1)$,
then dividing by $\delta t$;
$\dfrac{\delta \vec{r}}{\delta t} = \dfrac{\delta \vec{ \theta}}{\delta t} \times \vec{r}$,
and then making $\lim_{\delta \to 0}$;
$\vec{v} = \vec{\omega} \times \vec{r}$
but I don't quite catch where that particular equation (1) came from. The Marion book (on page 40) states that the proof is on "Marion (Ma65a, Section 2.5)", but I cannot find where is that referring to...
So I was wondering if someone could help me on the matter.
(I'll add an image that could help visualize the situation:)