You never push a heavy rope. It will hang down in a catenary shape and you need to always pull to keep it in place.
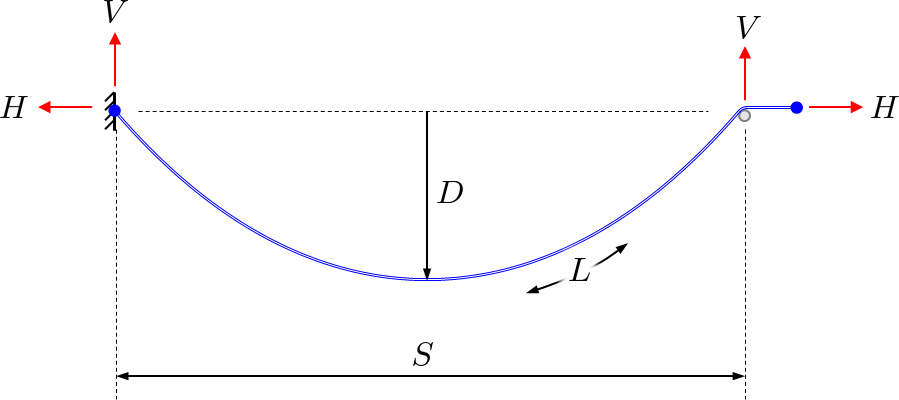
The amount you need to pull is given by the catenary equations. Consider the hanging cable with dimensions shown below:
Actually given the pull force $H$, the remaining dimensions and values are derived from the catenary equations. You need a measure of the weight of the rope, and it is given as $w = \frac{m g}{L}$ usually, that is $w$ is weight per unit length. Also you must know $S$, the span where the rope hangs from.
I have summarized the catenary equations below, as well as their parabolic approximations
| Quantity | Catenary | Approximation |
|---|---|---|
| Catenary Constant, $a$ | $ a = \frac{H}{w} $ | |
| Hanging Length, $L$ | $L = 2 a \,\sinh \left( \frac{S}{2 a} \right)$ | $L = S + \frac{w^2 S^3}{24 H^2}$ |
| Maximum Sag, $D$ | $D = a \left( \cosh \left( \frac{S}{2 a} \right) -1 \right)$ | $D = \frac{w S^2}{8 H}$ |
| Support Force, $V$ | $V = H \sinh \left( \frac{S}{2 a} \right) $ | $V = \frac{w S}{2} + \frac{w^3 S^3}{48 H^2}$ |
| Total Force, $T=\sqrt{H^2+V^2}$ | $T = H \cosh \left( \frac{S}{2 a} \right) $ | $T =H + \frac{w^2 S^2}{8 H}$ |