In my opinion, it's better to reason with a position-vs-time graph (a spacetime diagram).
To start you off, below is one using the usual convention where t runs along the horizontal axis
(although many relativity discussions use time t along the vertical axis).
https://www.desmos.com/calculator/c2mtuzt6ty
I was following you until...
Therefore, it takes two seconds for the light beam to 'pull ahead' of the vehicle by 300,000km.
In addition, it's not clear to me why you say
However, when I plug 0.5c into the time dilation formula, I find that the observer in the spacecraft does not measure one second to pass for every two seconds that pass for the observer outside.
In any case, I think it is best to draw a diagram to describe your scenario.
By the way, the arithmetic for calculations in relativity is easier if one chooses a relative velocities of $\frac{3}{5}c$ or $\frac{4}{5}c$, which lead to right triangles with pythagorean triples. (This does not happen for $\frac{1}{2}c$ or $0.99c$.)
The mathematics of time-dilation is related to trigonometry with adjacent side of a right-triangle. This fact and a diagram often help guide my physical intuition.
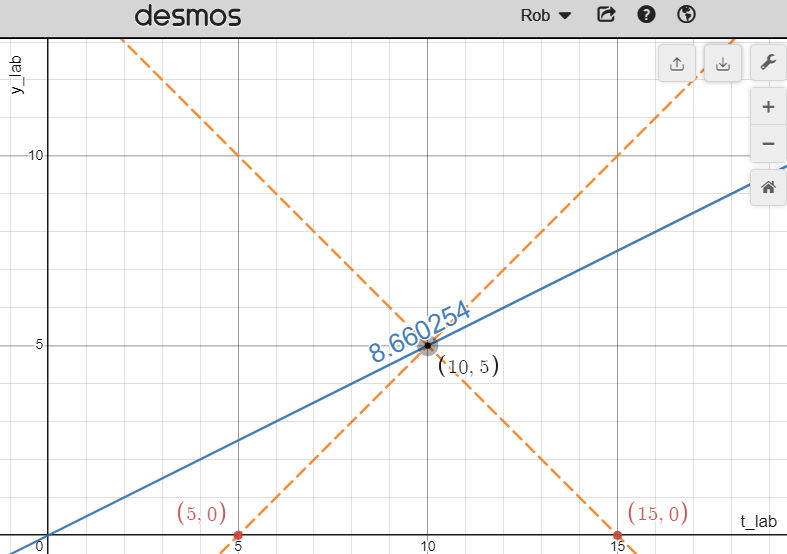
By the way, one way to get the 8.660254 as the elapsed time along the spaceship worldline is by $$\sqrt{(15)(5)}=10\left(\frac{\sqrt{3}}{2}\right)=8.660254...,$$ where $5$ and $15$ are ``radar-times'' from a radar measurement made by the lab.
