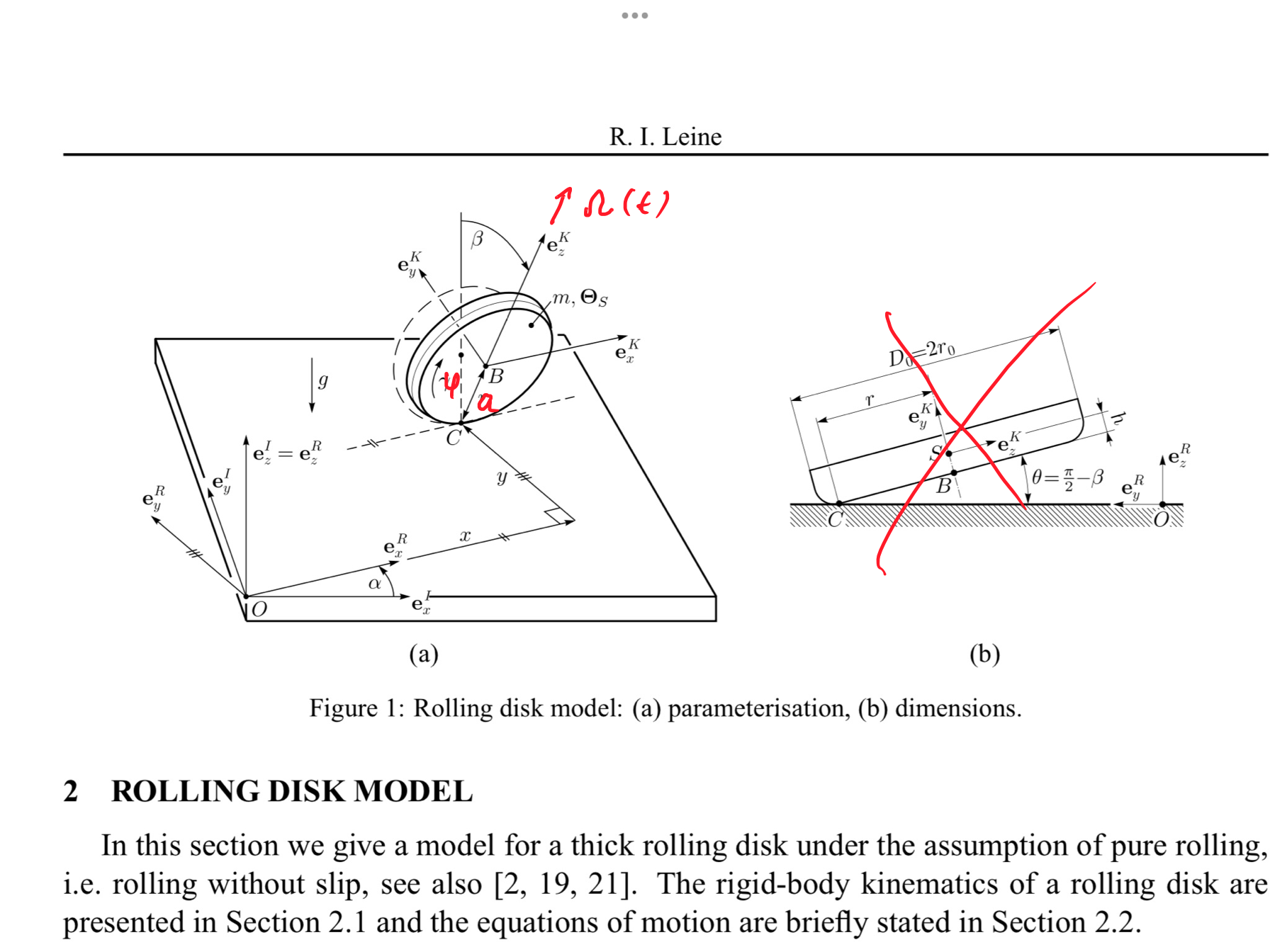
MEASUREMENTS OF THE FINITE-TIME SINGULARITY OF THE EULER DISK R. I. Leine Institute of Mechanical Systems, Department of Mechanical and Process Engineering, ETH Zurich, CH-8092 Zurich, Switzerland, [email protected]
I am not sure if this help you
the linearized equations of motion are:
\begin{align*} & \left[ \begin {array}{c} {\ddot x}\\ {\ddot y} \\ \ddot\varphi \\ \ddot\beta \end {array} \right] = \left[ \begin {array}{c} 2\,{\frac {\sin \left( \Omega\,t \right) \Omega\,\cos \left( \beta \right) \dot\beta \,a}{ \left( \cos \left( \beta \right) \right) ^{2}-6}} \\ -2\,{\frac {\cos \left( \Omega\,t \right) \Omega\,\cos \left( \beta \right) \dot\beta \,a}{ \left( \cos \left( \beta \right) \right) ^{2}-6}}\\ -2\,{\frac {\Omega \,\cos \left( \beta \right) \dot\beta }{ \left( \cos \left( \beta \right) \right) ^{2}-6}}\\ -4\,{\frac {g\sin \left( \beta \right) }{a}}\end {array} \right] \end{align*} and the roll conditions : \begin{align*} &{\dot x}+\sin \left( \Omega\,t \right) \dot\varphi \,a=0\\ &{\dot y}-\cos \left( \Omega\,t \right) \dot\varphi \,a=0 \end{align*}
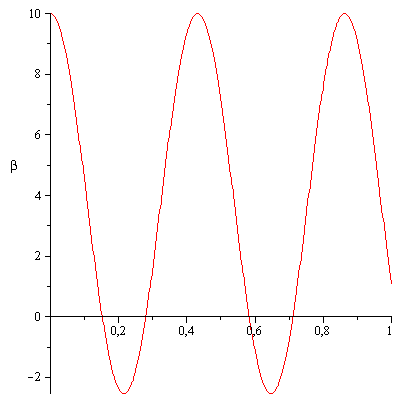
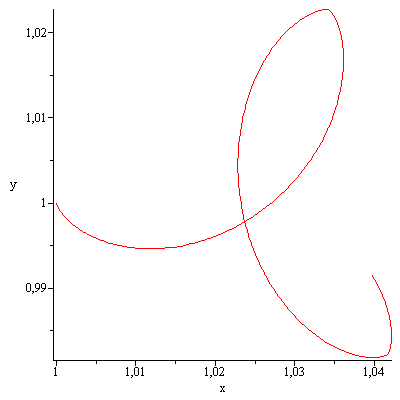
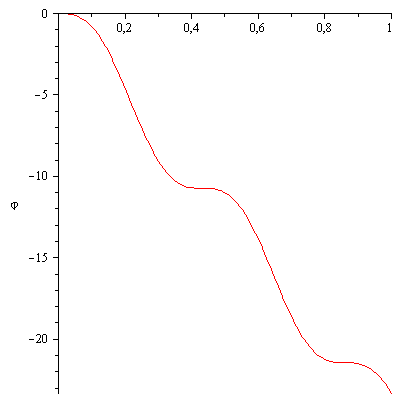
the simulation results of the non linear equations