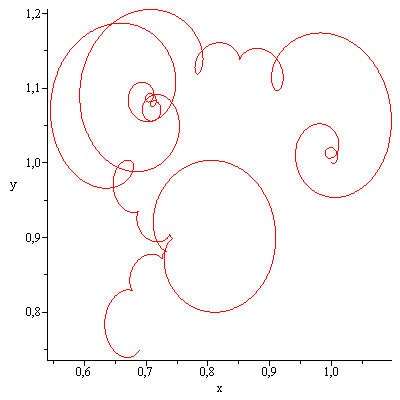
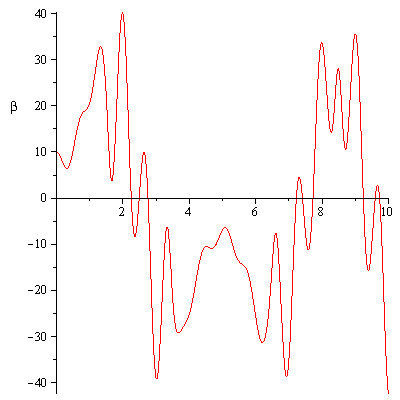
I am not sure if this help you
the linearized equations of motion are:
\begin{align*} & \ddot{x}=-\frac{1}{2}\,\,a\sin \left( \Omega\,t \right) \Omega\,\cos \left( \beta \right) \dot{\beta}\\ &\ddot y=\frac{1}{2}\,\,a\cos \left( \Omega\,t \right) \Omega\,\cos \left( \beta \right) \dot{\beta}\\ &\ddot{\varphi}=\frac{1}{2}\,\,\Omega\,\cos \left( \beta \right) \dot{\beta}\\ &\ddot{\beta}=\frac{1}{2}\,\,{\frac {-4\,g\sin \left( \beta \right) +{\Omega}^{2}\cos \left( \beta \right) a\sin \left( \beta \right) }{a}} \end{align*}
with the rolling conditions
\begin{align*} &{\dot x}+\sin \left( \Omega\,t \right) \dot\varphi \,a=0\\ &{\dot y}-\cos \left( \Omega\,t \right) \dot\varphi \,a=0 \end{align*}
the simulation results of the non linear equations