Is it possible for a point-like system to behave like $x(t) = \frac{t}{2}\log(t^2)$ near $t=0$? (infinite speed)

I know beforehand that relativity theory forbids anything with mass from travel faster than speedlight, so please stay with reading the full question first before answer - is more a conceptual/mathematical issue.

For generality issues, I will work with dimensionless units (is explained later).

This question is a modification of a previous one already closed and deleted (at least I tried to, but the system didn't allow it), because of a misused of the term "particle" to explain a point-like system described on time as a scalar variable, and I still don´t know the precise technical terminology, so I hope you get the idea. Apologizes in advance to everyone for any inconvenience caused.

Imagine a point-like system which position in time is described by the equation: $$x(t)=\frac{t}{2}\log(t^2)$$ and the point starts moving at time $t_0=-0.2$ with initial speed and the experiments ends measuring its position at time $t_f=0.2$ following the equation $x(t)$.

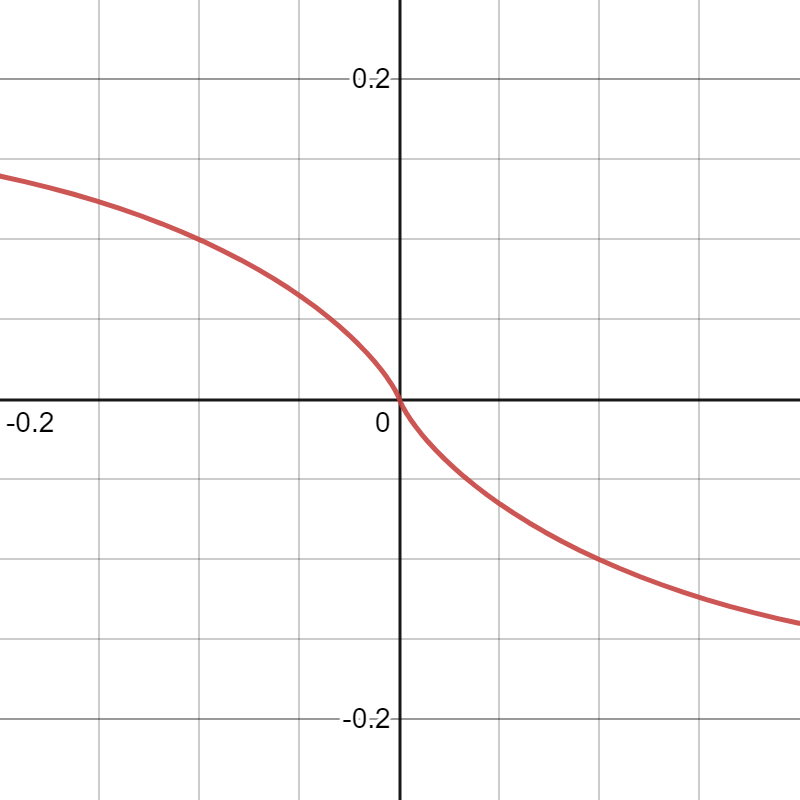
From the plot it look like something quite feasible to be done, but if I focus on their derivative I will note that there is a point in space where it maximum speed will rise to infinity without any discontinuity on the position of the point-like element: $$\frac{d}{dt}x(t) = 1+\frac{\log(t^2)}{2}$$

Since the maximum speed diverges $\lim\limits_{t\to 0}\|\dot{x}(t)\|_\infty \to \infty$, near zero is a neighborhood where the variable is "softly/continuously" (but not smoothly) achieving speeds that could be higher than the speed of light, but it never "teleport" since the function is continuous and is only singular in a zero-measure point of time (even, $\int\limits_{-0.2}^{0.2} |\dot{x}|^2\,dt < \infty$ and $\int\limits_{-0.2}^{0.2} |\dot{x}|\,dt < \infty$).

So, I am looking for examples of alike systems, if there are any, that could behave likewise $x(t)$ (on the Motivation I extend on which kind of systems I believe could be candidates).

1. Is this kind of system attainable under current interpretation of physics laws?
2. Where I am messing up with the concept of speed and its divergence?
3. If this is an achievable system, Do you know a mechanic system that behaves like this?
4. If no mechanical system is possible, Any other kind of physical model where some variable behaves as reaching infinite speed continuously in finite time?

Motivation

I would like to made an explanation of why this question is interesting in my opinion:

In this video I found by accident on youtube, Terence Tao, one of the most important nowadays mathematician, is reviewing finite-time blow up solutions to differential equations. There, he talk about the possibility of having this kind of solutions which reaches infinity in finite time for the Navier-Stokes equation, which is indeed a widely known physical system.

Thinking in the speed limits rise by Relativity Theory due to Einstein, I was wondering if this talk is just a mathematical curiosity which cannot be happening in real life, but then I saw this video about the Euler´s disk toy, where, for the wobbling rate the solutions indeed shows to have a finite time blow up for a inertial system (it just a frequency ratio so inertia restrictions indeed are not applying).

On this question, also I figure out that sometimes a finite time blow up system could be thought as the reciprocal of a solution of finite duration to a differential equation (meaning here, it achieves zero by it own dynamics and stays there forever after - some examples of finite duration solutions here, here, and and here).

So if some people are researching this kind of things, I was thinking that maybe something that achieving the blow up behavior in their speed profile could also be possible (at least is possible in the math).... this is why I keep unitless/dimensionless the differential equations, since maybe a model where inertia is not involved could fit the presented situation.

Another examples, the point-like system could be the displacement of a shadow, or the geometric point where scissors close as the example shown on this video. With this, inertia is not needed to be involved in the point-like system, but as the example of the Euler's Disk toy, the system could indeed be a realizable experiment with a variable showing a finite time blow up behavior (I don´t know if, maybe, by integration of this wobbling rate I could found a variable which do the trick).