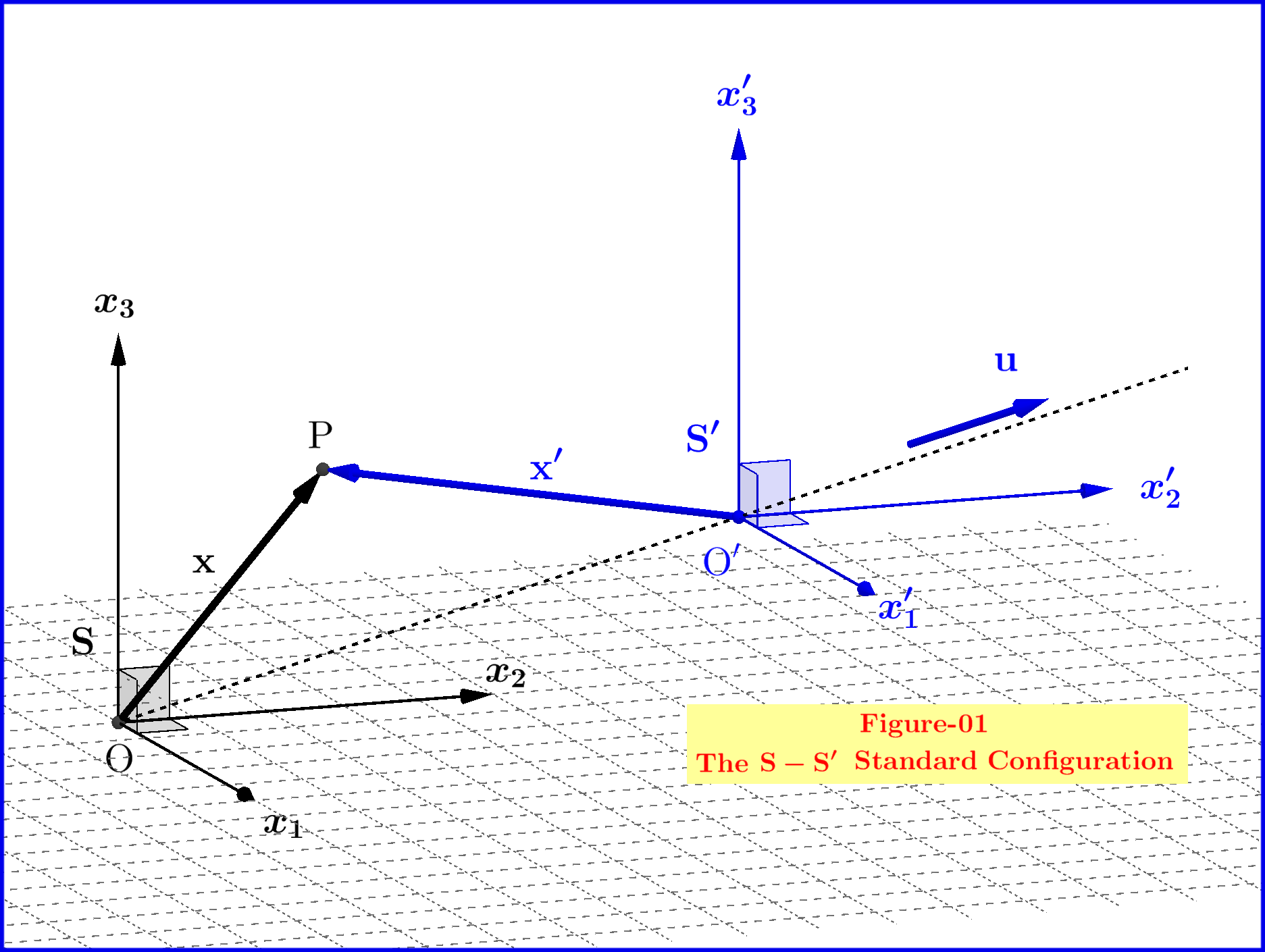
The 3+1-Lorentz transformation is \begin{align} \mathbf{x}^{\boldsymbol{\prime}} & \boldsymbol{=} \mathbf{x}\boldsymbol{+} \dfrac{\gamma^2_{\mathrm u}}{c^2 \left(\gamma_{\mathrm u}\boldsymbol{+}1\right)}\left(\mathbf{u}\boldsymbol{\cdot} \mathbf{x}\right)\mathbf{u}\boldsymbol{-}\dfrac{\gamma_{\mathrm u}\mathbf{u}}{c}c\,t \tag{01a}\label{01a}\\ c\,t^{\boldsymbol{\prime}} & \boldsymbol{=} \gamma_{\mathrm u}\left(c\,t\boldsymbol{-} \dfrac{\mathbf{u}\boldsymbol{\cdot} \mathbf{x}}{c}\right) \tag{01b}\label{01b}\\ \gamma_{\mathrm u} & \boldsymbol{=} \left(1\boldsymbol{-}\dfrac{\mathrm u^2}{c^2}\right)^{\boldsymbol{-}\frac12} \tag{01c}\label{01c} \end{align} and in differential form \begin{align} \mathrm d\mathbf{x}^{\boldsymbol{\prime}} & \boldsymbol{=} \mathrm d\mathbf{x}\boldsymbol{+}\dfrac{\gamma^2_{\mathrm u}}{c^2 \left(\gamma_{\mathrm u}\boldsymbol{+}1\right)} \left(\mathbf{u}\boldsymbol{\cdot} \mathrm d\mathbf{x}\right)\mathbf{u}\boldsymbol{-}\dfrac{\gamma_{\mathrm u}\mathbf{u}}{c}c\,\mathrm dt \tag{02a}\label{02a}\\ c\, \mathrm dt^{\boldsymbol{\prime}} & \boldsymbol{=} \gamma_{\mathrm u}\left(c\,\mathrm dt\boldsymbol{-} \dfrac{\mathbf{u}\boldsymbol{\cdot} \mathrm d\mathbf{x}}{c}\right) \tag{02b}\label{02b} \end{align}
Could you check in \eqref{02a} what would be the quantity $\mathrm d\mathbf{x}^{\boldsymbol{\prime}}$ if in this same equation you replace \begin{equation} \mathbf{u}\boldsymbol{\longrightarrow}\dfrac{\mathrm d\mathbf{x}}{\mathrm dt} \tag{03}\label{03} \end{equation} under the assumption \begin{equation} \left\Vert\dfrac{\mathrm d\mathbf{x}}{\mathrm dt}\right\Vert <c \tag{04}\label{04} \end{equation}