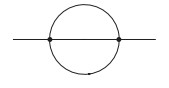
Edit: This is in $\phi^4$ theory.

Given this Feynman diagram

And using this formula to calculate the symmetry factor

$S = v\prod_{k}(k!)^{\pi _{k}}$

I calculate: v = 1, as you can only change the vertices labels once and retain the diagrams topology.

$\pi _{0} = 0$ as there are 0 pairs of vertices connected by 0 identical propagators.

$\pi _{1} = 0$ similarly

$\pi _{2} = 1$, as the pair are connected by 2 identical propagators.

$\pi _{3}$ = $\pi _{4} = 0$ as above.

This gives an overall symmetry factor of 2.

However, in the solution, $\pi _{2} = 0$, and $\pi _{3} = 1$, giving an overall symmetry factor of 6.

From my understanding of $\pi _{k}$, I don't see how this can be, as there aren't 3 identical propagators connecting this pair. What makes them identical?