The probability amplitude for single particle to enter our system with momentum $k$ and leaving with momentum $q$ can be calculated as \begin{align} A(k\to q ) = \langle q| T e^{i \int_{-\infty}^\infty dt \; H_i} |k \rangle \, \end{align} where $|k \rangle \equiv \sqrt{2\omega_k} a^\dagger(k) |0\rangle$ and $\langle q | \equiv \langle 0 | a(k) \sqrt{2\omega_q} $. If we use the usual series expansion of the exponential function $e^x = 1+x+x^2/2 +\ldots$, we can consider the probability amplitude term by term $$ A(k\to q ) = A^{(0)} + A^{(1)}+ \ldots$$ The first term reads \begin{align} A^{(0)} = \langle q| 1 |k \rangle = (2\pi)^3 \delta( k - q) \, . \end{align} This is exactly what we would expect. The result is only nonzero for $k=q$ since there is only one particle in the system and thus no momentum transfer can happen. But then, what do all additional terms describe? Do they vanish? The probability that we end up with a particle of momentum $q$ if we start with a particle of momentum $k$ should be $100\%$ if $q=k$ and zero otherwise. This is already described perfectly by $A^{(0)}$ and thus it's not clear to me what the remaining terms contribute. Do they really alter the first order result?

For concreteness, let's consider $\phi^4$ theory, $H_i = \frac{-\lambda}{4!} \phi^4 $. The next term in the series expansion reads \begin{align} A^{(1)} = -i \frac{\lambda}{4!} \langle q| T \phi^4 |k \rangle \, . \end{align} It can be evaluated, for example by using Wick's theorem. The calculation is done on page 178-180 in the book Quantum Field Theory for the Gifted Amateur by Blundell and Lancaster. They find, for example, that there are 12 terms which yield a contribution of the form (Eq. 19.16 in the book) $$ -i \frac{\lambda}{2} \int d^4 z \frac{d^4k}{(2\pi)^4} e^{iz(q-p)} \frac{1}{k^2-m^2+ i \epsilon} .$$ Then they suddenly become quite and stop with the words "we could, at this point, collect the terms together and prepare to integrate the position of the interactions over all spacetime." This seems quite odd, but let's carry out the integration nevertheless. The $d^4z$ integration yields a delta $$ -i \frac{\lambda}{2} \delta(q-p) \int \frac{d^4k}{(2\pi)^4} \frac{1}{k^2-m^2+ i \epsilon} ,$$ which, as before, describe the conservation of momentum. But what about the remaining $d^4k$ integral? I know that diagrammatically the term here represents a self-loop diagram. 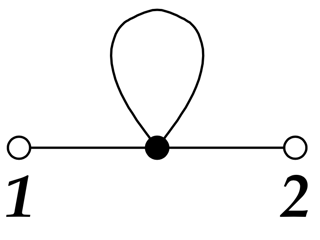The argument given above seems to suggest that it vanishes. But then I would assume that Blundell and Lancaster would mention this and we wouldn't talk about self-loop diagrams at all. Thus there must be something wrong in my arguments.

I'm aware of the fact that tadpole diagrams can be removed by normal ordering the interaction Hamiltonian from the start. Thus they really have no impact on observables. However, I'm trying to understand this without such an adhoc argument. In other words, how can we understand the contributions of tadpole diagrams without simply throwing them into the bin from the start?