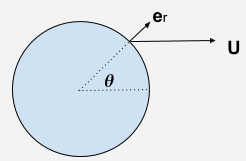
Since the flow is irrotational, velocity vector may be written as $\mathbf{u}=\nabla\phi$, where $\phi$ is a scalar potential. The cylinder moves with constant velocity $\mathbf{U}$ (vectors are denoted by bold-face font). Which means that at the cylinder's surface $\mathbf{u}=\nabla\phi=\mathbf{U}$.
Consider a cylindrical coordinate system whose origin is instantaneously coincident with the center of the cylinder. Now the normal to the surface of the cylinder is the unit radial vector $\mathbf{e}_r$. Forming the scalar product of the previous expression with $\mathbf{e}_r$ we get: $$\mathbf{e}_r\cdot\nabla\phi=\mathbf{e}_r\cdot\mathbf{U}\\\Rightarrow\quad\frac{\partial\phi}{\partial r}=U\cos\theta$$ in which $U$ is the magnitude of cylinder's velocity.