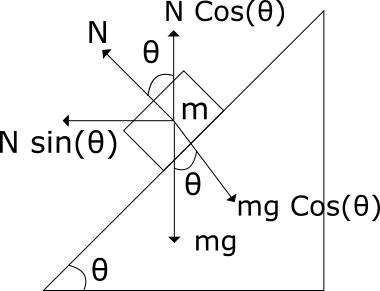
Suppose there is a banked road on which a body is placed as shown in the figure.
Now to derive the relation between the velocity and the angle of inclination of the slope we do the following:-
Taking horizontal component of normal reaction and equating it to centripetal force.
$$N \sin(\theta) = f_c = {mv^2\over r}\qquad (1)$$
equating normal reaction to component of weight, co-linear to normal reaction.
$$N = mg \cos(\theta)\qquad (2)$$
Substituting $(2)$ in $(1)$
$$mg\cos(\theta)\sin(\theta) = {mv^2\over r}$$ $$\sin(2\theta) = {2v^2\over gr}$$ $$\theta = \large{\arcsin\left({2v^2\over gr}\right)\over 2}$$
But in solution set, they took $N \cos(\theta) = mg \qquad (3)$
Dividing $(1)$ by $(3)$
$$\tan (\theta) = {v^2\over gr}$$ $$\theta = \arctan\left({v^2\over gr}\right)$$
From $(3)$, $N =\large{mg \over \cos(\theta)}$, whereas from $(2)$ , $mg\cos(\theta) = N$. Now both of these can't be true. So why is $(2)$ false and $(3)$ true ?
I have found many other similar questions in this site but none of the answers were quite satisfying.
Please don't flag the question as duplicate because I have been struggling a long to find the answer.
Any help is highly appreciated.