I am trying to independently solve physics problems and I am attempting to solve Chapter 5 problem 4-4 on page 140 from the book "Feynman's Tips on Physics".

The problem is as follows:

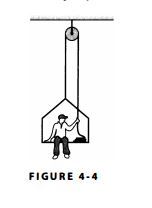
A painter weighing 180 lbs working from a "bosun's" chair hung down the side of a tall building desires to move in a hurry. He pulls down on a fall rope with such a force that he presses against the chair with only a force of 100 lb. The chair itself weighs 30 lb.

(a) What is the acceleration of the painter and the chair?

(b) What is the total force supported by the pulley?

The diagram is as follows:

MY WORK:

(a) The question states the weights of the painter and the chair are 180 lbs and 30 lbs respectively. I used this idea to perform the following step:

$$\text {Total mass of the painter and the chair} = \left(\frac{(180 + 30) \rm lbs}{g}\right) $$

He exerts a downward force of 100 lb on the chair. His net motion will be upwards.

So the equation I formed is:

$\text{Total force from the upwards motion = Weight of man + Weight of chair + Force on the chair}$

$\frac{180 + 30}{g}\times a = $180 lb + 30 lb + 100 lb

This is giving me:

a = $\frac{31 g}{21} \frac {m}{s^2}$

What am I missing in my analysis of the question?

(b)

The net downwards force is $\text{Weight of man + Weight of chair + Force exerted on the chair}$ which is $\text{310 lbs}$. I am compelled to think that an equal and opposite upwards force of 310 lbs will be exerted on the pulley.

The tension in the pulley string came to my mind but it gets cancelled out. The 100 lbs downwards force on one side will exert an equal and opposite upwards force on the other side. Consequently, the resultant force on the string will be zero.

Are there any factors I am not considering in my analysis of (b)?