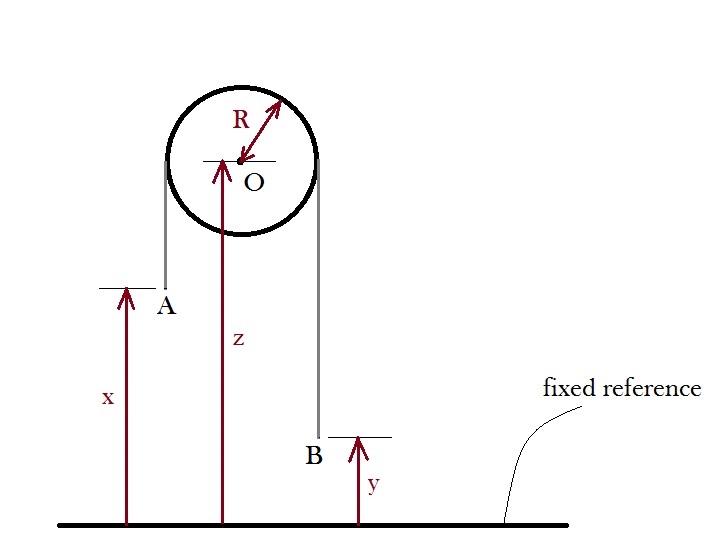
I think there is a typographical mistake in your question. I guess you want a proof for $\dot z=\large{\frac{\dot x+\dot y}2}$ or $v_O=\large{\frac{v_A+v_B}2}$.
If the length of the string is fixed (i.e. if the string is inextensible), then we have ($l$ is the length of the string): $$l=(z-x)+(z-y)+2R=2z-(x+y)+2R$$ $$\Longrightarrow\; 2z=x+y+\textrm{constant}$$ $$\Longrightarrow\; \frac d{dt}(2z)=\frac d{dt}\left(x+y+\textrm{constant}\right)$$ $$\Longrightarrow\; 2\dot z=\dot x+\dot y\quad \text{or}\quad 2v_O=v_A+v_B$$