As you correctly note, the solution is different when the applied forces are not equal. The bar is not in static equilibrium: Both static and dynamic forces deform the bar in motion. These concepts are illustrated by superposition.
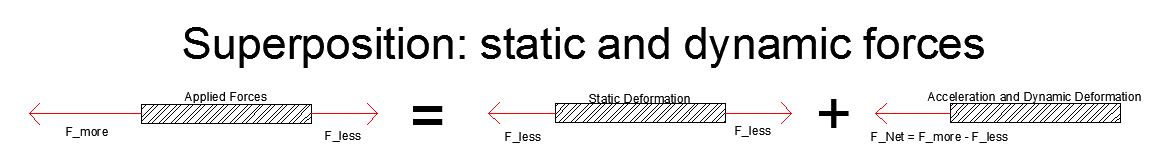$$\delta = \delta_{static} + \delta_{dynamic} \qquad = \frac {F_\text{less}L}{EA} + \frac{(F_{more} - F_{less})L}{2AE}$$
During changes in acceleration (when $\frac{\mathrm{d\;a(t)}}{\mathrm{dt}} \neq 0$), forces and accelerations within the damped solid body are transient, where $a(x,t)$, until they reach steady-state, where $\frac{\partial{\;a(x,t)}}{\partial{x}} = 0$.
Transient deformations in solid bodies are illustrated by a mass/spring system, where each mass element can be thought to represent differential mass element.
Newton's Second Law requires that the bar (of mass $M$) accelerate in the direction of $F_{net}$. $$\sum F \;\text{on bar:} \qquad F_\text{more}-F_\text{less} = Ma \qquad \Rightarrow \;\therefore a = \frac{F_{more} - F_{less}}{M}$$
The derivation of deformation is shown when a single force acts on the bar.
Dynamic Deformation:
A Free Body Diagram is taken at an arbitrary cross-section of the bar, where the mass of the split body is $m = (\frac{M}{L})x$. Summation of forces acting on $m$ is solved for $T(x)$.
$$\sum F \;\text{on split body:} \qquad F_{o} - T = ma$$ $$F_{o} - T = \overbrace{(\frac{M}{L}x)}^\text{m} \overbrace{(\frac{F_{o}}{M})}^\text{a} = \frac{F_{o}}{L}x \qquad \Rightarrow \qquad T = F_{o} - \frac{F_{o}x}{L}$$ $$\therefore T = F_{o}(1-\frac{x}{L})$$ The static axial deformation ($\delta = \frac{FL}{AE}$) written in differential form:
$$\mathrm{d \delta} = \frac{T \mathrm{dx}}{AE} = \frac{[F_{o}(1-\frac{x}{L})]\mathrm{dx}}{AE}$$ Integrate differential deformation over the length of the bar to determine total deformation: $$\delta = \int_0^L \mathrm{d \delta} \qquad = \frac{F_{o}}{AE} \int_0^L 1-\frac{x}{L} \mathrm{dx} \qquad \Rightarrow \; \therefore \delta = \frac{F_{o}L}{2AE}$$
The derivation can be generalized to include both forces, where $T(x) = F_{more} - \frac{F_{more}-F_{less}}{L}x$, the same solution given by superposition.
References: