Basically the whole kinetic energy is transfered to pressure, and then this pressure will be transfered to kinetic energy again; this time only the direction is as defined by hydrostatic pressure; perpendicular to surface.
This above gives a following basis;
Kinetic energy of the ball is also it's potential energy (no friction on fall) Ekin = m g H This is then transferred to pressure through the ball surface; A = 4 pi r^2
This pressure then splashes the fluid up;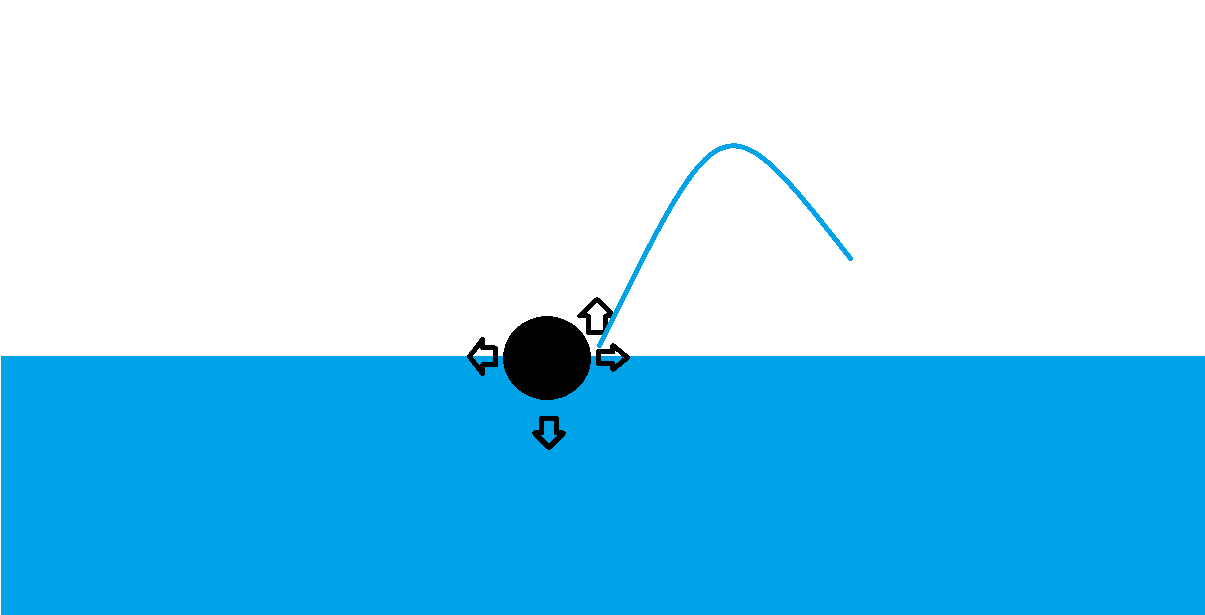 
In optimal case the diameter of the ball is almost zero, and viscosity of the fluid is such, that the ball would stop in a distance of slightly more than r.
This would lead to a situation where the vertical velocity of the water is very low, and thus the water would jump almost directly upwards. This doesn't actually matter too much, if the air friction is not considered.
Ok, so an answer, if the density of the ball is same as the density of the fluid. Then the fluid would jump to same height as the ball was dropped, if we also consider that there is no viscous losses. This is never true, and thus the ball drops deeper in the fluid and the losses reduces the available energy. This all could be calculated. But the interesting thing is that there is a hole in the water when it goes deeper;
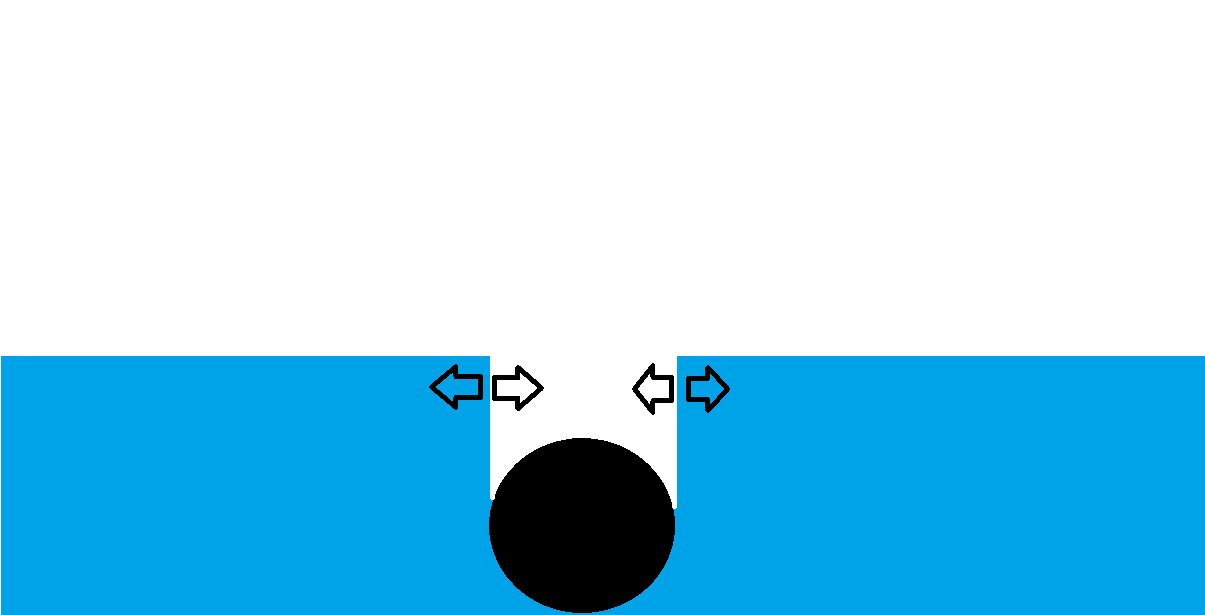
And this means that the fluid which has maximum pressure has now a surface with no pressure. And therefore the fluid goes with even higher velocity back to fill this hole; as the velocity come from pressure difference, what happens;
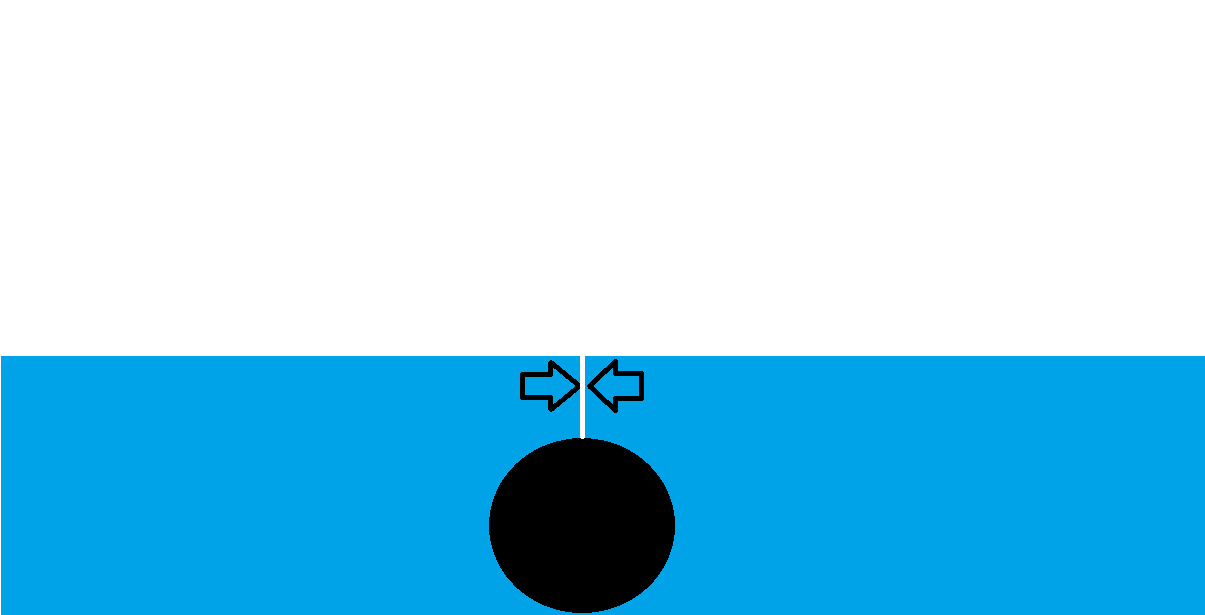It collides in the middle of the hole, but this time there is many velocities reaching the same point at the same time. Again all these velocities are transferred to pressure and the fluid takes new direction.
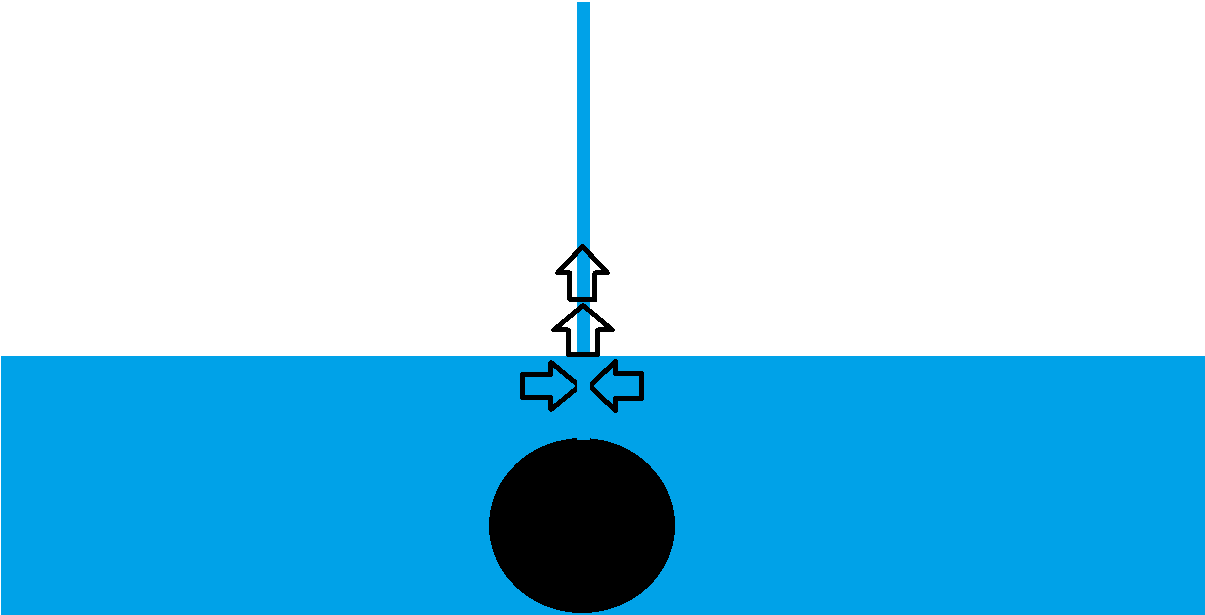In 2-dimensional world this new velocity component would be 2-times the original. In 3-D reality it's more, and in true reality it's limited by viscous losses, surface tensions, etc. etc.
So to conclude this all; The splash hight can be anything. The round splash could theoretically reach the Height H, but the Middle-splash can be even higher.
At this video found from comments, there is a golf-ball used to do the splash. And such a Golf ball makes a higher middle-splash than a round ball, because the boundary layer of the ball makes less losses, but also disturbs the fluid less. And therefore the returning middle splash is so big in this video; the collision happens with minimal disturbances; and the velocity vectors really hits against each others.