3
$\begingroup$

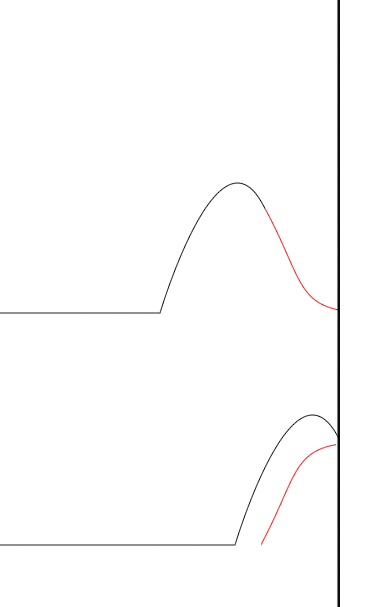
When we have a rope with one fixed end and we send a pulse through it, the reflected pulse is inverted. My question is as follows - is it correct to say that near the end (when the pulse hits the fixed end) the reflected-inverted pulse is superposing with the part of the pulse which didn't hit the end yet? In other words, is it right that the pulse pattern which we can observe when the incident pulse hits the fixed end is simply overlapping of two waves - the part which is incident and the part which is reflected?

For example:

enter image description here

Improve this question
$\endgroup$
3
  • 1
    $\begingroup$ I've always taken this to be the case. For a while I wondered how the wave continued to propagate when it fully destructively interferes with itself but I think the answer is that the wave energy is converted into tension (or other stress) in the rope temporary. $\endgroup$
    – Brandon Enright
    Commented Jan 31, 2014 at 21:30
  • 1
    $\begingroup$ @BrandonEnright - thank you for your comment, sir. This was confusing for me as well. $\endgroup$
    – user38671
    Commented Jan 31, 2014 at 22:05
  • $\begingroup$ When it's "fully destructively interfering" as you describe, different parts of the rope are moving in different directions. (I doubt there's any extra tension since the rope is completely at its equilibrium position, but I could be wrong.) The conditions $y(x)=0$ and the particular form of $\dot{y}(x)$ are enough to "recover" the reflected and inverted wave. $\endgroup$
    – BMS
    Commented Jan 31, 2014 at 22:13

2 Answers 2

Reset to default
1
$\begingroup$

If you are asking whether the standing wave pattern you get is the effect of the superposition of two waves, then the answers is yes. Just take a look at the Wikipedia page of standing waves

Improve this answer
$\endgroup$
2
  • $\begingroup$ I understand the principle behind standing waves. I just felt that it is sort of stable pattern which is created after some long period of time. I thought that the boundary process I'm asking about in this particular question maybe somewhat more complicated. For example, maybe the pulse is reflected only as a whole. $\endgroup$
    – user38671
    Commented Jan 31, 2014 at 22:02
  • $\begingroup$ Mmh, ok. I don't think that it would take that long a time to get this stable effect but the boundary proces is indeed interesting. $\endgroup$
    – candela137
    Commented Jan 31, 2014 at 22:40
0
$\begingroup$

Yes. It is a result of linear differential equations, whereas two or more special solutions to the equation can be superimposed to form a general solution. In your case the wave traveling to the left is one special solution and another wave traveling to the right is a second special solution.

Together they make the general solution of a wave on a wire

$$ y(x,t) = A\,{\rm S}\left( \omega\, ( t - \frac{x}{c} ) \right)+B\,{\rm S}\left( \omega\, ( t + \frac{x}{c} ) \right) $$

where ${\rm S}()$ is the shape function, $c$ is the wave speed and $\omega$ is the frequency in radians per second. The coefficients $A$ and $B$ are the amplitudes of the two waves. When a fixed node exists then $A=-B$.

Improve this answer
$\endgroup$
1
  • $\begingroup$ Thank you very much, sir. So basically, my illustration is correct? $\endgroup$
    – user38671
    Commented Jan 31, 2014 at 22:15

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.