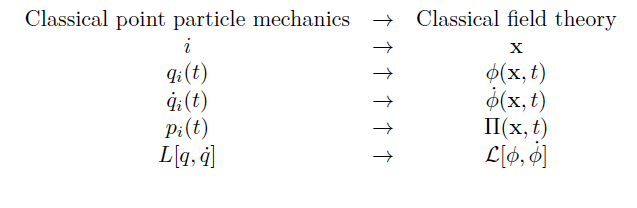
Although I am not 100% sure what you are asking, I believe you are talking about considering a lattice of discrete points and taking the continuous limit such that the distance between the lattice points tend to zero.
The simplest example is to consider an 1-dimensional elastic rod with mass density $\mu$. The force applied on it due to Young's modulus is given by:
\begin{equation}
F = -Y \xi
\end{equation}
where $\xi$ denotes the deflection of the elastic rod from its equilibrium position. We can think of the rod as an infinite amount of equally spaced spaced particles at rest where we will let $m$ denote the mass of each particle (which is of course the same for each particle) and we let $a$ denote the distance between the particles. Now, we can write the mass density as follows:
\begin{equation}
\mu = \frac{dm}{dx} = \displaystyle\lim_{a\to 0} \frac{m}{a}
\end{equation}
Now we will assume that each particle only interacts with its nearest neighbours and so the force between the particles can be approximated by using Hooke's law:
\begin{equation}
F = - \kappa \left(y_{i+1}-y_i\right) = - \left( \kappa a \right) \frac{y_{i+1}-y_i}{a}
\end{equation}
Furthermore, by writing the force expressed in terms of Young's modulus in terms of the relative distance $a$:
\begin{equation}
F = - Y \frac{y_{i+1}-y_i}{a}
\end{equation}
we can write:
\begin{equation}
Y= \displaystyle\lim_{a\to 0} \left(\kappa a \right)
\end{equation}
To sum up, we have related Hooke's constant to Young's modulus.
Furthermore, by ordinary classical mechanics we can write the potential energies in all the springs as:
\begin{equation}
V= \sum\limits_{i} \frac{1}{2} \kappa \Delta y_i^2 = \sum\limits_{i} \frac{1}{2} \kappa \left(y_{i+1}-y_i\right)^2
\end{equation}
and the kinetic energy of all particles is given by:
\begin{equation}
T= \sum\limits_{i} \frac{1}{2} m \dot{y}_i^2
\end{equation}
Therefore, the Lagrangian of the system is given by:
\begin{equation}
L=T-V = \sum\limits_{i}\left[ \frac{1}{2} m \dot{y}_i^2 - \frac{1}{2} \kappa \left(y_{i+1}-y_i\right)^2 \right]
\end{equation}
Using the Euler-Lagrange equation, we can easily find the equations of motion for each particle $j$ in the discretized rod:
\begin{equation}
\frac{\partial L}{\partial y_k} - \frac{d}{dt} \left( \frac{\partial L}{\partial \dot{y}_k} \right) = 0
\end{equation}
\begin{equation}
\Rightarrow \frac{\partial }{\partial y_k} \left( \sum\limits_{i} \frac{1}{2} \kappa \left(y_{i+1}-y_i\right)^2 \right) + \frac{d}{dt} \left[ \frac{\partial }{\partial \dot{y}_k} \left( \sum\limits_{i} \frac{1}{2} m \dot{y}_i^2 \right) \right] = 0
\end{equation}
\begin{equation}
\Rightarrow \frac{\partial}{\partial y_k} \left( \frac{1}{2} \kappa \left(y_{k+1}-y_k\right)^2 + \frac{1}{2} \kappa \left(y_{k}-y_{k-1}\right)^2 \right) + \frac{d}{dt} \left[ \frac{\partial }{\partial \dot{y}_k} \left( \frac{1}{2} m \dot{y}_k^2 \right) \right] = 0
\end{equation}
\begin{equation}
\Rightarrow - \kappa \left(y_{k+1}-y_k\right) + \kappa \left(y_{k}-y_{k-1}\right) + m \ddot{y}_k = 0
\end{equation}
Let us now consider the limit that the spacing in the discretized rod tends to zero:
\begin{align}
y_k(t) & \rightarrow y(x,t) \\
y_{k+1}(t) & \rightarrow y(x+a,t) \\
y_{k-1}(t) & \rightarrow y(x-a,t) \\
y_{k+2}(t) & \rightarrow y(x+2a,t) \\
y_{k-2}(t) & \rightarrow y(x-2a,t) \\
& \dots
\end{align}
The equations of motion can now be written as:
\begin{equation}
- \kappa \left[ y(x+a,t) - y(x,t) - y(x,t) + y(x-a,t) \right] + m \ddot{y}(x,t) = 0
\end{equation}
\begin{equation}
\Rightarrow - \kappa \left[ \frac{y(x+a,t) - y(x,t)}{a} - \frac{y(x,t) - y(x-a,t)}{a} \right] + \frac{m}{a} \ddot{y}(x,t) = 0
\end{equation}
\begin{equation}
\Rightarrow - \left( \kappa a \right) \left[ \frac{\left(y(x+a,t) - y(x,t)\right)/a}{a} - \frac{\left(y(x,t) - y(x-a,t)\right)/a}{a} \right] + \frac{m}{a} \ddot{y}(x,t) = 0
\end{equation}
Taking the limit $a \rightarrow 0$, we can write:
\begin{equation}
\displaystyle\lim_{a\to 0} \frac{y(x+a,t) - y(x,t)}{a} = \frac{\partial y(x,t)}{\partial x}
\end{equation}
and:
\begin{equation}
\displaystyle\lim_{a\to 0} \frac{y(x+a,t) - 2y(x,t) + y(x-a,t)}{a^2} = \frac{\partial^2 y(x,t)}{\partial x^2}
\end{equation}
Therefore, we obtain the equations of motion for the vibration of the field $y(x,t)$:
\begin{equation}
-Y \frac{ \partial^2 y(x,t)}{\partial x^2} + \mu \frac{ \partial^2 y(x,t)}{\partial t^2} = 0
\end{equation}
Edit: