Throwing a ball upwards in an accelerating train

Question

If I throw a ball upwards to a certain height in an accelerating train, will it end up in my hand? At the moment I release the ball, it will have a velocity equal to that of the train at that instant. But because the train is accelerating, the train will have a greater velocity and thus the ball will travel lesser distance and should fall behind me. Is my reasoning correct?

Answer 1

User Sahil Chadha has already answered the question, but here's the math and a pretty picture for anyone who is unconvinced that you're right.

Since the train is accelerating, from the perspective of an observer on the train, the ball will experience a (fictitious) force in the direction opposite the train's travel having magnitude $ma$ where $m$ is the mass of the ball and $a$ is the magnitude of the acceleration of the train. If we call the direction of travel the positive $x$-direction, and if we call the "up" direction the positive $y$-direction, then the equations of motion in the $x$- and $y$-directions will therefore be as follows: \begin{align} \ddot x &= -a \\ \ddot y &= -g. \end{align} The general solution is \begin{align} x(t) &= x_0 + v_{x,0} t - \frac{1}{2}a t^2 \\ y(t) &= y_0 + v_{y,0} t - \frac{1}{2}g t^2 \end{align} Now, let's say that the origin of our coordinate system lies at the point from which the ball is thrown so that $x_0 = y_0 = 0$ and that the ball is thrown up at time $t=0$ with velocity $v_{y,0} = v$ and $v_{x,0} = 0$ in the positive $y$-direction, then the solutions becomes \begin{align} x(t) &= -\frac{1}{2}a t^2\\ y(t) &= vt - \frac{1}{2} gt^2 \end{align} So what does this trajectory look like? By solving the first equation for $t$, and plugging this back into the equation for $y$, we obtain the following expression for the $y$ coordinate of the particle as a function of its $x$ coordinate along the trajectory: \begin{align} y(x) = v\sqrt{-\frac{2x}{a}} +\frac{g}{a} x \end{align} Here's a Mathematica plot of what this trajectory looks like for $v = 1.0\,\mathrm m/\mathrm s$ and the list $a = 9.8,5.0,2.5,1,0.1\,\mathrm m/\mathrm s^2$ of values for the train's acceleration

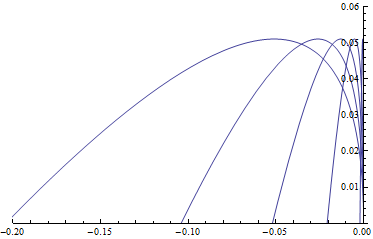
From the point of view of someone on the train, the ball flies backward in a sort of deformed parabola, but the smaller the acceleration is, the more it simply looks as it would if you were to throw a ball vertically in an un-accelerated train.

Answer 2

Yes your reasoning is correct,from the point of view of train the ball will travel in a tilted parabolic path as direction of apparent gravity will be different in the train and will not end up in your hand

Answer 3

we can also solve this using the concept of inertia. When we consider an accelerating train the velocity of the train continuously changes. The ball thrown upwards possesses the inertia of motion of the train. Hence at that moment when the train changes its velocity the ball continues to travel with the previous velocity of the train which is definitely less than the new velocity (because it is given that the train accelerates). Hence the ball falls backwards as the train now has a grater velocity than the ball.

Answer 4

when you launched the ball upwards,as at that instant when the ball is in air and it falling possibilities should be on the palm but practically,I think its not possible it will return to your palm because no driver can maintain an exact uniform speed without changes.Only machines or robots can do so.The person also launching the ball cannot be so accurate because there will always be reduction in useful energy.

Answer 5

rising and falling of ball takes time even if the train is in more or less uniform velocity suppose if upward rising of ball takes a time of 2 sec in a train any the train will move a certain distance even it is in motion resulting the ball may fall to the floor away from its original distance