Can a stable energy system be created using variable capacitors to generate high current for energy recovery?

Question

My idea is:

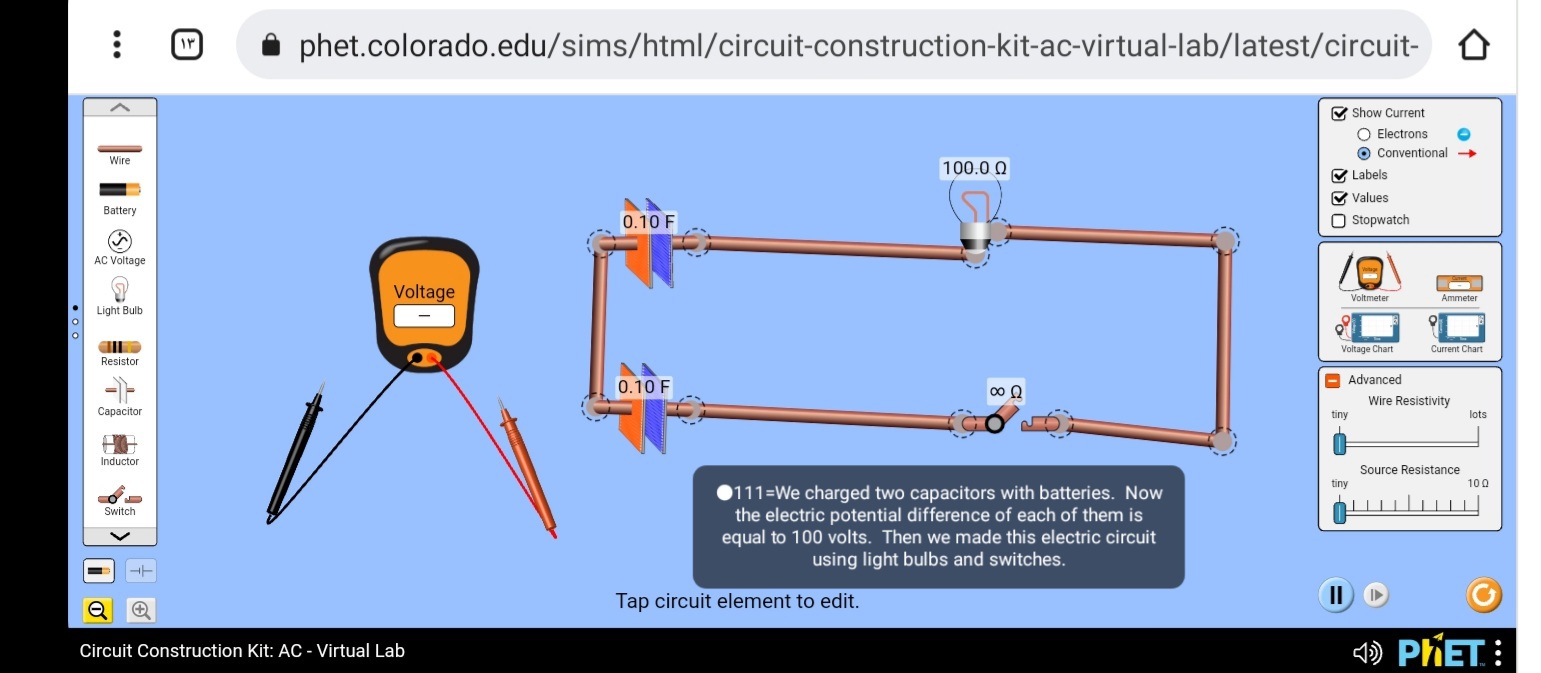
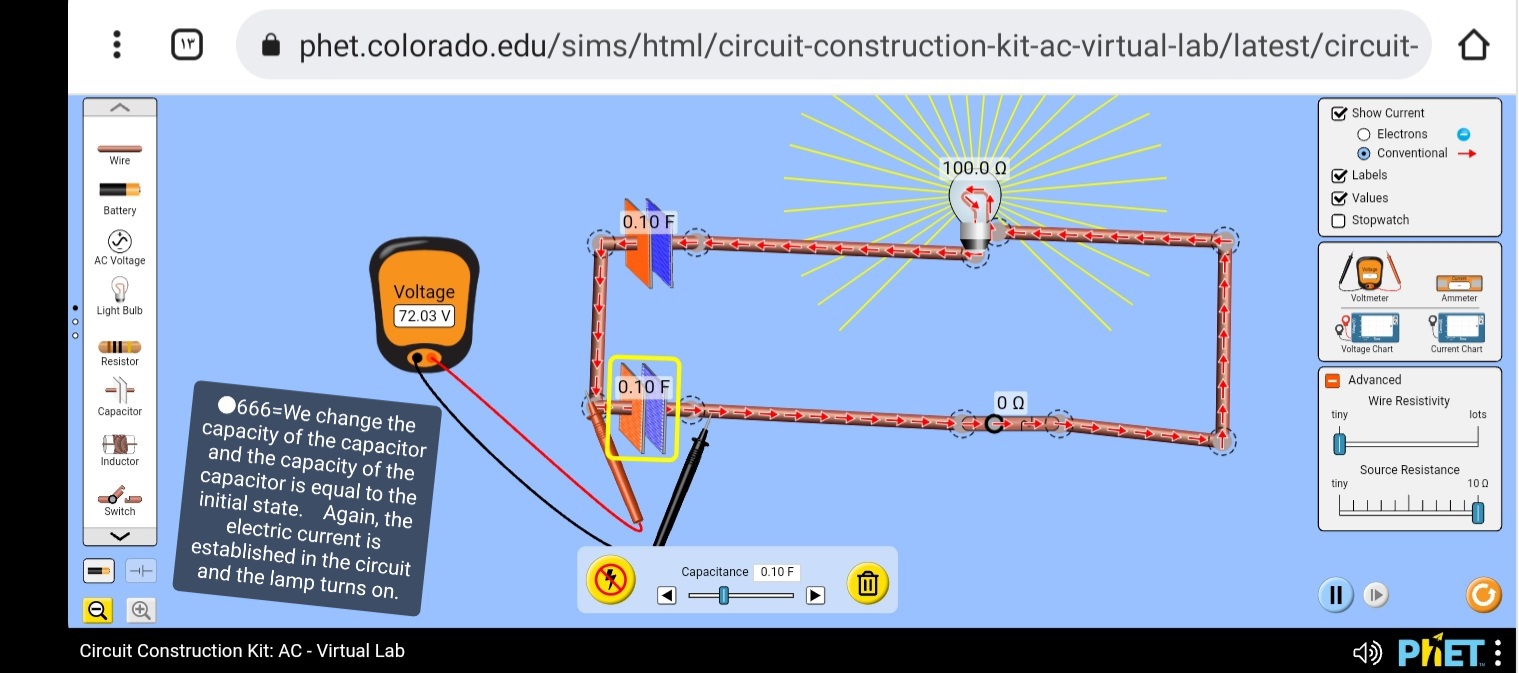
If two charged capacitors are connected in parallel so that their positive plates are connected together and their negative plates are connected together. Then, if the capacity of one of them changes, a significant current is created in the circuit. If we put a consumer (such as a lamp) in the path of this current, this energy can be used to turn on the lamp.

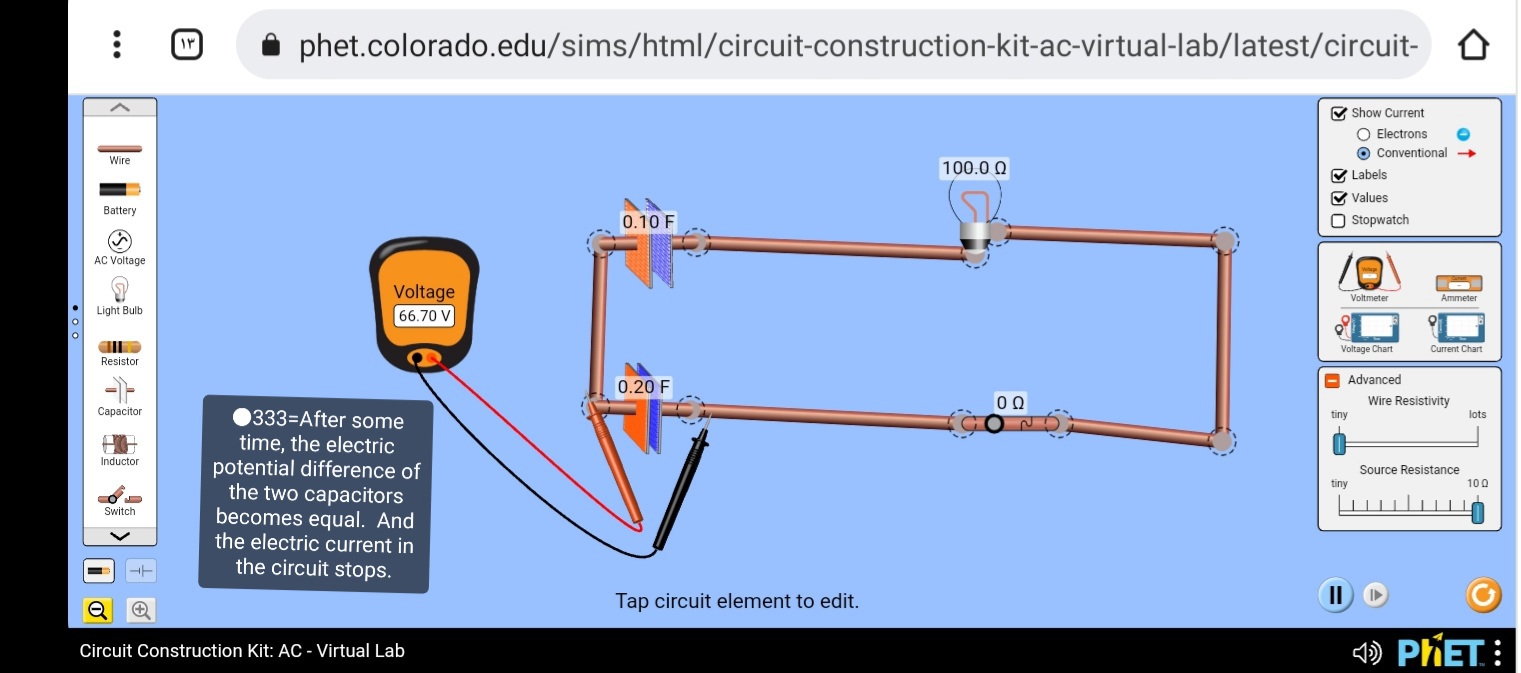
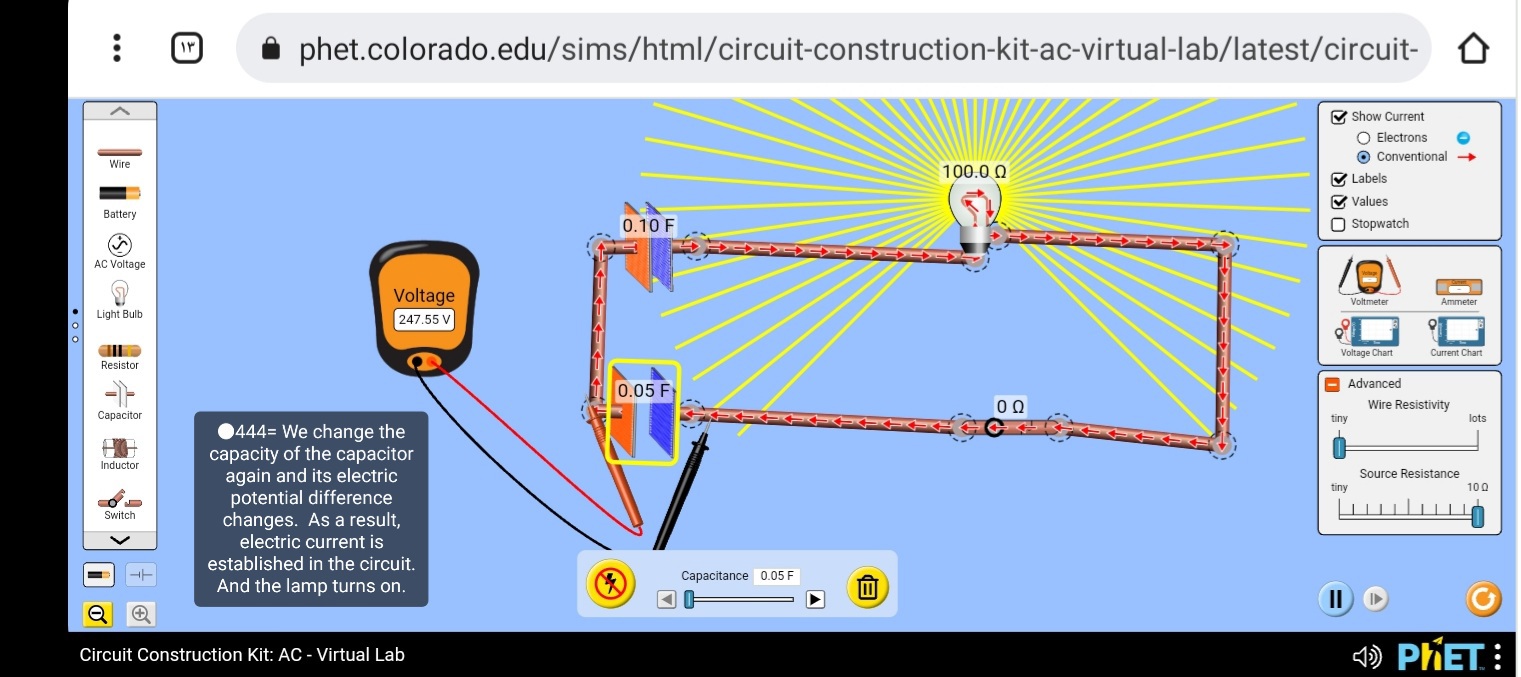
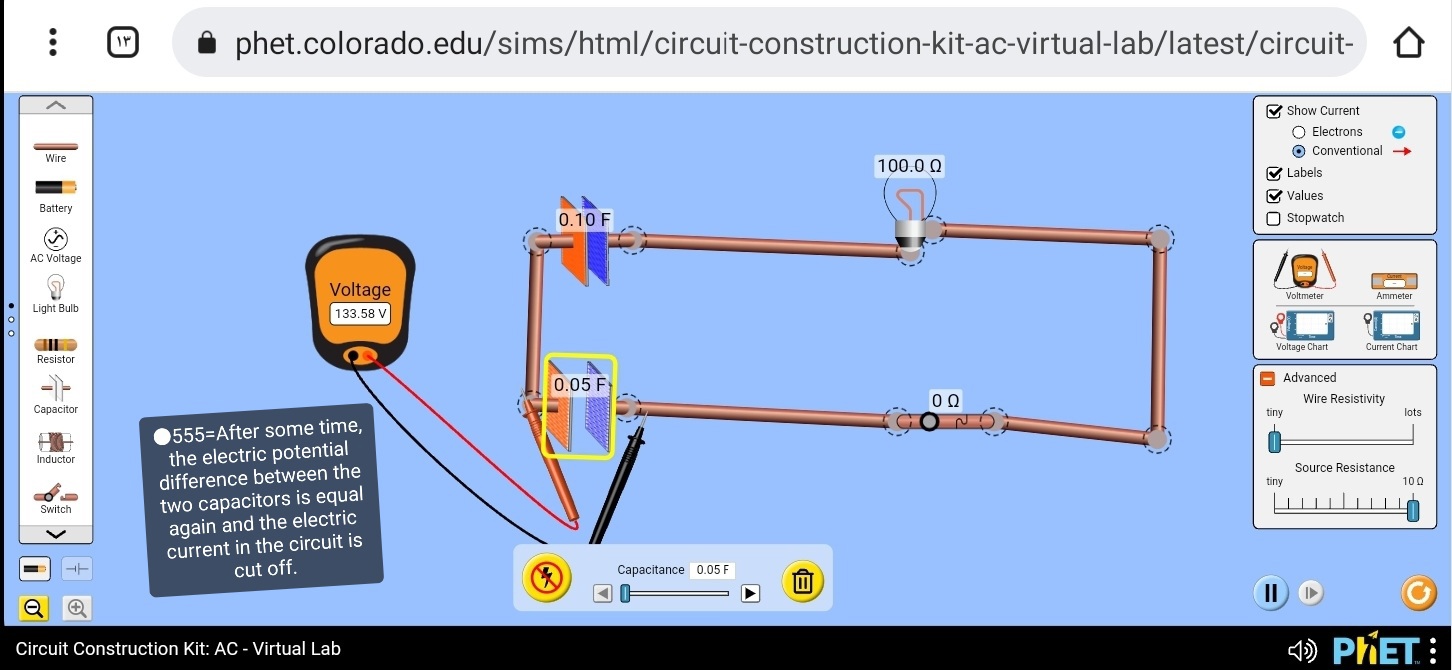
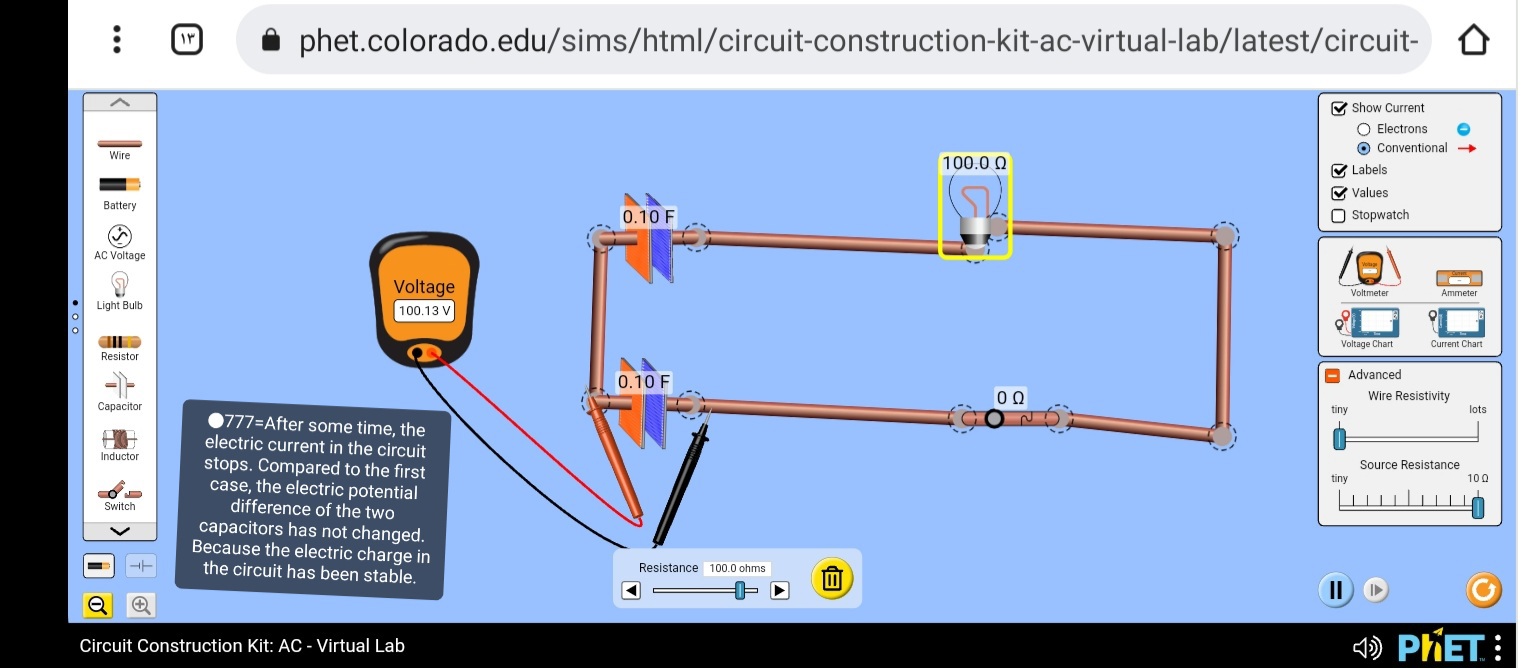
changing the capacity of one of the capacitors must be done continuously and current must be maintained in the circuit By doing this alternately, it can be seen that the current in the circuit is established, but its direction changes alternately. But the total electric charge difference of two capacitors is constant. That is, if we apply the same initial capacity to the capacitor whose capacity we have continuously changed, we will see that the total energy in the two capacitors has not changed in the ratio of the first state.

My question is:

1. Can the energy produced in this circuit be enough to compensate for the energy lost (for example, heat due to the change of capacitor capacity)?
2. If we can compensate for the energy loss, can such a system be used as a sustainable energy source?

3.This assembly consumes mechanical energy to change the capacitance of the capacitor. and produces electrical energy. If possible, specify with a theoretical or experimental example that the mechanical energy consumed is greater or the electrical energy produced

I am looking to understand what is the power dissipation in variable capacitors and whether this idea is practically or theoretically feasible. Any theoretical or practical views on this matter would be very helpful.

Answer 1

You seem to be asking whether your apparatus violates the law of conservation of energy. That is, you seem to be asking whether you can get "free energy" by manipulating those capacitors.

Any time you think you have found a way to cheat conservation of energy, the first question you should ask is, "what is wrong with my model?" Because, almost certainly, there is something wrong with it.

One thing that is wrong with your model (so far) is that you have not accounted for the mechanical force that is required to change the capacitance of your capacitors. Let's say, because it's a simple case that's easy to understand, that your capacitors each consist of two parallel plates, separated by vacuum.† When the capacitor is charged, the two plates attract each other, and an extended version of Coulomb's Law can be used to predict the attractive force between them.

Now comes the part I don't want to calculate...

I imagine that if you did a complete simulation of your apparatus including not only its electrical behavior, but also its mechanical behavior, then when you try to draw a plot of the force between the plates vs. the distance separating them, you will discover a hysteresis loop. Perhaps it will look something like this (wild ass guess):

There will be more force between the plates when you pull them apart than when you bring them back together.

The units on the area, A, enclosed by the loop are $\text{force}\times\text{distance}$—in other words, work (a.k.a., "energy"). Specifically, it represents the amount of energy that is transfered from your hand to the light bulb in each cycle of the machine.

Summary:

Yes. The light bulb will light, just as you say it will. But, the energy to light it comes from your hand or, from whatever mechanism changes the capacitance of the capacitors.

---

† There are other ways to build a capacitor and, other ways to change its capacitance (e.g., you could decrease the capacitance by withdrawing a dielectric from between the plates.) But, no matter how you do it, you always will have to work against some physical force when you want to decrease the capacitance/increase the voltage. However you do it, I'm guessing again, that there will be less force when you restore it to more capacitance and lower voltage.

Answer 2

1. Yes, there should theoretically be a positive energy output when the capacitor's capacitance is decreased. $q=CV$, so if the charge between the plates is to remain the same, the voltage will increase when capacitance is decreased. Once there's a voltage difference between the capacitors, current will momentarily flow.
2. No, it is not a sustainable energy source. Once the voltage across both capacitors is equal, there will be no current flow. Additionally, this scenario requires the capacitors to be charged in the first place.

Answer 3

If two charged capacitors are connected in parallel and the capacitance of one of them changes, a significant current is created in the circuit. If we put a consumer (such as a lamp) in the path of this current, this energy can be used to turn on the lamp.

Note, if you have a load between the two capacitors then they are no longer connected in parallel. The whole circuit then just becomes a RC circuit which discharges with some time constant. Varying the capacitors will change the time constant.

It takes work to change the capacitance of a charged capacitor. It is possible to use that work to power the load, at least for a while. But the source of energy is the work, not the capacitance. That just holds the energy.

Specifically, $E=\frac{1}{2}CV^2$ so if you keep the voltage fixed, then the work required to change the capacitance is $$ \Delta E = \frac{1}{2} V^2 (C_f-C_i)$$ And since $C=Q/V$ we can also write $E=\frac{1}{2}Q^2/C$. So if you keep the charge fixed, then the work required to change the capacitance is $$\Delta E = \frac{1}{2}Q^2 (\frac{1}{C_f}-\frac{1}{C_i})$$

Answer 4

We charged two capacitors with a battery and the electric potential difference of each of them is equal to 100 volts. Then we made this electric circuit using light bulbs and switches.

We double the capacity of one of the capacitors. As a result, its electric potential difference is equal to 50 volts. We close the key. Then the electric current is established in the circuit. And the lamp turns on.

After some time, the electric potential difference of the two capacitors becomes equal. And the electric current in the circuit stops.

We change the capacity of the capacitor again and its electric potential difference changes. As a result, electric current is established in the circuit. And the lamp turns on.

After some time, the electric potential difference between the two capacitors is equal again and the electric current in the circuit is cut off.

We change the capacity of the capacitor and the capacity of the capacitor is equal to the initial state. Again, the electric current is established in the circuit and the lamp turns on.

After some time, the electric current in the circuit stops. Compared to the first case, the electric potential difference of the two capacitors has not changed. Because the electric charge in the circuit has been stable.