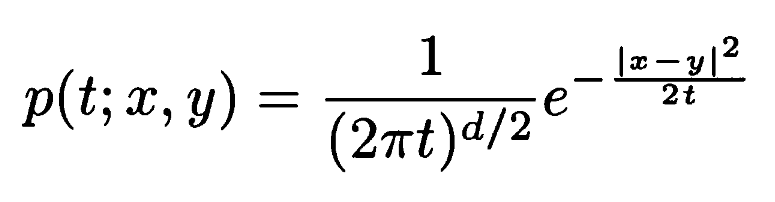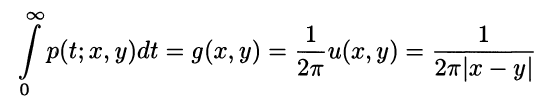Since no one provided an answer, I'll do it. But I don't know what user_1_1_1's mathematical background is, so I will try to give links to every keyword I am using.
The "probability amplitude" you have described is the Heat Kernel. That is to say, the fundamental solution to the heat equation:
\begin{equation}
(\partial_t-\Delta)\Phi=0
\end{equation}
Where $\Delta$ is the Laplacian. One can generalize this "heat equation" by replacing the Laplacian with an arbitrary elliptic differential operator $D^2$ in any dimension. For example, let $D^2 = \partial^2-m^2$ be the Klein-Gordon operator in Euclidean signature. The modified Heat equation is:
\begin{equation}
(\partial_s-D^2)\Phi=0
\end{equation}
Where $s$ is the Schwinger proper-time of the following Worldline formalism we will use to solve this equation. First, let us use the Fourier transform of the Klein-Gordon operator:
\begin{equation}
(\partial_s-[-p^2-m^2])\tilde{\Phi}=0 \Longrightarrow \tilde{\Phi} = e^{-s(p^2+m^2)}
\end{equation}
An immediate corollary is that the integral over the Schwinger proper time is:
\begin{equation}
\int_0^\infty ds\,e^{-s(p^2+m^2)} = \frac{1}{p^2+m^2}
\end{equation}
And this is the propagator of a massive scalar field, in Fourier space, hence my comment to OP.
Now, to find the space-dependent solution, one has two choices: either inverse-Fourier-transforming the solution $\tilde{\Phi}$, or using the Worldline formalism. I do prefer the second approach because I find it more insightful about why integrating the Schwinger proper time gives a propagator (and for its generalization in curved space). So let us proceed a bit formally and rewrite:
\begin{equation}
\Phi=e^{s(\partial^2-m^2)}\delta(x-y)
\end{equation}
This is simply a compact formula for the Weierstrass transform of a Dirac delta. Now, we can write it as:
\begin{equation}
\Phi = \langle y | e^{s(\hat{\partial}{}^2-m^2)} | x \rangle
\end{equation}
Where the hat over the partial derivative operators means we transform a differential operator acting on functions, to an operator acting on bras and kets. Upon treating $\hat{\partial}{}^2-m^2$ like a Hamiltonian $\hat{H}$, we notice that this $\Phi$ looks like an evolution operator. We can give a formula for $\Phi$ involving the standard procedure of Quantum Mechanics for finding the evolution operator using the Feynman path integral formulation:
\begin{equation}
\Phi = \int_{z(0)=x}^{z(s)=y}\mathcal{D}z\,e^{-\int_0^s d\tau\,(\frac{1}{2}\dot{z}{}^2+m^2)} \tag{$\ast$}
\end{equation}
This expression coincides with the position representation of a Klein-Gordon operator. If you are familiar with this expression, it makes a lot of sense for its integral $\int_0^\infty ds\,\Phi$ to be a propagator! Indeed, this is the transition amplitude from $x$ to $y$ in a time $s$, which we integrate over all positive $s$. And this transition amplitude is for a point particle described by the Worldline action $\int_0^s d\tau(\frac{1}{2}\dot{z}^2+m^2)$. In other words, we traded a field the space-time for its configuration, which is a point in the space of fields.
Now, you may wonder "Why did he use a complicated expression just to find the inverse Fourier Transform of $\tilde{\Phi}$?". The answer is simple: the more general you go, the more you can do. Indeed I have first generalized the Laplacian in 3 dimensions to $D^2$, a general elliptic differential operator. Then, I traded the inverse Fourier transform for a path integral. But this Worldline formalism words in curved space too, while the momentum space may not exist is a general curved space. Moreover, the Heat-Kernel in curved space is well understood. And you can, for example, add couplings to other fields.
But the story can go even deeper. Indeed, the path integral is, in general, not well defined. But since our operator $D^2$ is elliptic, the worldline action is quadratic, which means it is a piece of cake when using (co)homological integration
I hope I have clarified why it is not a coincidence that, when integrating the Heat-Kernel over the Schwinger parameter, we obtain a propagator in any dimension, for any coupling, in any curved space.