0
$\begingroup$

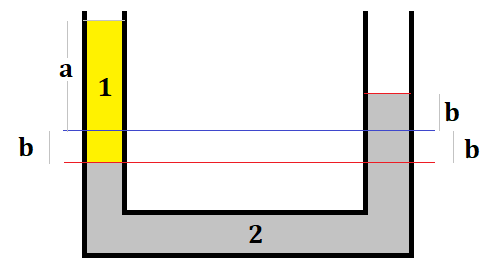
Consider the following diagram.

Because any point in the same level must have the same hydrostatic pressure.

Using the red line as a reference, I have \begin{align}\require{cancel} (a+b)\rho_1 \bcancel{g} &= 2b\rho_2\bcancel{g}\\ \end{align}

But using the blue line as a reference, I have \begin{align}\require{cancel} a \rho_1 \bcancel{g} &= b\rho_2 \bcancel{g}\\ \end{align}

Why are they different? I am really confused.

enter image description here

Improve this question
$\endgroup$
7
  • $\begingroup$ Why is the blue line relevant? If you remove the stuff above that line, the remaining stuff isn't in equilibrium. $\endgroup$
    – PM 2Ring
    Commented Nov 26 at 12:04
  • $\begingroup$ Because any point in the same level must have the same hydrostatic pressure. $\endgroup$
    – D G
    Commented Nov 26 at 12:06
  • 1
    $\begingroup$ “I have [equation]. But…I have [equation]” is not clear. What model and reasoning are you applying to get these equations? I’d expect something like a force balance, or Pascal’s law, or hydrostatic pressure variation. Clarification is also needed as to why you consider the equations to be inconsistent. Why can't two different equations both be true? $\endgroup$
    – Chemomechanics
    Commented Nov 26 at 14:53
  • $\begingroup$ Thank you for editing the question to give the premise. Is the premise true? By subtracting twice the second equation from the first, we find that $a=b$, which means that the liquids have the same density. From this, we conclude that the premise doesn’t hold if the line doesn’t pass through regions of uniform density of a connected fluid. If liquids 1 and 2 have different densities, the red reference line is valid, but the blue one isn’t. Nor, for example, is a reference at the right surface level, which gives zero (gauge) pressure on the right but represents some of liquid 1 on the left. $\endgroup$
    – Chemomechanics
    Commented Nov 27 at 12:37
  • $\begingroup$ @DG "any point in the same level must have the same hydrostatic pressure" - only if the liquid below that level is homogenous i.e. it has the same density at each depth. This is true of the red line, but it is not true of the blue line unless we assume that the two liquids have the same density. $\endgroup$
    – gandalf61
    Commented Nov 27 at 15:54

1 Answer 1

Reset to default
3
$\begingroup$

Because any point in the same level must have the same hydrostatic pressure.

Is the premise true?

By subtracting twice the second equation from the first, we find that a=b, which means that the diagram must not to be to scale and that the liquids must have the same density.

From this, we conclude that the premise doesn’t hold if the line doesn’t pass through connected regions of a uniform-density fluid.

In other words, if liquids 1 and 2 have different densities, the red reference line is valid, but the blue one isn’t.

This should also be evident by considering a horizontal reference at the level of the right surface, which corresponds to zero (gauge) pressure on the right but represents some of liquid 1’s weight on the left. These two values can’t be equal.

Improve this answer
$\endgroup$
9
  • $\begingroup$ So how should the premise be corrected? According to @gandalf61: "only if the liquid below that level is homogenous". I need the proof of this constraint too. $\endgroup$
    – D G
    Commented Nov 28 at 14:26
  • $\begingroup$ The proof ultimately comes from the force balance that relates the vertical pressure differential acting on a small fluid element to the element’s downward weight. A single fluid is assumed, and so the derived relationship $\Delta P=\rho gh$ contains only a single density $\rho$. The blue reference line switches fluids. $\endgroup$
    – Chemomechanics
    Commented Nov 28 at 14:58
  • $\begingroup$ Sorry. I don't understand. The sentences sound confusing. I cannot find the interconnection between each statement. They may be understandable only for experts. Simpler ones please. $\endgroup$
    – D G
    Commented Nov 28 at 15:54
  • $\begingroup$ To help gauge how best to edit the answer for clarity: Are you familiar with how $\Delta P=\rho gh$ is derived? $\endgroup$
    – Chemomechanics
    Commented Nov 28 at 18:43
  • 1
    $\begingroup$ Exactly. The problem with the blue reference line is that the conceptual path no longer moves through liquid 2 alone. However, you could use that path and the same relations to show that that pressure is higher there on the left side by $(\rho_2-\rho_1)gb$. $\endgroup$
    – Chemomechanics
    Commented Nov 29 at 15:44

Not the answer you're looking for? Browse other questions tagged or ask your own question.