As with many concepts taught in introductory physics classes, there are simplifying assumptions that should be better explained to more inquisitive students for them to better understand the full picture. Unfortunately, the price to be paid for this is a little more complication.
For starters, I can't resist putting in a plug for an answer I wrote some time ago to explain that the concept of a "friction $\mu$" is a very rough approximation and should be thought of as a calculation simplification, NOT an accurate description of the real world.
Why are my heavier objects sliding on a smaller incline than lighter objects? Coefficient of Static Friction
Now, let's look at the situation you suggest. All tires behaving equally (an important assumption) so that we can basically assume one generalized contact patch for the vehicle, which is assumed to be a point mass $m$. We neglect COM height off the ground. Also, notably, the vehicle is moving at constant speed $v$ at a radius $r$ with no forward / tangential force required, as already noted in a comment by @naturallyInconsistent. At the limit of frictional ability to keep the vehicle on a constant radius, we have:
Centripetal Force = friction coeff * Normal Force
$m v^2/r = \mu \ m \ g$
So as noted by @gandalf61 we need a square root sign to solve for the critical velocity which I will rename $v^*$
$v^* = \sqrt{\mu \ r \ g}$
Now, let's relax the assumption of no tangential force and see what happens. In other words, the tires need to provide some forward force (to overcome air drag, etc. like you wrote) as well as side force. All tires still behave equally (say, maybe 4WD and all-wheel steering). As you note, the total tractive force available is $\mu N$ in MAGNITUDE but it must be distributed between centripetal and tangential components. So looking from above, the situation is something like the picture below.
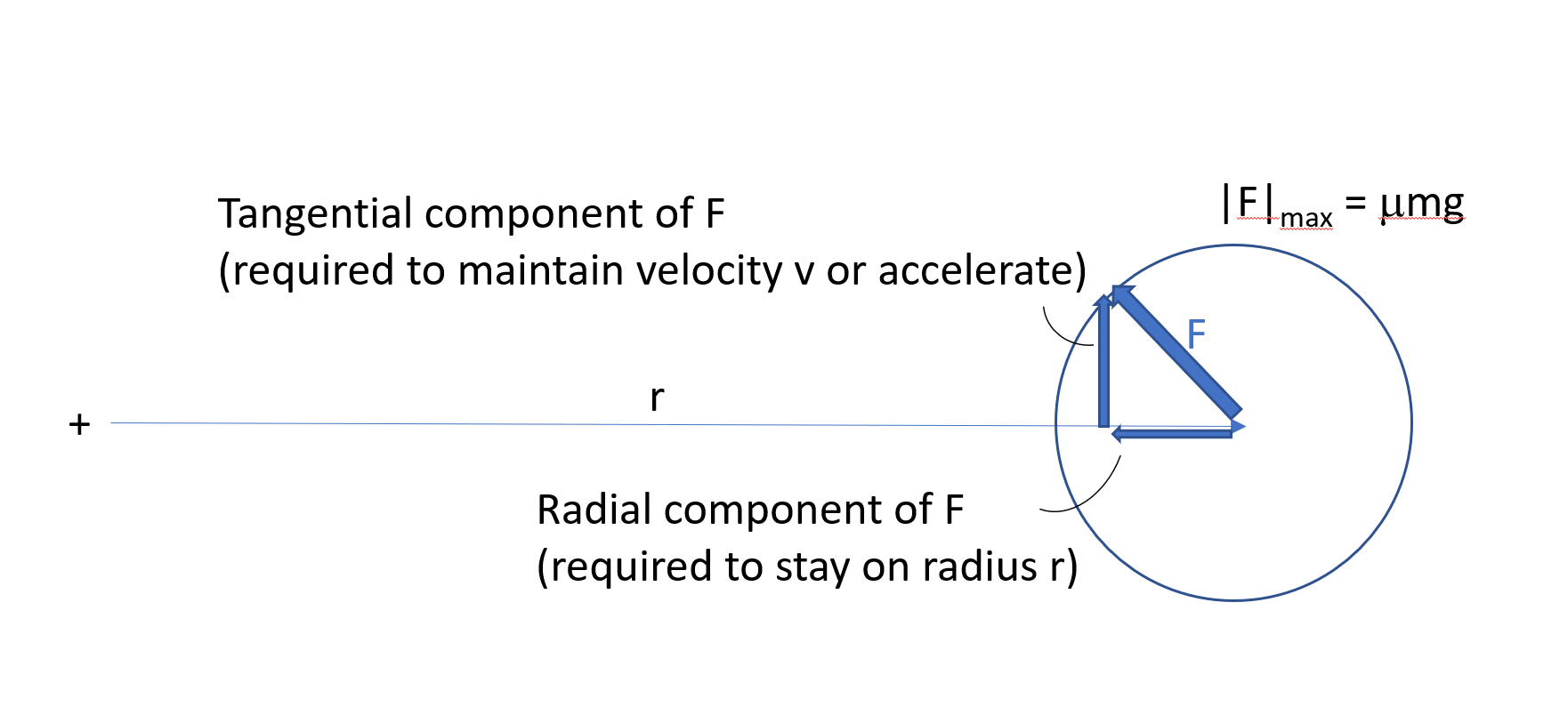
To be clear, the magnitude of $F$ does not have to be EQUAL to $\mu \ m \ g$. It can be less, if the driver doesn't press the gas or allows for a larger radius turn. But it cannot be larger. Once the tip of the $F$ vector is on that max circle, it can only change direction, not get larger. Braking is analogous to acceleration, by the way. The tangential force would be pointing backwards in that case. That's why expert race car drivers usually brake BEFORE they enter a turn - because they want to use that entire $F$ to point purely radially in order to maximize their speed through the turn. They also start wide, cut to the middle, and exit wide - this uses the width of the whole lane to maximize the effective radius. By pressing the accelerator or braking, the driver is modulating the tangential component of the force. By turning, the driver is modulating the radial component of the force.
Now let's relax more assumptions. In the majority of vehicles, either the front or the rear wheels provide the forward force. Because of unequal weight distribution (another assumption) and so-called "weight transfer," the front tires provide more force during braking and actually experience a little bit of unloading during acceleration. The opposite is true for the rear, and there is also a left-right effect during cornering which is noticeable (related to center of mass height). The net effect is that normal force is not equally distributed and each tire actually has its own slightly different max friction circle. The forward or braking force will also differ, especially between front and rear (in particular, non-driven wheels will provide no forward force).
If the driver exceeds the max allowable tractive force with the front tires, there is a situation called "understeer" where the car stops turning and simply plows forward. If the rear tires lose traction, the situation is called "oversteer" and the car tends to spin around (an explanation would require assuming distributed mass and some additional math). Understeer is considered less bad than oversteer, so most modern cars have features which make cars more likely to lose traction in the front instead of the rear under extreme or panic situations.
Additional information can be found here
https://en.wikipedia.org/wiki/Circle_of_forces
ADDENDUM - Answers to follow-up questions
"just to be clear can you mention the nature of frictional forces in each case(as in static/kinetic)"
Little digression here. One of my pet peeves about engineering education which seems to emerge quite a bit in this forum is the misunderstanding that "$F = \mu N$" is some kind of basic law of mechanics when it really isn't. $\Sigma \mathbf{F} = m \mathbf{a}$ is fundamental. $\Sigma \boldsymbol{\tau} = \dot{\mathbf{H}}$ is fundamental. $F = \mu N$ is a very rough approximation that makes it simpler to solve undergraduate physics problems. Separating the $\mu$ into different $\mu_s$ for static contact and $\mu_d$ for dynamic contact as some texts do is yet another crude approximation which actually creates math discontinuities and related problems. So it's fine to use these approximations and terms for rough calculations, but don't treat them as an accurate description of complex phenomena like friction.
But back to your question. In overly simplified terms, the friction between tire and road would be considered static if $|F| < \mu N$ because in a simple way of thinking about it, there is no sliding between the tire and the road. In reality, though, there IS a continuous deformation of the tire that produces an "effective slip" that is described here
https://en.wikipedia.org/wiki/Slip_angle
and here
https://en.wikipedia.org/wiki/Slip_(vehicle_dynamics)
"Also when the road is banked at a certain angle and there is no tangential acceleration,what is the nature of frictional force?"
The above observation remains the same if the roadway is banked, but the forces obviously change.

