Doesn't nonlocality follow from nonrealism in the EPR thought experiment and Bell tests? (Or: How is nonlocal realism viable?)

Question

Original Post, 9/13/2024

It is often said that the Bell test disqualifies "local realistic" theories from quantum physics. But some people emphasize the issue of realism, while others emphasize the issue of locality. For instance, an exchange took place some years ago between Lee Smolin and Jeremy Bernstein; it is emblematic of the controversy I am referring to: Einstein: an Exchange.

The reason this controversy puzzles me is because, at least in my understanding, the either/or forced by the Bell test is not between locality and realism (as Smolin claims, along with many on this site: here and here); on the contrary, it seems to me that locality only becomes an issue because realism is at issue. The conclusion of nonlocality is not independent of realism; in fact, it follows from the denial of realism.

In the EPR thought experiment (on which the Bell tests are based) a pair of entangled particles, A and B are measured at separate, distant locations. Now, according to the Copenhagen interpretation, A and B do not have definite values for their properties prior to measurement. They do not have pre-measurement values. I take this to be a denial of "realism": the particles lack definite "reality" before measurement.

But EPR then bring up the phenomenon of entanglement (i.e., necessary anticorrelation; e.g., when A is spin up, B must be spin down) and point out that Copenhagen cannot account for the anticorrelations predicted by entanglement---unless it claims that the value that A takes at measurement somehow affects the value that B takes at measurement, instantaneously (or at any rate faster than lightspeed). EPR argue that it is more reasonable to assume that A and B had their values assigned “at birth”, not at measurement. And, inasmuch as quantum mechanics is incapable of telling us what those real, existing values are, it is an “incomplete” theory.

So, for EPR, the issue of locality only arises as a result of denying realism (in the form of pre-measurement values). Bell’s inequality forced the issue by showing that, if we assume there are pre-measurement values (more specifically, if we assume that the values for A and B’s properties are predetermined at their "birth", and not at their measurement) then we are committed to expect certain statistical correlations between measurements, to which a non-realist is not committed and is free to violate. The Bell test shows that the inequality is indeed violated; therefore, we are committed to non-realism, while EPR shows that we are thereby also committed to non-locality.

So, what’s the deal? Do I simply misunderstand the Bell test? Or are those who insist that realism can be rescued by denying locality simply wrong?

For context, while I have reviewed many, many articles and videos explaining Bell’s inequality and the Bell tests (including the original articles by EPR and Bell), the only video that has made complete sense to me is from Brian Greene, who is in turn reporting what he was taught by N. David Mermin: Daily Equation #21. Perhaps that is the source of my difficulty...?

Author's Clarifications, 9/16/2024

The answers so far (especially from @flippiefanus) have helped clarify what I mean by "realism". Ironically, by "realism" I mean theories which do not treat the “wave collapse” as something real, but as merely a representation of the experimenter’s changing knowledge of the situation. The experimenter discovers where the particle really was: the concealing “fog” of the superposition burns away. But those who hold that wave collapse is real are, in regards to the particle, “nonrealist”: before measurement, the particle was in a strange, superpositional, spread-out state. Here, there is a change in the state of the particle, not merely in the experimenter. The particle has no trajectory; it sprung out of the wave function at measurement.

To put my cards on the table, I strongly favor the “realist” view: I prefer for superposition to represent the state of our knowledge, not of the system itself. I have an affinity for Bohmian Mechanics, and I’m excited that people say it still has a chance. But I struggle to believe them, due to my (mis?)understanding of the Bell test.

I hope this may be more clearly illustrated with the following, which was inspired by @KDP 's answer:

Local Realism Alice and Bob each flip a coin. They see the other's result. They then go into separate rooms and write what they each got. They give their answers to Sarah, who checks if their answers match. Obviously, they'll match 100% of the time. But Bell's Theorem disqualifies this approach.

Local Nonrealism Alice and Bob go into separate rooms, and each flips a coin. They still have to guess what the other got, without communicating to each other. Thus, only having knowledge of what they themselves got, they have only a 50% chance of guessing the full result correctly. My (mis?)understanding of EPR was that it disqualified this possibility.

Nonlocal Nonrealism Alice and Bob go to separate rooms, flip their coins, but then communicate their results to each other via walkie-talkie before writing them down. (I take this to be the Copenhagen Interpretation post-Bell.)

Nonlocal Realism E.g., Bohmian Mechanics. Alice and Bob flip their coins in the same room, and move to separate rooms afterwards. They also have walkie-talkies with which to communicate with each other. But this seems redundant; they don't need a walkie-talkie, they already know what the other's answer is. The walkie-talkies (nonlocality) are unnecessary to explain their 100% success rate; that is taken care of by the "realism" part (the fact they flipped their coins before separating).

However, the walkie-talkies would not be redundant if Alice and Bob reflipped their coins once they were separated. In other words, the particles change mid-flight between the source and the detector, while nonlocal influences ensure they nevertheless remain continually anticorrelated.

So I think my question has effectively morphed into: Is this effectively what Bohmian Mechanics requires? Or, alternatively: How is Nonlocal Realism a viable stance?

Answer 1

The thrust of Bell's Theorem is that any classical random variables (which, by definition, satisfy the Kolmogorov axioms) have to satisfy certain inequalities. Some quantum mechanical observables fail to satisfy those inequalities, and therefore cannot be modeled as classical random variables.

That, it seems to me, is the key issue. You can work up verbal descriptions that invoke failures of locality, failures of realism, etc., but these are all just different ways of describing the fundamental observation that quantum observables cannot be classical random variables. People are welcome to their linguistic preferences, but (except of course for the cranks) they're all essentially saying the same thing.

Answer 2

(ADDED 9/16: My much longer response, explaining why there are no "local nonrealistic" models of entanglement, can now be found in this related recent question.)

original response:

You are completely right that the ill-begotten phrase "local realism" has confused many people into thinking they could somehow "save locality" by "giving up realism"... whatever that particular person even means by realism. But it doesn't take long to drill down and figure out that there's no coherent notion of "realism" where this tradeoff makes any sense. The best account of all this is Travis Norsen's piece on the topic: https://arxiv.org/abs/quant-ph/0607057 .

In your answer, you are seeming to interpret 'realism' as Norsen describes in his section 5, 'Metaphysical Realism'. But I think you are phrasing it backwards. Locality doesn't gain traction when one gives up realism. Instead, locality only makes sense if you've already granted some sort of realism; otherwise, there is nothing (real) left to which you can ask "is this behavior local or not"?.

As Norsen clearly explains, you cannot "save locality" by getting rid of reality. If you get rid of reality there's nothing left behind to which a concept of "locality" can possibly apply. An anti-realist hasn't raised issues of locality, they've eliminated those issues from having any meaning.

And they've not just eliminated locality, but really all of physics. As Norsen puts it, "Starting at the beginning, does one accept Metaphysical Realism? If not, there is nothing more to be said– at least, nothing that should be of any interest to physicists."

Answer 3

It is often said that the Bell test disqualifies "local realistic" theories from quantum physics.

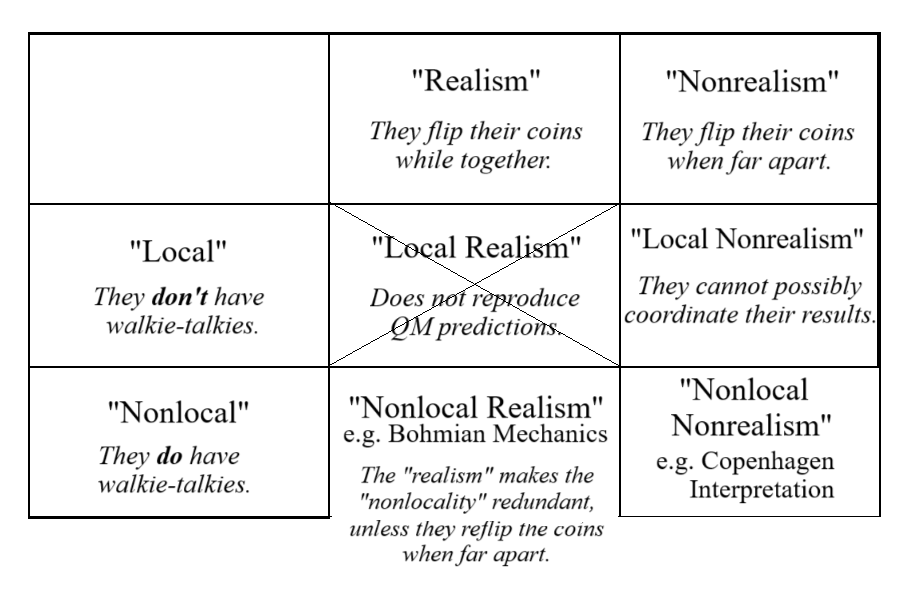
This implies that there are 3 out of 4 classes of theories from the following list that can be compatible with the outcomes of quantum experiments:

Class 1) Local & realistic.
Class 2) Local & non-realistic.
Class 3) Non-local & realistic
Class 4) Non-local & non-realistic.

A lot of people feel uncomfortable with rejecting non-locality, as they (possibly incorrectly) feel it flies in the face of Special Relativity, so they come up with theories that fit into class 2 to try and preserve locality. Personally, since it can be demonstrated that the non-locality of quantum physics does not result in the possibility of superluminal communication at the macro level, it does not bother me in the slightest.

Of course, a lot depends on what we mean by realism. I do not think anyone has ever produced a comprehensive, definitive list of the properties a theory must have in order to be qualified as a realistic theory. Some such lists might include the following:

a) A particle has a definite state at any instant.
b) There is a single universe.
c) Retro-causality is not allowed.
d) The future cannot be predicted with 100% certainty.
e) The evolution of the universe cannot be rewound to a past event, deleting the history of that evolution and then replayed again to create a different history.

... and so on. These properties in this list might be called elements of reality. Casting out any given element does not automatically result in a valid local theory that is consistent with quantum mechanics. Discarding element (a) on its own can not result in a valid local theory. MWI obviously discards element (b) and probably elements (c) and (e) as well. Element (d) is controversial and debatable. Some might argue that element (d) is not an element of reality and that a realistic theory requires that with full knowledge of current conditions, we can predict the future with $100\%$ certainty. Super determinism is a theory that rejects the element of reality (d) and, indeed, such a theory does not require non-local influences and means we have to give up notions of free will. Pilot wave theory or Bohmian mechanics rejects element (c).

Any sensible discussion or analysis of Bell's test requires we define a clear definition of the elements of reality that qualify a theory to be called a realistic theory. (Not necessarily my list).

The conclusion of nonlocality is not independent of realism; in fact, it follows from the denial of realism.

Class 3 type theories (Non-local & realistic) can be consistent with quantum mechanics and do not require the rejection of realism in order to have a consistent and valid non-local theory that agrees with the observed outcomes of real quantum experiments.

Here is an "in your face" quantum thought experiment that makes it obvious that in this experiment, any explanation cannot be both non-local and realistic.

The set-up is very simple, so relatively immune to hand-waving obfuscation. Ann and Bob have polarising analysers, and they are spacelike separated. Each analyser has two detectors. If a photon is detected at detector $\text{H}$, we call the result $1$, and if detected at detector $\text{V}$, we record a $0$ result. We also record the time of detection and the orientation of the analyser for Ann's analyser ($\theta_A$) and Bob's analyser ($\theta_{\text{B}}$). Entangled photon pairs are prepared in orthogonal polarised states and sent in opposite directions to Alice and Bob. Alice and Bob are allowed to rotate their analysers to any position at any time and then read off their results when an entangled photon is received. Quantum mechanics predicts that the correlations of Ann and Bob's results will be $\sin^2(\theta_{\text{A}}-\theta_{\text{B}})$. Any purely local interpretation has to explain how the angle of Ann's analyser ($\theta_{\text{A}}$) is known at Bob's location when Ann and Bob are spacelike separated. It is as simple as that.

Imagine a third party ($\text{C}$) that prepares the entangled photons, and this third party has full knowledge of the sent photons. Let's imagine that when $\text{C}$ sends a vertical photon to Bob, he also sends a message saying: "I have sent you a vertical photon, and I have sent Ann a horizontal photon." When Bob receives the message, he knows the position of his own analyser ($\theta_{\text{A}}$), and he has full knowledge of the states of both the entangled photons at the time of emission, but he still cannot determine the outcome of $\sin^2(\theta_{\text{A}}-\theta_{\text{B}})$ even with full knowledge of the photons states at preparation because he does not know $\theta_{\text{A}}$. Therefore, realism does not rule out non-locality, in this case.

Or are those who insist that realism can be rescued by denying locality simply wrong?

They are not wrong. With non-locality, it does not matter if the theory is realist or not; the theory can be valid either way (Class 3 & 4 type theories.) It insists that the theories are local in nature and require realism (a reasonable description of reality) to be sacrificed.

For example, if Bob has non-local information about the position of Ann's polarising analyser ($\theta_{\text{A}}$) and combines this with his local knowledge of the position of his own analyser ($\theta_{\text{B}}$) then he has all the information he needs to determine the outcome using the correlation relationship $\sin^2(\theta_{\text{A}}-\theta_{\text{B}})$ and this does not rule out realism, but neither does it require it.

Such a realist would simply say entanglement phenomena result from particles having anti-correlated values "from birth", which measurement simply discovers. They have no need for nonlocality. – Michael Pierce

The problem with this approach is that it simply does not work and does not reproduce the results of quantum mechanics as observed in laboratories.

We could list locality as an element of reality (i.e. no event can be influenced by another event that is not in its past light cone), and using this definition, we can cut down Bell's theorem to "No realist theory can reproduce the predictions of quantum mechanics". To reproduce the results of quantum experiments, we have to throw out at least one element of what we would normally call a reasonable description of reality.

The Bell test shows that the inequality is indeed violated; therefore, we are committed to non-realism, while EPR shows that we are thereby also committed to non-locality

You have not explained how you have reached your conclusion. Bell's test allows for the existence and validity of Class 3 type theories (non-local & realistic), so we are not committed to non-realism and also allows for the existence and validity of Class 2 type theories (local & non-realistic), so we are not committed to non-local theories.

KDP I would be extraordinarily interested! ....In any case, what you described is essentially what I'm after, but my (mis)understanding of Bell's Theorem is holding me back. I have no qualms with nonlocality, but I really do not like nonrealism (in the sense of things not having pre-measurement values). And people keep saying these are independent of each other, but that doesn't make sense to me, for the reasons given above. – Michael Pierce

As promised, here is a description where the photons have definite pre-measurement values:

The set-up: There are 3 ways to prepare entangled polarised photons using Parametric Down Conversion, and it is possible to prepare entangled polarised photons that have the same polarisation as each other. Type II SPDC, where the photons are prepared orthogonal to each other, is cheaper and less fiddly, and that is why is most often used in labs and descriptions of experiments. Having the photons prepared parallel to each other is easier to visualise, and that is what I will use here. This means the quantum predictions for the correlation outcomes are according to $\cos^2(\text{A}-\text{B})$ where $\text{A}$ and $\text{B}$ are the orientations of Ann and Bob's analysers, respectively. They both have polarising beam splitters that they use as their polarising analyser. Each beam splitter sends a photon to one of two detectors. One detector indicates the photon was polarised parallel to the optical axis of the beam splitter, and the other detector detects photons that were orthogonal to the optical axis. If a photon is at $45^\circ$ to the optical axis, there is a $50:50$ chance that it will either go to the parallel or the orthogonal detector of the analyser assembly.
There is no requirement to assign a non-detection to an assumed orthogonal detection as would be required for an ordinary polarising filter. Classically when a photon is polarised at $45^\circ$ to the vertical passes through a vertical polarising filter, $50\%$ of 'the light' passes through and $50\%$ is absorbed by the filter. Quantum mechanics says each individual photon either passes through the filter or is wholly absorbed by the filter. The $50\%$ diminution of intensity in quantum mechanics is a statistical summation due to $50\%$ of the individual photons passing through and $50\%$ being absorbed. This probabilistic nature of polarising filters is unavoidable and not up for debate because it is observed in a single polarising beam splitter analyser without involving entanglement or anything fancy like that. For our purposes, here, a detection on the parallel detector is assigned a value of "$1$", and a positive detection of the detector orthogonal to the optical axis is assigned the value "$0$". Either way, the polarising analysers must obey Malus' law. This dictates that if a given photon has an orientation of $\theta_p$ relative to the vertical and the analyser has an orientation of $\theta_a$ relative to the vertical, then the probability of getting a "$1$" result is $\cos^2(\theta_p -\theta_a)$. Alice and Bob are free to rotate their analysers to any position.

Sample run 1: Let's assume at the time of reception, both Ann and Bob's analysers are vertical. QM predicts that the correlation of their results must be unity. Also, assume that the entangled photons are initially orientated at $45^\circ$ from vertical "at birth". Classically, we would expect there to be a $50:50$ chance of getting a $1$ at Ann's analyser and a $50:50$ chance of getting a $1$ at Bob's analyser. There is a chance that their results do not correlate and do not agree with QM. In the non-local interpretation, whatever happens to one entangled photon instantly also happens to the other entangled photon. This is what allows the realism philosophy to reproduce the predictions of QM. If the photon going to Ann's analyser is rotated to the vertical as it passes through her analyser, then she gets a result of "$1$" and instantly, the photon going to Bob's analyser rotates to the same vertical position and is guaranteed to pass through Bob' vertical analyser and produce a "$1$" result. If on the other hand, the photon passing through Ann's analyser is rotated to horizontal (which has a $50\%$ chance of happening), giving a "$0$" result, then the photon approaching Bob's analyser instantly rotates to horizontal and has a $100\%$ probability of being detected as a "$0$" result on Bob's analyser. This means Ann and Bob's detections will be $100\%$ correlated as demanded by QM. Whether one of the photons arrives at Ann or Bob's analyser first does not change the outcome. It is immaterial.

Note that at every moment, the photons have a definite state and location except when they are scrambled at the polarising filters, but after passing through, they have a new but definite orientation. However, when we look at the results, we cannot exactly determine the original orientation of the photons. Nor can Ann or Bob determine the orientation of the other's analyser at the time of reception. While having an exact state at the time of creation is not incompatible with QM, we can not measure that exact state. The so-called superposition of states of the photons in travel can be thought of as a reflection of our inability to measure their exact state.

Sample run 2: Let's assume at the time of reception, Ann's polariser is vertical and Bob's analyser is horizontal. QM predicts that the correlation of their results must be zero. Also, assume that the entangled photons are initially orientated at $45^\circ$ from vertical "at birth". Classically, we would expect there to be a $50\%$ chance of Anne getting a "$1$" at her analyser and a $50\%$ chance of Bob getting a "$1$" at his analyser. If they both get a "$1$", this would be a contradiction with the QM expectation.

Once again, the situation is saved by allowing non-local interaction of the entangled photons. If the photon going to Ann's analyser is rotated to the vertical as it passes through her analyser, then she gets a result of "$1$" and instantly, the photon going to Bob's analyser rotates to the same vertical position and is guaranteed not to pass through Bob' horizontal analyser and produce a "$0$" result. If on the other hand, the photon passing through Ann's analyser is rotated to horizontal (which has a $50\%$ chance of happening), giving a "$0$" result, then the photon approaching Bob's analyser instantly rotates to horizontal and has a $100\%$ probability of being detected as a "$1$" result on Bob's analyser. This means Ann and Bob's detections do not agree, and there is a $0\%$ correlation in agreement with the expectations of QM.

Sample run 3: Let's assume at the time of reception, Ann's analyser is vertical, and Bob's analyser is $30^\circ$ from the vertical. QM predicts that the correlation of their results must be $\cos^2(0-30) = 0.75$. Let's assume the prepared entangled photons are both initially orientated at 60 degrees from the vertical. On arrival at Ann's analyser, Ann's photon has a $\cos^2(60-0) = 0.25$ probability of being detected as a "$1$". If it is detected as a one, Bob's photon is immediately rotated to the vertical position and, on passing through Bob's analyser, has a $0.75$ chance of being detected as a "$1$". Ann's photon also has a $0.75$ probability of being detected as a "$0$" and if that was the case, then Bob's photon would have been rotated to $90^\circ$ from vertical. The photon going towards Bob's analyser would now have a $\cos^2(90-30) = 0.25$ probability of being detected as a "$1$" and a $0.75$ chance of being detected as a "$0$". This means if Ann detects a "$0$", there is a $75\%$ chance that Bob also receives a "$0$". If Ann detected a "$1$", there is also a $75\%$ chance Bob also detected a "$1$". Overall, the chance they either both receive a "$1$" or both detect a "$0$" is $75\%$, which agrees with the correlation probability predicted by QM.

This means we can take a realistic position of asserting the entangled photons have a definite state when created, and the outcomes would not be incompatible with QM, but unfortunately, we can not have everything. We have accepted that taking a measurement with the analysers alters what we are measuring and that the outcome of passing through an analyser is probabilistic in nature, and, of course, this analysis assumes that there is a non-local connection between the entangled photons. Without the non-local connection, the correlations would not be consistent with the predictions of QM. It is interesting to note that the photons passing through Bob's analyser do not have to know the position of Ann's analyser. Without the non-local connection of the entangled photons, we would have to assume something drastic like invoking multitudes of invisible parallel universes or giving particles the ability to retroactively go back into the past and change the orientations of the emitted entangled particles or be able to predict the future positions of the analysers with $100\%$ accuracy.

Final note: Entangled particles are not the only quantum game in town that we have to explain. When we consider experiments based on Mach Zehnder interferometers, they are not explained by entanglement, and the Many Worlds Interpretation begins to gain traction here. That is a different story, though.

Answer 4

Sorry for adding yet another answer to the already long list of answers, most of which provide much valuable information. However, it seems to me that core misunderstanding in the OP question has not been addressed yet.

The reason that the OP claims that the removal of realism inadvertently also removes locality is captured in the statement:

... it claims that the value that A takes at measurement somehow affects the value that B takes at measurement, instantaneously (or at any rate faster than lightspeed).

This statement thus involves the concept of quantum collapse, which is a consequence of using the Copenhagen interpretation.

Remember that interpretations of quantum mechanics are not part of physics in the sense of being products of the scientific method. As David Mermin stated in his Physics Today Commentary titled Quantum mechanics: Fixing the shifty split:

"New interpretations appear every year. None ever disappear."

There are other interpretations that do not involve quantum collapse. Therefore, when the measurement is made they do not require a faster-than-light process taking place. Hence, no violation of locality.

When we consider the expression of a quantum state that consists of a quantum superposition, and then apply a projective measurement on one of the subsystems, we end up with a result that does not say anything about some faster-than-light process. The projective measurement imposes a measurement basis and the state is converted to a form that is expressed in terms of this basis. It does not require any faster-than-light collapse.

Hope my explanation makes it clear.

Answer 5

Quantum theory describes systems in terms of observables represented by Hermitean operators that describe the evolution of multiple possible values of the system in question. This contradicts the idea that there is a single pre-existing value of a measured quantity: realism. The equations of motion in terms of those observables are local: the only way information can be sent from one place to another place is by some physical system carrying that information at or below the speed of light.

If you have two entangled systems $S_1$ and $S_2$ and you measure them the quantum information required to bring about the correlations doesn't change the expectation values of measurements on that system alone: this is called locally inaccessible information. This locally inaccessible information gives rise to the correlations when the results are compared:

https://arxiv.org/abs/quant-ph/9906007

https://arxiv.org/abs/1109.6223

Quantum theory is local and it doesn't satisfy realism.

Answer 6

There have been a slew of absolutely wonderful answers, and I would have to take some time to digest them too, but I have something that might help clarify the historical situation for OP.

This is actually somewhat of a recurring topic plaguing the interpretation of QM that basically nobody gets correct, and especially frustrating that the canonical and definitive historian also got it wrong.

The issue is this: The impression that relativity causes on people who understand it, is that one's understanding of the universe is vastly expanded because of it. The opposite is true of quantum theory; the pioneers definitely all had doubts about whether the quantum theory as put together last century, is the correct final picture to discuss.

As such, it never occurred to Einstein et al that the impression that relativity brought to the table, could even be wrong!

This is actually important to the understanding of the history of quantum theory, because even in the all-important 1927 Solvay conference, the pioneers really did not understand what Einstein was talking about. When you pick up the actual conference proceedings (this links to a great book with commentary; if you want to buy it, be careful that this is quantum theory at the crossroads, not quantum mechanics; that is a different book), you will realise that the entire Bohr-Einstein debates had been Bohr and gang not realising that Einstein was trying to convey that QM conflicted with SR. He simply did not have the terminology of ``locality" to convey this conflict to the rest of them. I mean, Einstein had a 20-year head start thinking about these issues, and it was very frustrating seeing that the Copenhagen gang totally miss what it is that Einstein was talking about, and then (likely inadvertently) smearing him after that conference.

It is only after EPR that it became clear that Einstein had been concerned about locality all along. That is, Einstein took the ``no-superluminal communication" as an unstated assumption, i.e. locality, and in particular, also unstatedly assumed that non-local correlations are also outlawed, and then derived that quantum theory, as currently stated, is non-real, using that as to claim that quantum theory is incomplete, where another assumption is that non-real theories are incomplete. That's the historical context in which all of those arguments would make sense.

Sadly, by then, the Copenhagen gang had already solidified their characterisation of the Bohr-Einstein debates, leading to the historians getting this nuance wrong.

---

As you now know, when Bell's tests are actually done, in a sense, it pits QM and SR at loggerheads to the experimental test, and it was QM that emerged intact, and whatever misunderstandings we had, it would have to fall on the SR side.

That is, if you want to have a hope of even understanding how we currently stand on all these issues, you must NOT make the same mistake as Einstein et al in the EPR paper of assuming SR outright.

After all, the celebrated spin-statistics theorem of QFT is also the same one that asserts that QFT is in agreement with causality and locality; in particular, superluminal communication is impossible under QFT. That is not in disagreement with non-local correlations; whatever is your preferred interpretation of QM, you must be able to take these facts into account. For the purposes of these interpretation discussions, the union of SR with QM in the form of QFT is to be considered complete and successful. (It should be needless to say, that if you are interested in how the spin-stats theorem relates to this issue, you should consult the standard text on this, namely the PCT, Spin and Statistics, and All That)

---

Up to this point, it should be clear that the above already required that the reader distinguish between local interactions as opposed to non-local correlations, with the two things as not being in conflict, since both of these are true as far as physics currently stands.

However, there is also quite a need to be careful of what ``realism" means too. I mean, taking the position of my enemies the Copenhagen interpretationists (lol), the experiments have already ruled out the possibility of "even before measuring, the variables have one specific value for every spacetime event (we could have put the detectors at)". Whether this is interpretation-dependent or not, is not of acute interest to me right now.

Instead, there is also a realism that you can choose, say, between whether the quantum wavefunction is real or epistemic: is the wavefunction just reflecting experimenter's information, or does it refer to the real world in some sense? Please do not assume that people are on the same page on this. (I know your edited question already made this clear.) Similarly, there are other realism definition and questions that one would have to be careful to distinguish.

Amusingly, the "flipping coins near or far apart" picture that the OP new edit puts, makes the realism question also a non-locality question. This is, again, unfortunate and conflating the two things, but also demonstrates how difficult it is to just state the issues involved. I refer the OP to KDP's 2nd answer for a discussion of how this link to non-locality could be resolved by some interpretations. But technically, realism or not is a separate question from locality or not; after all, we have examples of theories in all 3 quadrants as yet unfalsified by experiment, signifying that there must be independence.

Answer 7

Apologies for this 2nd answer, but it is a response to the updated question, and my original answer is already far too long. In the updated version, the OP introduces a thought experiment that involves tangible objects to try and get a grasp on the possibilities. I would like to introduce an alternative thought experiment (I will call the duck and umbrella experiment) that also uses tangible objects to try and illustrate what is going on:

The rules are simple. A messenger with a box is sent to Alice, and a corresponding messenger with a box is sent to Bob. When Alice receives her box, she is allowed to ask the messenger two questions, namely:

$$\text{"What type of object is the box"} \quad \text{and} \quad \text{"What colour is the object?"}.$$

She can ask those questions in any order of her choosing, and then she is allowed to open the box to confirm the contents. The objects can either be an umbrella or a duck (with a 50:50 probability), and the colours can either be red or blue (also with a 50:50 probability). The same rules apply to Bob. A final rule is that if they both ask the same first question, they should get opposite answers as each other, and there is a 50% chance that the answers to their second questions will be the same.

You, as the packager, fill each box with an object of your choosing and send them on their way to Bob or Alice. Your task is to try and duplicate the predictions of QM by your choice of what you put in the boxes. First, you put a blue umbrella in the box for Alice and a red duck in the box for Bob.

Alice asks: "Type, colour?" and the messenger replies: "Umbrella, blue".
Bob asks: "Type, colour?"
and his messenger replies: "Duck, red".

So far, so good. You know, in order to be consistent with the predictions of QM, Bob would expect to get the same colour as Alice 50% of the time if his second question is about colour, so you package a blue umbrella in the box for Alice and a blue duck in the box for Bob. This time

Alice asks: "Colour, type?"
and the messenger replies: "Blue, umbrella".
Bob also asks: "Colour, type?"
and his messenger replies: "Blue, Duck".

You have failed because the answers contradict those of QM. The answers to the first question should never be the same. You cannot succeed, whatever you put in the boxes unless you know in advance what questions Alice and Bob are going to ask and in what order. You cannot emulate the results of QM with real tangible objects with definite states.

How can we reproduce the predictions of QM in this case? One way is to introduce non-locality. When Alice asks the first question, the object in Bob's box instantly changes to whatever is in Alice's box. This might seem a bit of a stretch. How else can we get the correct results? We can invoke retro-causality. In the above experiment, when Bob and Alice both ask what colour the object is as their first question, this change causes a retro-causal influence that causes you, the packager, to pack different colour objects in the boxes. We can also invoke super-determinism. Your act of packing the same colour objects in the boxes somehow prevents Alice and Bob both asking about colour in their first question. We can also invoke superpowers for the packager, such as being able to read the minds of Alice and Bob in the future and know exactly what questions they will decide to ask and pack accordingly. We could assume there are uncountably many parallel universes, and whatever can happen will happen in at least one of those universes, and, somehow, a particular history that is consistent with QM is selected as the one we experience. We could also suppose that some super-being created the entire history of the universe consistent with the rules of QM in advance, like a movie director, and all we are doing is watching the playback, and what is going to happen is inevitable. While no reasonable notion of reality can explain the predictions of QM, the good news is that we get to choose whatever fantastical version of reality that is consistent with QM that we prefer. Whatever version we choose is, by design, unfalsifiable, and our belief is essentially a religious belief based on faith because we cannot prove that version or disprove it.

To me, the least fantastical version is where quantum entangled particles are non-locally connected while they are in an entangled state.

The above thought experiment is justified by the results of the real-life Stern-Gerlach experiment. In that experiment, when particles are passed through a vertical magnetic analyser, half the atoms come out in the "Up" stream (Umbrellas), and half are directed to a "Down" stream (Ducks). If they are then passed through a second horizontal analyser, half are directed to the "Left" (blue), and half are directed to the "Right" (Red). If we take the stream of boxes that contained umbrellas and then take the red route, we can pass them through another colour analyser, and they will always come out as a stream of red objects as we would expect, but if we pass them through a second vertical analyser, half the objects will come out as umbrellas and half will come out as ducks. This means that we started with a stream of boxes that contained 100% umbrellas, and now half of the umbrellas have changed into ducks! Something magical has happened that cannot be reproduced by real tangible objects or a reasonable common-sense notion of reality.

The Stern-Gerlach experiment tells us when we measure a quantum particle, we change its state, and we can never acquire 100% knowledge of its original state. At the first detector, we determined with certainty that the original object was an umbrella, but in doing so, its colour was changed, so the second measurement tells us nothing about the original colour of the object. If we first determined the colour of the object, we would have no idea about what type of object the object started out as. This is a fundamental principle of QM. We can never fully determine the original state of a quantum particle. This is called the principle of complementarity. This means if we believe that a quantum particle has a definite state at creation, this is only a belief because we cannot prove what that state was. This is known as objective realism, as exemplified by Einstein's quote: "I like to think that the moon is there even if I don’t look at it". The belief that quantum objects have no definite state when not being measured is equally a religious belief because it cannot be proven or falsified. Objective realism is equally compatible with QM if we allow non-local interactions.

Answer 8

I agree with most of WillO's answer and some of Ken's. (Ken, I disagree with Norsen on many points, so that is our point of departure.) But a little more on this might help address the OP's question.

Bell assumes a specific definition of realism, and a specific definition of locality.

Realism: there are specific values of quantum (non-commuting) observables independent of the act of observation. In Bell's 1964 paper, see a, b and c after his (14) - "It follows that c is another unit vector..." That assumption is directly contrary to the pure contextualism of the quantum expectation value. Note that merely accepting the orthodox Heisenberg Uncertainty Principle (HUP) is essentially a rejection of Bell realism. The HUP refers to 2 non-commuting observables, while Bell's assumes 3.

Locality: the choice of measurement basis "here" cannot affect an outcome "there" - a distance outside a suitable light cone.

So technically, Bell allows (requires) you to reject one or both of these assumptions. Accordingly, you CAN save locality by rejecting Bell realism, despite what is said in some of the answers. However, the important caveat is that rejecting realism does not really explain anything in and of itself. You are pretty much back where you started, unless you have a non-realistic mechanism to match. And that could in fact involve nonlocality.

---

But... there is a lot more to the story TODAY (2024). In hundreds of experiments, nonlocality of varying types has been demonstrated. These experiments, along with theoretical advances since Bell, pretty well shut the door on any local interpretation of quantum mechanics. Of course, there are some that reject these newer experiments and theory, but that is a subject for another day.

If you want to know the most reasonable manner to describe the situation as of today: both realism and locality must both be rejected. There is not the slightest evidence of the existence of realism (which is a critical element of the Bohmian program, advanced by Norsen et al). And there substantial experimental evidence of nonlocality - as seen in papers by @KenWharton himself (he calls it a "W" setup - I like that Ken; which involves nonlocal entanglement swapping).

Of course, none of this actually describes any reasonable underlying mechanism. And yes, that is frustrating to everyone.

Answer 9

In this context a theory being "realist" means that you can explain measurement outcomes in terms of a hidden variable theory. A local realistic theory is then one which admits an explanation in terms of local hidden variables.

A local hidden variable explanation essentially means that you can explain all observed results as arising from classical correlations. In other words, it means that the probability $p(ab|xy)$ of Alice (Bob) observing the outcome $a$ ($b$) when performing the measurement $x$($y$) is conditionally independent with respect to an additional hidden variable. In practice, this means that there is some information that you could theoretically add to your model that would allow you to predict the measurement outcomes.

One simple classical example of such a situation is if Alice and Bob are given one marble each. Each marble is black and white, and you don't know which who's given which marble. But you know that in total there is one black and one white marble, and thus either Alice got white and Bob got black, or vice versa. In such a situation, the results of Alice's and Bob's "measurements" (which here would mean them looking at the marbles) are correlated (because if Alice sees a color you know Bob will see the other color), but there is information you can use to fully "explain" such correlation (in this simple example this is trivial: if you tell them the colors, then you know everything there is to know). The thing about "quantum nonlocality" is that there are situations where two observers see correlations which cannot however be "explained" in any such classical manner.

It's not true that "nonlocality follows from nonrealism" because you can have a non-realist theory where locality doesn't even enter the picture. For example, quantum contextuality is a form of quantum nonclassicality which you might consider as a generalisation of Bell-nonlocality and that doesn't (necessarily) care at all about locality. YOu can for example derive inequalities which rule out a local hidden variable (i.e. "realist") explanation for the results obtained from subsequence measurements on a single quantum state. For a nice recent review of the topic which covers this perspective see https://arxiv.org/abs/2102.13036.