0
$\begingroup$

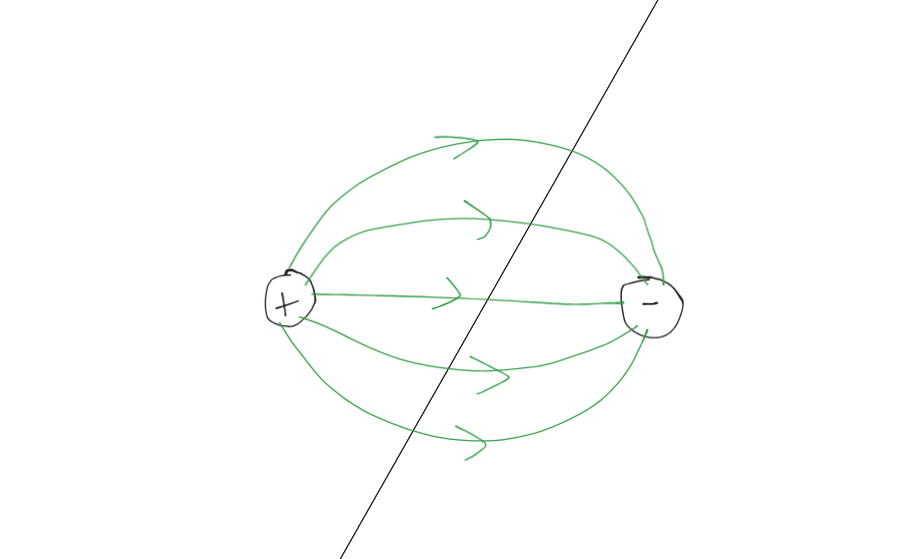
Let the magnitude of the charges be $q$.

Dipole

Since both charges have equal magnitude, every field line emerging from the positive charge will terminate at the negative charge (which can be proven from the fact that flux passing through any Gaussian surface containing both charges is $0$). So the flux passing through every plane which cuts the line segment joining the two charges and doesn't intersect any charge must be the same, since all field lines must intersect such a plane somewhere in their path.

But, what would the magnitude of flux be?

I initially guessed the answer to be $\cfrac{2q}{\epsilon_0}$ by considering Gaussian surfaces (cubes/cuboids) with infinitely long sides (with the plane as one of its faces) and thinking that since the electric field at other faces of the Gaussian surfaces is $0$, all flux from a charge passes through the plane (the flux through plane for both charges would have opposite signs, but they would also have opposite direction for area vector).

But the faces of Gaussian surface at infinity would also have area tending to infinity, so I don't think I can just say that flux through them would be $0$ (this would violate Gauss' law if I consider a Gaussian surface where all face are infinitely far away). So this answer is probably wrong.

Improve this question
$\endgroup$
1
  • $\begingroup$ Do remember that a dipole field also goes to zero faster at infinity than just for that of a monopole field. $\endgroup$
    – Triatticus
    Commented Sep 11 at 16:07

1 Answer 1

Reset to default
3
$\begingroup$

According to Gauss's law the outward flux through any surface surrounding total charge $q$ is $\frac q{\epsilon_0}$. Apply this to the positive charge in your dipole (that's with the negative charge outside the gaussian surface) and you'll see that the flux through your plane is $\frac q{\epsilon_0}$, as we assume that all the flux from the positive charge will end on the negative charge, there being no other charges in the vicinity.

If you apply G's law to the negative charge, you'll find an inward flux of $\frac q{\epsilon_0}$. This crosses the plane in the same direction as the flux from the positive charge crossed it. It is the SAME flux, not another equal flux: we've applied Gauss's law to individual charges; the presence of the other charge doesn't affect the total outward flux through a surface surrounding the charge we're considering, and it won't affect the flux through our plane as calculated from G's law applied to either charge.

You might now object that it's silly to say that the other charge in a dipole doesn't matter. But that's not the argument: the other charge in the dipole ensures that all the flux passes across the plane, going from the positive charge to the negative.

Improve this answer
$\endgroup$
4
  • $\begingroup$ Is thinking about it like two charges individually, where half of the flux emerging from each charge passes through the plane (and the other half diverges to infinity on the other side of the plane) also correct? $\endgroup$
    – brainfreeze
    Commented Sep 11 at 16:50
  • $\begingroup$ I'd say it ISN'T correct, or at least it's not a logical procedure. But what I've just done, for interest, is not to use Gauss's law at all, but to calculate the flux crossing each zone (between radius $\rho$ and $(\rho+d\rho)$, of a symmetrically placed plane. The field strength is found using the inverse square law, remembering that both charges contribute equally. You get the same answer, as by correct application of G's law, namely $q/\epsilon_0$. $\endgroup$
    – Philip Wood
    Commented Sep 11 at 17:14
  • $\begingroup$ Sorry but I don't quite understand that. If there's only one charge in space and an infinite plane at a finite distance to it, shouldn't half of the flux from that charge pass through the plane, and thus the contribution of both charges in the flux should be the equal regardless of whether the plane is symmetrically placed or not? $\endgroup$
    – brainfreeze
    Commented Sep 11 at 17:36
  • $\begingroup$ Oh, I see where you're coming from, and why this argument will give the right answer. I still prefer a single application of G's law, or an argument based on inverse square law field strengths. $\endgroup$
    – Philip Wood
    Commented Sep 11 at 17:49

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.