2
$\begingroup$

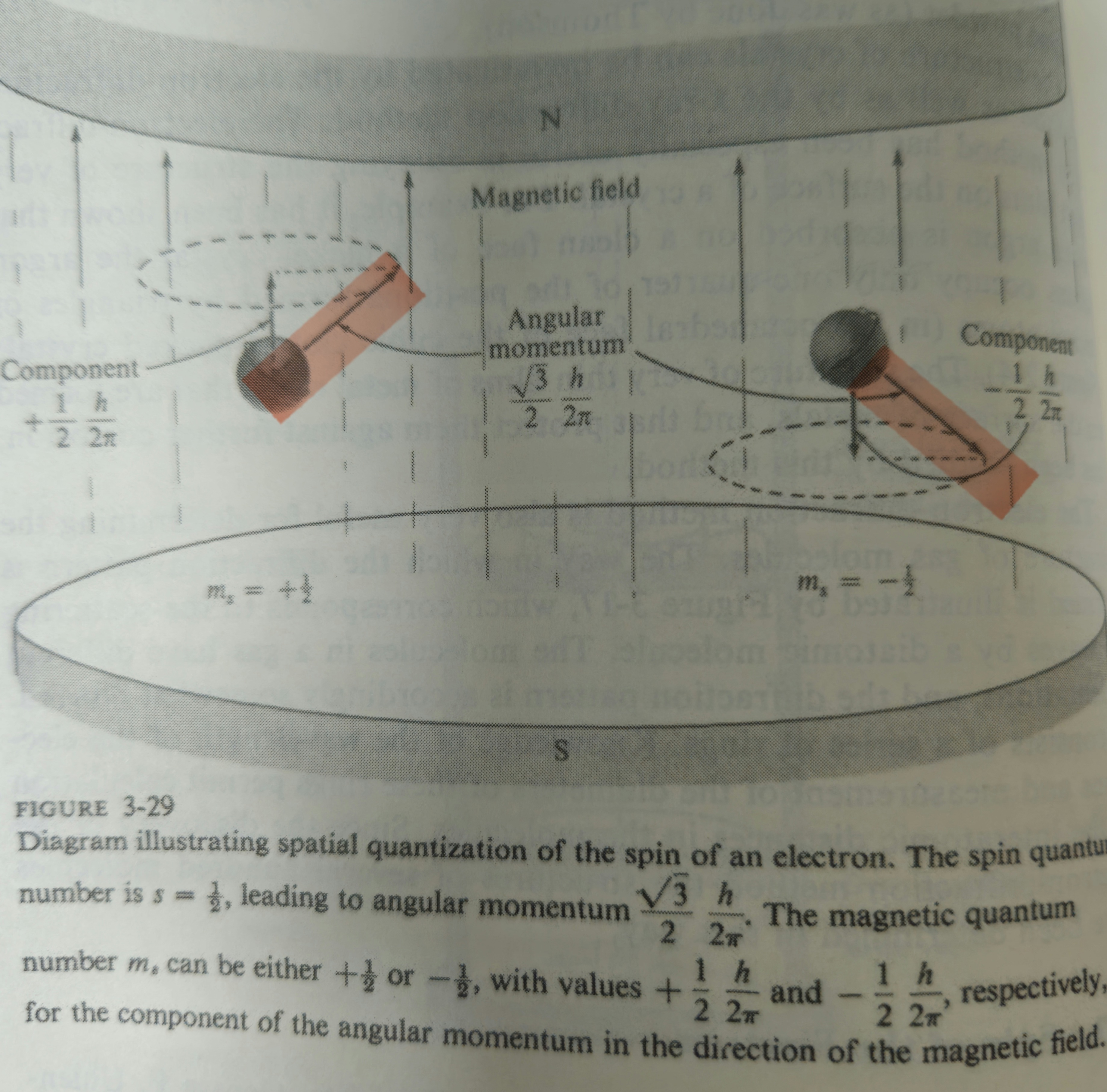
I have a question regarding the interpretation in General Chemistry by Pauling (p. 77 - 78) about the spin of the electron/ it's angular momentum vector direction in a magnetic field:

It was discovered in 1925 by two Dutch physicists, George E. Uhlenbeck (born 1900) and Samuel A. Goudsmit (born 1902), that the electron has properties corresponding to its having a spin; it can be described as rotating about an axis in a way that can be compared with the rotation of the earth about an axis through its north pole and south pole. The amount of spin (angular momentum) is the same for all electrons, but the orientation of the axis can change. With respect to a specified direction, such as the direction of the earth's magnetic field, a free electron can orient itself in either one of only two ways: either it lines up parallel to the field, or antiparallel (with the opposite orientation).

Keeping this in mind, on the next page he shows the following illustration:

enter image description here

It seems to me, that the angular momentum vector does not line up parallel/ antiparallel to the field. Is the previous statement therefore wrong or am I missing a particular word or emphasis?

Improve this question
$\endgroup$
1
  • 1
    $\begingroup$ I believe by the phrase "With respect to a specified direction", and looking at the figure, the book means to say that the dot product of the electron spin with a unit vector in some specific direction can take one of two values: $\pm \hbar/2$. This is weird, classically -- why can't the dot product be zero, sometimes, for example -- but this is part of how quantum mechanics works. $\endgroup$
    – Andrew
    Commented Sep 9 at 11:27

2 Answers 2

Reset to default
1
$\begingroup$

Basically Pauling is considering the total spin angular momentum operator squared $\mathbf{S}^2$, with eigenvalues $\hbar^2 s(s+1)$. For $s=1/2$, like an electron's spin, the squared total angular momentum is $3\hbar^2/2$. So the square root of that would be the "total spin angular momentum", $\sqrt{3/2}\hbar$. I assume he probably introduced orbital angular momentum earlier in the text, which also follows $\hbar^2 l(l+1)$. Then my interpretation of this is that he's using orbital angular momentum as an analogy, but remarking that what one measures is the component of spin in the direction of measurement (the direction of the magnetic field).

I don't think it's meant to be a rigorous explanation.

Improve this answer
$\endgroup$
1
$\begingroup$

The spin can not align itself directly to the magnetic field due to its nature with the half-integer eigenvalues.

So, it does the next best thing: In this classical picture, the spin is precessing around the direction of the magnetic field. I think the dashed circles should depict that (This is the classical effect of a magnetic field on a magnetic moment). You could say that the averaged spin is aligned with the field, which is propably meant by the first statement.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.