0
$\begingroup$

I had this doubt after studying about spring balances. Suppose there's this set-up in which a spring balance on the table is attached to blocks on either sides. 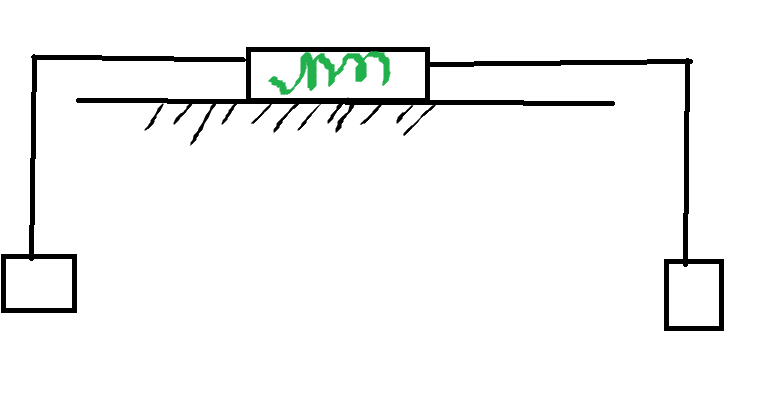enter image description here Something like this. So I wanted to know the reading in 2 cases.

  1. Spring has some mass, smooth surface, unequal masses. I think the tension in both strings will be unequal and it will cause some acceleration in the spring balance. In this case do we calculate the net force on the spring balance?

  2. Same scenario, but friction is present. Now let's assume that the spring doesn't accelerate. Again, do we consider the net force to be the reading?

Improve this question
$\endgroup$

1 Answer 1

Reset to default
1
$\begingroup$

In [the no-friction] case do we calculate the net force on the spring balance?

No. Imagine if the masses were equal. The net force on the spring is zero, it does not accelerate, but the spring's extension is proportional to the weight of the masses.

friction is present ... do we consider the net force to be the reading?

You can make the same argument here. You're never reading net force. What you are reading in this case depends on the geometry of the device. If the weights are unequal, but friction prevents the spring from moving, then there must be a non-zero force from friction. Where is that force applied? Is it to one end or the other on the spring?

Improve this answer
$\endgroup$
2
  • $\begingroup$ Thanks for the answer. It answers most of my question. I still have this doubt. In the first scenario (no friction), say I have a 20kg block on one end and 40 kg on the other. How should I approach this to find out the reading? Should I consider it like the problem involving three blocks, connected to each other by springs? Like I know that the 40kg block will accelerate down, and 20 kg up. The spring will also accelerate to the right (considering that the 40kg is attached to its right end) $\endgroup$
    – entropy
    Commented Aug 15 at 9:23
  • $\begingroup$ As long as the spring is "light", then it is reading the tension in the line. So if you can calculate the tension, you can estimate the (steady-state) reading of the spring scale. $\endgroup$
    – BowlOfRed
    Commented Aug 15 at 15:46

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.