I am currently trying to understand the Lindblad superoperator $$\mathcal{L}[d]\rho = d \rho d^\dagger - (d^\dagger d \rho + \rho d^\dagger d)/2$$ for a simple 2 level system.
In the literature i found, the operator d is seen as a jump operator that can be written in the form $$d = \left|a\right>\left<b\right|.$$
Modeling a system with Hamiltonian $H=0$ (Markov Approximation) and one damping operator we get the Lindblad Master equation $$\dot{\rho} = \gamma \mathcal{L}[d]\rho$$ where $\gamma$ is the damping rate.
For example, with $d=\left| 0 \right>\left<1\right|$ the system will get pulled from $\left| 1 \right>$ to $\left|0 \right>$, just like i would expect when viewing $d$ as a jump operator.

This is no longer the case when using $d = \left( \frac{\left| 0 \right> +\left| 1\right>}{\sqrt{2}} \right) \left< 0 \right|$. I would expect that the system is pulled from $\left| 0 \right>$ to $\frac{\left| 0 \right> +\left| 1\right>}{\sqrt{2}}$. The system will instead get damped towards $\left|1\right>$.
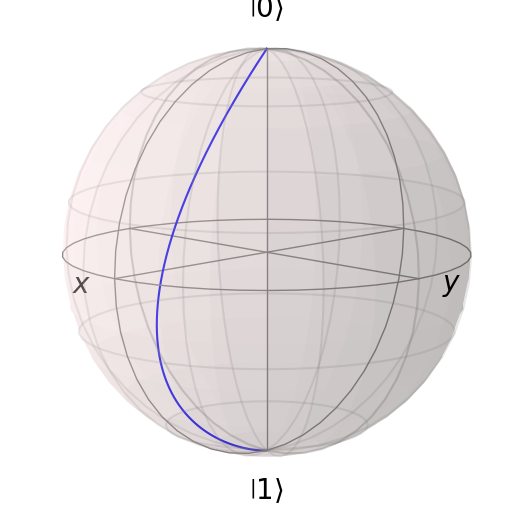
What is going here? Is this view of d as a jump operator only true for basis states? And if so, why? I have read here, that there are not formal constraints on the form of $d$, so how can an operator like $d = \left( \frac{\left| 0\right> +\left| 1\right>}{\sqrt{2}} \right) \left< 0\right|$ be interpretated?
Thank you for your help.
