Position representation of an arbitrary operator and phase space representation

Question

I'm confused with something regarding the rigorous mathematical definition of an arbitrary operator in the position/momentum basis and how it connects to the phase-space representation (https://en.wikipedia.org/wiki/Wigner%E2%80%93Weyl_transform, https://en.wikipedia.org/wiki/Wigner_quasiprobability_distribution#:~:text=The%20Wigner%20function%20W(x,p)%20with%20the%20Wigner%20function).

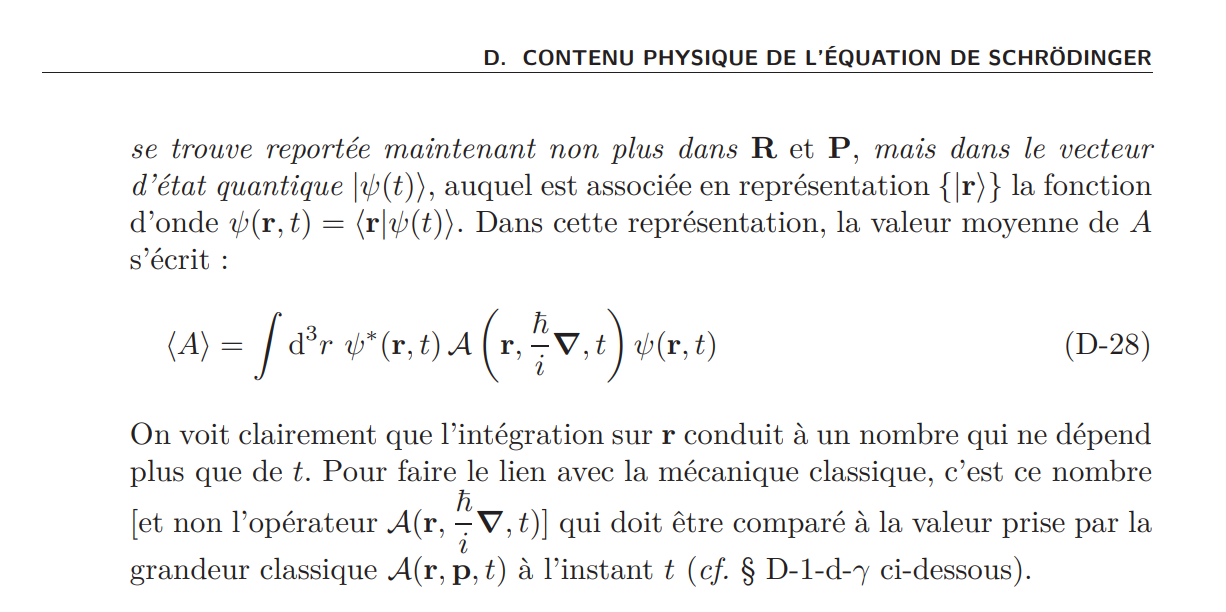
In a lot of quantum mechancis textbooks the quantum average of an operator $\hat A$ for a state vector $|\psi(t)>$ is usually expressed in the position basis (and likewise in the momentum basis) at time $t$ as

$<\psi(t) | \hat A | \psi(t) > = \int_{\mathbb R}dx\psi(x,t)^*\hat A \psi(x,t)$.

This definition fails to satisfy me because it appears to confuse the operators that act on state vectors like $|\psi(t)>$ of the Hilbert space $\mathcal H$ and operators acting on square-integrable function like $\psi(x,t)$. It would make more sense to me, as discussed in Schrödinger equation in position representation if instead we had

$<\psi(t) | \hat A | \psi(t) > = \iint_{\mathbb R^2}dxdy\psi(x)\psi^*(x,t)A(x,y)\psi(y,t)$

with then $A(x,y) = <x|\hat A|y>$ as it would for any other discrete basis.

Now this confuses me because most textbooks I came across that dealt with this (for instance the COHEN-TANNOUDJI) seem to prefer to write the operator $\hat A$ as $\hat A(\hat x)$ arguing that it implies that

$<x|\hat A(\hat x) | \psi(t)> = A(x)\psi(x,t)$

which I intuitively understand because said operator and the position operator $\hat x$ share the position basis as an eigenbasis.

However, in the phase-space formulation of quantum mechanics, objects like $A(x,p)$ and $<x|\hat A | y>$ exist and I can't make sense of the two together with $A(\hat x)$.

Could anyone enlighten me? Can all operators be written in terms of the canonical variables $x$ and $p$? does it make sense to talk about the matrix element $<x|\hat A|y>$? what about $<x|\hat A(\hat x, \hat p) | y>$? Is the standard textbook quantum average legitimate once the matrix is diagonal in the position/momentum basis?

Here is the Quantum mechanics I textbook from Cohen-Tannoudji (the French version but it should be self-explanatory).

Answer 1

I'm not sure where your Hilbert space misconceptions are, but you've definitely made a hash out of phase-space quantization.

In Hilbert space, $$ \hat A = \int\!\! dx dy~ |x\rangle \langle x| \hat A |y\rangle \langle y| , $$ where the matrix elements $\langle x| \hat A |y\rangle $ are indeed functions of two x,y coordinates. Let's stick to the expression of all operators in coordinate space, even though you could easily Fourier transform to momentum space, avoided here, since it confuses you so.

This is true for any operator including momenta, $$ \hat p = -i\hbar \int\!\! dx ~ |x\rangle \partial _x \langle x| , $$ so I would write such operators as $\cal {A}(\hat x, \hat p)$, where $\hat x$ and $\hat p$ are in a nontrivial ordering you must specify, but which is normally unambiguous in simple QM problems not involving angular momenta or exponentials of operators. That is to say, $$ \langle x| \hat p|y\rangle= -i\hbar \partial_x \delta(x-y) ~~~\leadsto \\ \langle \psi|\hat p|\psi\rangle = -i\hbar \int\!\! dx \psi^*(x) \partial_x \psi(x). $$ I have no access to your text, but, as your instructor should have explained to you, patiently, $p(x)$ is not a function of x, but, instead, a non-diagonal operator, as you see above, acting on functions of x and not commuting with x. You are struggling against bad notation, which your text might/should have clarified.

The Wigner map of such operators is a function of two c-number parameters, in phase space. $$ A(x,p)= 2\int\!\!dy ~ e^{-i2py/\hbar}~\langle x+y|\hat A| x-y\rangle . $$

This map is invertible to Hilbert space operators by the Weyl map from phase space to Hilbert space, producing an operator equivalent to the original $\cal {A}(\hat x, \hat p)$, in a special ordering, achievable from the original through Heisenberg's commutation relations.

In this vademecum booklet you may work out through its exercises this full-circle garland of transformations.

---

Illustration edit in response to chat

Consider $\cal{ A}= \hat p \hat x$, to get $$ \langle x | \hat p \hat x |y\rangle = -i\hbar y\partial_x \delta(x-y), $$ etc... for longer concatenations of $\hat x$s and $\hat p$s... Add monomials of every order, to obtain arbitrary polynomials. Do you now see how it works?

_{Note that the subtly different $\cal{ B}= \hat x\hat p$, yields $$ \langle x | \hat x \hat p |y\rangle = -i\hbar x\partial_x \delta(x-y), $$ instead, so that the difference of the two amounts to $-i\hbar$. Thus $B(x,p)= xp+i\hbar/2$ while $A(x,p)= xp-i\hbar/2$. (The Weyl transform of xp is symmetric ordering.) This sort of thing produces the "corrections" of phase-space quantization.}