Let's suppose I have 2 boxes, one with gas molecules in. The other without. I then magically remove the barrier connecting the two gasses. The pressure inside the box and volume taken up by the gas have both changed. But the gas has done no work.
My question is simple, based on the definition of work done by a gas$$W = \int pdV$$ it seems to me like the gas should be doing work, but I know it's not, so I am clearly missing something.
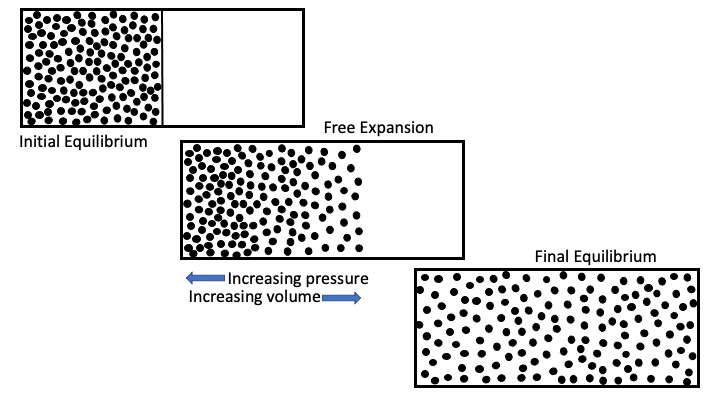
The meta-question is: on what is the work being done? When there was a barrier, particles were colliding with the barrier, they were bouncing off and changing their momentum, which implied that there was a force by the particles on the barrier (known as "pressure") and by the barrier on the particles (that led to the momentum kick).
When the barrier is removed, the particles have nothing on which to push, so they have nothing on which to do work. And there is nothing pushing back on the particles, so there is no work being done on the gas.
It is true, as pointed out in the comments, that $W=\int P(V) dV$ only holds for quasistatic processes, in which the pressure of the gas is approximately constant throughout the gas. This is not what is breaking down in this situation; the gas is not moving so quickly that it can't come to equilibrium throughout itself.** What is breaking down is that this type of work comes from force times displacement, so if there is no force then there is no work (here: there is no pressure). It's like saying "how much energy would it take for a ball moving in space to keep moving in that direction" and the answer is zero, if nothing is stopping it.
** There may be a bit of debate of this point on Wikipedia. As the gas expands into the empty chamber, is the density on the left the same as on the right? You might actually get a gradient of densities initially, as some particles start moving into the empty space, so they say that there might not be full equilibrium throughout the gas. But again, pressure exists as a concept only if there is something on which the forces act, so if you let the particles bounce around for a little bit they'll come to equilibrium throughout the bigger volume without having had to push to get there.
The equation $W = \int pdV$ is for the boundary work done by the system on its surroundings. If the gas is the system, the rigid boxes the boundary, and everything outside the boxes the surroundings, then the gas does no work.
But within the boxes upon removal of the common wall there is work being done by one part of the gas on the other. See the diagrams below. A pressure gradient is produced during the expansion such that the gas towards the left has a higher local pressure than the gas towards the right (the evacuated space). This results in the gas towards the left doing work on the gas towards the right "pushing" into the evacuated space. However, the energy transferred from the gas towards the left equals the energy gained by the gas towards the right, for a net work of zero.
Hope this helps.
Expansion of ideal gas in vacuum doesn't do any work. In dW=pdV p is the pressure of the environment, which is 0, so W=0 as well. Which is logical, the higher the pressure against expansion, the more work is done by expanding some volume.
