How to understand the use of infinitesimals in physics, more specifically in engineering textbooks?

Question

I'll preface by saying that I've gone through many stackex threads and have not found a satisfying answer that would help me understand this. I am truly sorry in advance for the lengthy post, but if you are able to spare a few minutes to help me understand this, it would make a world difference in my understanding!

I am a mechanical engineering grad and I have been struggling with trying to understand how physicists and in particular engineering professors that write textbooks use infinitesimals to come up with formulas.

So far, from what I have gathered by reading on the internet trying to understand the different usage of infinitesimal quantities in math and physics is that physicists, and even more so engineers are not very exact in their usage (according to mathematicians) and that they are using infinitesimals and derivatives in an intuitive way, which helps with getting a result.

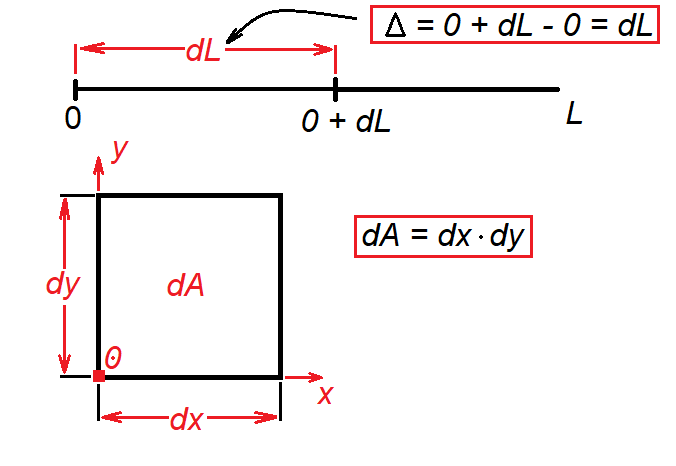
I have watched countless YT videos during my university (unfortunately derivatives and infinitesimals were never explicitly explained in our physics and engineering classes, rather just thrown at us) and many regard infinitesimals and "small amounts" - a small length of wire ds, small amount of charge dq, small amount of mass dm. This was my first issue - I've always considered any quantity starting with a d to be a "change" in some quantity, rather than an amount. But I guess that mathematically it should be one and the same thing - you could have a starting charge of 0, and a final amount of charge of infinitesimally small, and their difference, or a change between those quantities would equal the infinitesimal charge => infinitesimal charge dq = dq - 0. Basically two sides of the same coin.

But what about something like picture related? So we have a differential volume cube: am I correct in my assessment that this dV is just a "change" from a point (0 volume) to a small amount of volume dV? And pressure is obviously supposed to be different on the other side, so that is represented with the change in pressure with partials?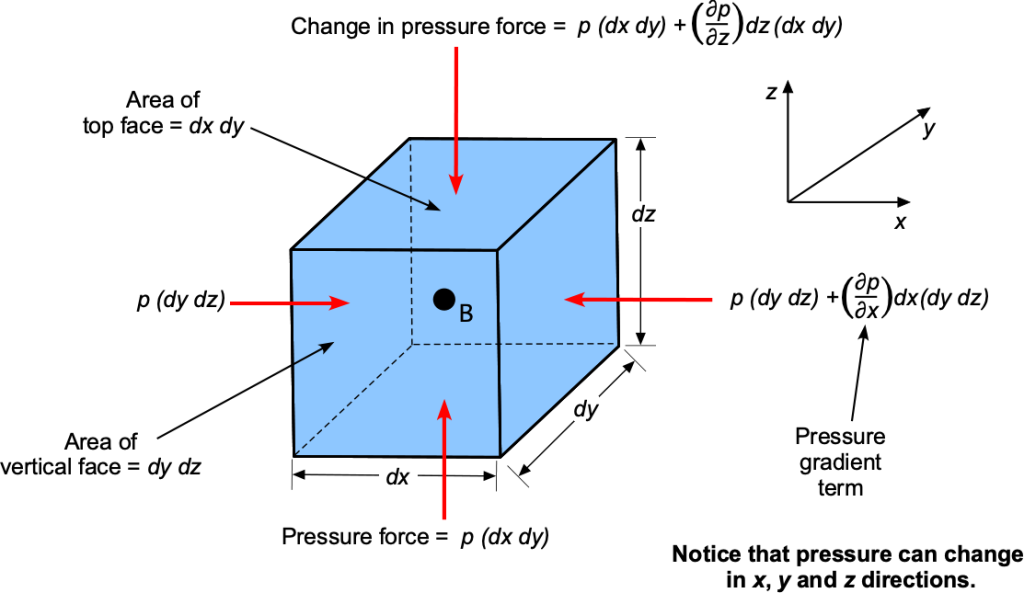

Reason I am so hellbound on this difference between "amount" and "change" infinitesimals is because I have always considered those two to be different, and even found a paper online that explored students' struggles with calculus in physics, where the creators of the paper categorized differentials in different groups, some being "change" and some "amount" differentials. This has given me some sort of an explanation and relief, but I am still not 100% happy.

And one more thing I am trying to wrap my head around is infinitesimal pressure. Pressure is defined as a force acting on a surface. This is average pressure. Then there is infinitesimal pressure, which is pressure at a point, since pressure can obviously vary from point to point in actuality. What I have trouble understanding is the use of differential equation: $$ p = \frac{dF}{dA} $$

I understand intuitively what it is supposed to represent, but not sure how it mathematically makes sense. We take the limit of an area to go to 0, and then we gain insight in how pressure behaves at a single point (which is also counterintuitive but let's leave that for out). But mathematically, it means a "change in force for a change in area"? Or do we come back to the idea of an "infinitesimal amount of force per infinitesimal amount of area", where area is just a change from 0 to dA? I hope I am making sense here, because this should be a really simple case. But whenever I encounter a new differential expression, I am unsure how to understand it. I will attach one more picture for clarity of what I mean in my head when I say amount = change.

Answer 1

First, mathematicians are much pickier about the fine details of logic than physicists and engineers. They have to be. They want to prove statements that are true. They start with axioms that are true. They use that logic that allows you to prove new true statements if you only use true statements. A single false statement could be used to prove other false statements, and the entire structure of math would collapse.

Physicists and engineers are interested in describing the behavior of the universe. They use math for this. But sometimes the description is only an approximation. They do the best they can, but they will use a mathematically iffy shortcut if the outcome matches the universe well.

You can approximate an integral or derivative with finite differences. As the size of the intervals get small, the error gets small. Mathematicians have to take limits and prove that the error remaining is exactly $0$. Physicists can wave their hands and say that if you use an "infinitesimal" size that error is so small we don't care.

The language of infinitesimals has been present since the beginning of calculus. It is a handy imprecise way to think about it. Mathematicians and physicists use it. But mathematicians also prove it rigorously.

---

You can use infinitesimals to represent either a small amount or a small change.

If you are integrating the area under $y=x^2$, you divide the x axis into many small parts where each part is an amount of the x axis. You calculate the area under the curve for each small part by amount times height. You add them all up.

An infinitesimal volume can be a small amount like this. If you want to integrate mass density over a volume, you would divide the volume into many small parts where each part is an amount of volume. You calculate the mass of each part, and add them all up.

A small change would likely be related to a derivative. If you are walking up a hill, the steepness of the hill is a useful property to know. You divide the x axis into many small parts, where each part is a step or change in horizontal distance from the start. Each step results in a change in altitude. Slope is change in height over change in horizontal distance.

Pressure can be understood like this. Divide the area up into many small parts. Calculate the force over an area. Add a small change to the area. Calculate the small change to the total force.

Answer 2

Am I correct in my assessment that this $dV$ is just a "change" from a point (0 volume) to a small amount of volume $dV$ ?

No. $dV$ is simply the volume of the cube, which is assumed to be small - it does not represent a change from anything to anything. The sides of the cube have lengths $dx, dy, dz$ so we have $dV=(dx)(dy)(dz)$. The lengths $dx, dy, dz$ are assumed to be small enough so that the difference in pressure $p$ across, say the $x$ dimension of the cube can be approximated using the first partial derivative of $p$ only i.e. $p(x+dx) - p(x) \approx \left( \frac {\partial p}{\partial x} \right) dx$.

But mathematically, it (pressure) means a "change in force for a change in area" ?

No. Again, $dF$ and $dA$ do not represent a change in force or area - they represent a small force or area. The basic idea is that if we just define pressure as force divided by area then the notion of pressure at a single point becomes difficult since we are dividing zero force by zero area, and the ratio is undefined. However, if we instead measure the force $\delta F$ across a small but finite area $\delta A$ then we can define the average pressure across $\delta A$ as

$\displaystyle p_{avg} = \frac {\delta F} {\delta A}$

and if we then allow $\delta A$ to approach zero then the pressure at a point can be defined as

$\displaystyle p = \lim_{\delta A \rightarrow 0} p_{avg} = \lim_{\delta A \rightarrow 0} \frac {\delta F} {\delta A} = \frac {dF}{dA}$

As noted elsewhere, physicists tend to be more intuitive and less rigorous in their handling of "infinitesimals" and derivatives than mathematicians (in fact, a mathematician would try to avoid the term "infinitesimal" altogether). Mathematicians worry about whether the limit of a ratio as both quantities approach zero is well defined; whether the functions involved are continuous; whether these functions actually have well defined derivatives everywhere etc. Physicists tend to take all these niceties for granted.