Conceptual doubt related to normal force in a two-block system kept on frictionless surface

Question

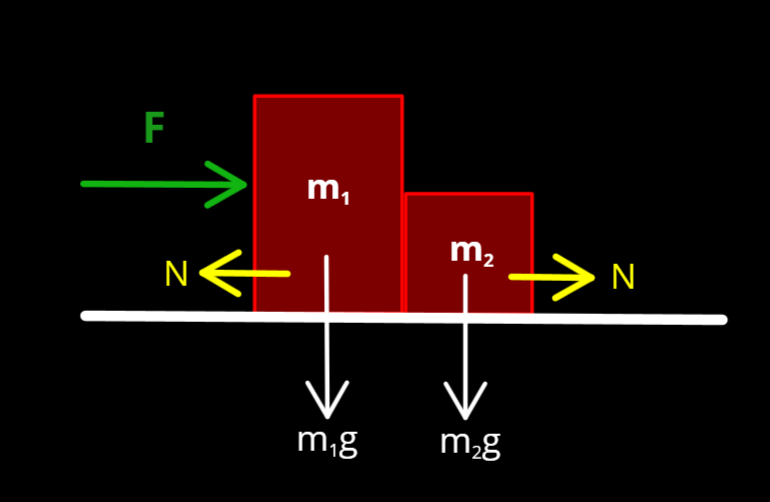
I was solving this question in which we had to calculate the normal force between blocks $m_1$ and $m_2$

Here, two blocks of mass $m_1$ and $m_2$ are in contact on a frictionless surface, and a force F is applied on the larger block. I have shown all the forces which act on them individually.

We can see that as both blocks are in contact, their accelerations must be equal.

So, I wrote the following equations using Newton's second law of motion:

1$)$ $\color{blue}{F - N = m_1a}$

2$)$ $\color{blue}{N - m_2a}$

Adding these we get

$$\color{green}{a = \frac{F}{m_1 + m_2}}$$

And substituting it and solving we get the normal contact force as

$$\color{red}{N = \frac{m_2F}{m_1 + m_2}}$$

My Doubt here:

From the expression, we got for the normal contact we can see that it is less than F. Why did the magnitude of normal force come out to be less than F? Does it have to do something with the fact that the surface is frictionless?

Answer 1

Here are a couple of ways of thinking about it.

The two blocks are accelerating to the right. Consider the block on the left. For it to accelerate to the right the applied force acting on it to the right has to be greater than the contact force on it to the left.

Or, consider the block on the right. The only horizontal force acting on it is the contact force to the right. Compare that to the system of the two blocks moving together. The only horizontal force acting on that system is the applied force. The single block on the left has the same acceleration as the whole system but it has a smaller mass so the net force on it must be smaller. That is, the contact force must be smaller than the applied force.