There is no well established taxonomy of dividing the inspiral into "late" and "early" parts. When people do talk about the "late inspiral" they mean "that part of the inspiral in which the PN approximation is inadequate." Where that is depends on a combination of things including the parameters of the binary (mass-ratio, spins, etc.), the order of the PN approximation, and how accurate you need the final answer.
For equal mass, non-spinning binaries, and if you don't need a particularly accurate answer a 3PN approximation can be accurate enough for the entire inspiral phase (and you need full Numerical Relativity only for the final plunge and merger.)
This changes a lot when the mass-ratio becomes smaller. One of the effects of making the mass-ratio smaller is that the (specific) energy lost to gravitational waves also becomes smaller, and binary evolves slower, completing more gravitational wave cycles during the inspiral. A consequence of this is that you need to know the energy lost per GW cycle much more accurately. Specifically, the accumulated error in the gravitational wave phase scales roughly with the reciprocal of the (symmetric) mass-ratio $\nu$. Consequently, if we want the inspiral to stay roughly in phase all through the inspiral, this means that we can tolerate an relative error in the energy flux that is rough $O(\nu)$.
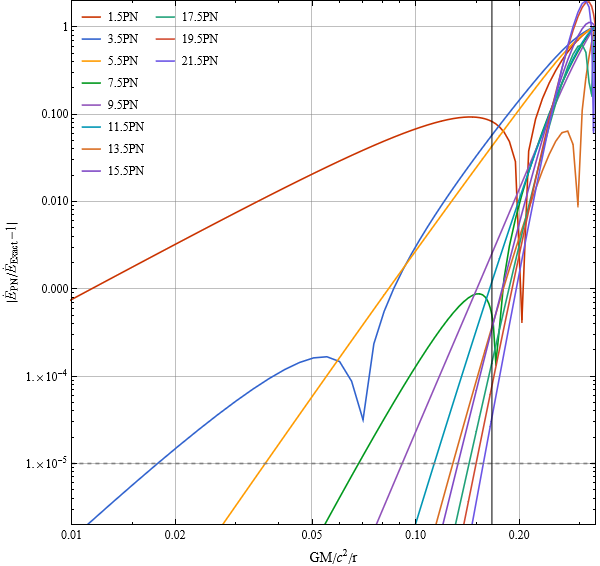
For $\nu=10^{-5}$, the relative error in the 5.5PN flux (chosen simply because I had the plot at hand) for quasicircular non-spinning binaries becomes larger than $10^{-5}$ at a separation of roughly $25 GM/c^2$. So, in this case the part of the inspiral between $25 GM/c^2$ and the last stable orbit ($6 GM/c^2$) can be considered "late inspiral". Since the shrinkage of the orbit is $O(\nu)$ per orbital cycles, this means that the binary $\nu=10^{-5}$ completes $O(10/\nu) \approx 10^6$ orbital cycles in the "late inspiral". These are very rough "back of the envelope" estimates. They can be made more precise by looking at numerical simulations of such inspirals, e.g. using the publicly available fast EMRI waveforms package, which uses interpolate numerical data obtained in the small mass-ratio limit.
Edit:
Below is a plot showing the relative difference between the linear in $\nu$ part of the the energy flux to its $n$PN approximation as a function of the "compactness" ($GM/(c^2r)$) of the binary, using PN approximations available from the Black Hole Perturbation toolkit, and numerical data I generated myself.