The number of windings is not written in the magnetic flux formulas in high school textbooks, but I come across articles saying that the number of windings affects the magnetic flux formulas. Is this true or not?
-
$\begingroup$ Please clarify your specific problem or provide additional details to highlight exactly what you need. As it's currently written, it's hard to tell exactly what you're asking. $\endgroup$– Community BotCommented May 31 at 18:39
-
$\begingroup$ Related : How to calculate flux in a helical wire?. $\endgroup$– VoulkosCommented May 31 at 19:33
-
$\begingroup$ And another related post: Magnetic flux linkage of a solenoid is equal to BAN, but what is A representing? $\endgroup$– FarcherCommented Jun 1 at 8:06
2 Answers
You need to focus on the surface across which you compute the magnetic flux: a coil with $N$ windings can be thought as $N$ circular surface.
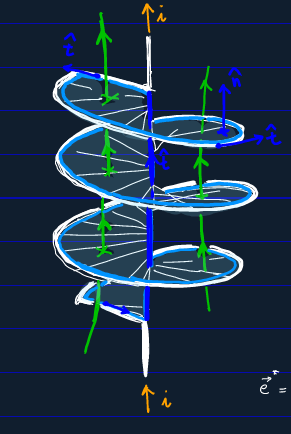 [Image taken from here]
[Image taken from here]
Let's call $\Phi$ the flux across a section of the coil, and $\Psi$ the linkage flux across. Neglecting dispersed fluxes, coil linkage flux $\Psi$ is $N$ times the flux through $\Phi$ one of these sections,
$$\Psi = N \, \Phi \ ,$$
being $\Phi = B \, A$, with $A$ the section of the coil, and $B$ the uniform magnetic flux inside the coil, that can be evaluated (using Ampére law in steady conditions) as
$$B \, \ell = \mu \, N \, i \qquad \rightarrow \qquad B = \mu \, \frac{N}{\ell} i$$
with $N$ number of windings, $\ell$ coil length, $\frac{N}{\ell}$ number of winding density, $i$ the current in the inductor, and $\mu$ the permeability of the medium in the core of the coil.
Putting everything together and using Faraday's law on the coil, the voltage across the ends of the coil is
$$v = \dfrac{d \Psi}{dt} = \frac{d}{dt} \left( N \Phi \right) = \frac{d}{dt} \left( N A B \right) = \frac{d}{dt} \left( \mu \frac{A N^2}{\ell} i \right) = L \dfrac{d i}{d t} \ ,$$
being $L$ the inductance, here assumed to be constant.
-
$\begingroup$ I'm sorry for asking the question wrong, my question is this: If we put a solenoid with no current flowing through it in the appropriate direction to an area that already has a magnetic field, will the magnetic flux of this solenoid depend on the number of windings? $\endgroup$ Commented May 31 at 18:54
-
$\begingroup$ Let's do this way. Check if this answer is ok for this "wrong" question, and upvote and accept if ok and then open a new question. And add the link of the new question in comments here below. Short answer to the new question: read the first lines of this answer. It depends on the surface across which you evaluate the flux $\endgroup$– basicsCommented May 31 at 18:56
When talking about coils, the term magnetic flux is somewhat ambiguous without context. For any given surface $S$, bounded by some loop $C$, we can define it as the surface integral $$\Phi = \int\limits_S \vec B\cdot d\vec A.$$ This can be thought qualitatively as the number of magnetic field lines through $S$. The ambiguity arises from which surface $S$ (or loop $C$) one has in mind.
Consider the cross-section $S_1$ of this core somewhere along a coil with $N$ turns. We can call the flux through it $\Phi_1$. If the coil is sufficiently small, or is wound around a magnetic core which is good at confining the magnetic field within, $\Phi_1$ is roughly the same wherever you take the cross-section $S_1$.
Next, we take $C$ to be a circuit loop that runs along the conductor of the coil. The surface $S_2$ bounded by it is very complicated, but it essentially intersects the magnetic core about $N$ times. Therefore, the flux through $S_2$ is $\Phi_2 \approx N\Phi_1$.
If the magnetic field is created by some other source, then $\Phi_1$ doesn't depend on $N$, but $\Phi_2$ is approximately proportional to $N$. In the context of magnetic circuits and coils, $\Phi_1$ is often referred to as the flux (or the flux in the core if there is a magnetic core), and $\Phi_2$ as the flux linked by the coil.
