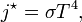The change of entropy is defined $$\Delta S = \int \frac{dQ_\mathrm{rev}}{T}.$$ If a system is isolated the heat transfer between the system and the surroundings is zero ($dQ = 0$), thus $\Delta S = 0$.
However, it is commonly stated that the entropy of an isolated system can increase. How is this possible, given the above definition of entropy?
One reason is that different parts of the system can be at different temperatures. If a part of the system at $T_1$ transfers an amount $\Delta Q$ of heat to a part of the system at $T_2<T_1$, the hot part's entropy changes by
$$\Delta S_\text{(hot)} = -\frac{\Delta Q}{T_1}$$
but the entropy of the cold part changes by
$$\Delta S_\text{(cold)} = \frac{\Delta Q}{T_2},$$
so the total entropy change is
$$\Delta S = \Delta S_\text{(cold)} + \Delta S_\text{(hot)} = \Delta Q \left( \frac{1}{T_2} - \frac{1}{T_1} \right) > 0. $$
Another reason, as Ignacio Vergara Kausel pointed out, is that entropy changes can also occur for other reasons than heat flow. For example, chemical reactions can change a system's composition, which affects its entropy.
Because that's not the whole picture and the entropy of a system can change by other means. A more complete picture can be seen here.
The definition of entropy $$dS = \frac{\delta Q}{T}$$ only applies for reversible processes. For every irreversible process, $$dS > \frac{\delta Q}{T}.$$ Therefore, if the sytem is isolated ($\delta Q = 0$), and an irreversible process occurs, $dS > 0$.
Simple irreversible processes include friction, mixing, and heat transfer accross a finite temperature difference.
An isolated body in vacuum will radiate continuously and loose energy by the black body radiation :
j is the total power per unit area radiated away
and as it radiates it cools. An estimate of the time taken for cooling is here.
The law that entropy increases or stays constant can be applied only to closed systems. The closed system in this case is the body radiating + all the photons radiated. The entropy of the body decreases, but the whole system's entropy increases since the number of infrared is enormous and continually increasing. The statistical expression of entropy is applicable here, and not the thermodynamic one, because the system body+radiation does not have one temperature.
It is worth noting that the entropy of an isolated body cooling with black body radiation decreases for the body. If it is made of crystallizable material it will crystallize and be highly ordered. The same is true for all living matter. A cell lives by continually decreasing its own entropy, but raising the entropy of cell+surroundings.
