2
$\begingroup$

I've been thinking of this proof of the 3rd law from the 2nd law. The proof is as follows.
We have a compound object made of two smaller objects, each of mass km and m so that the total mass is (k+1)m. We have a force exerted on the mass and its overall acceleration is $$a = \frac{F}{(k+1)m}$$.

enter image description here

Now we take a look at the constituent particles. Each will have the same acceleration as the whole, a. For a particle of mass m, the resultant force required to obtain an acceleration of a will be $$a = \frac{F}{(k+1)m} \times m = \frac{F}{k+1}$$
To have this resultant force for mass m, the mass km must apply on it a force of
$$F_1 = F-\frac{F}{k+1} = \frac{Fk}{k+1}$$

Now let us consider the other mass km. For km to have an acceleration of $\frac{F}{(k+1)m}$ the force needed is $\frac{Fk}{k+1}$. This is the force that the mass m exerts on the mass km. As we can see, the two forces are equal and act in opposite directions.

Improve this question
$\endgroup$
4
  • 1
    $\begingroup$ I've always viewed the third law more as being related to the conservation of momentum in a closed system. $\endgroup$
    – Cameron Williams
    Commented Apr 13 at 17:21
  • $\begingroup$ That’s exactly what the third law is. It is just the law of conservation of momentum in action. $\endgroup$
    – Matt Hanson
    Commented Apr 14 at 0:48
  • $\begingroup$ Yeah the law of momentum conservation is derived from the three laws. Corollary III of Newton's original Principia. $\endgroup$
    – Zamil Hoque Siddique
    Commented Apr 14 at 10:11
  • $\begingroup$ You're applying the second law in a way which assumes 3rd law holds. 2nd law says net impressed force on the combined body equals $ma$, but it does not by itself say this net force equals the external force $F$ - there may be other forces contributing to net impressed force, e.g. those due to mutual interaction of the two balls. Assuming net impressed force equals the external force is equivalent to assuming the internal forces sum up to zero, thus assuming the 3rd law. $\endgroup$
    – Ján Lalinský
    Commented Apr 17 at 0:11

4 Answers 4

Reset to default
4
$\begingroup$

In obtaining your first equation, you have used Newton's 3rd law implicitly, so your argument is circular.

Newton's 2nd law is a statement about the motion of individual particles. On its own, it has nothing to say about systems of multiple particles or the interactions between them. Suppose we try to apply it to your scenario, thinking of the "compound object" as a system of two particles with masses $m_1 = m$ and $m_2 = km$, constrained to move so that $a_1 = a_2 = a$. We then have two Newton's 2nd law equations for the two particles, \begin{align} F + F_{21,x} = m_1 a\\ F_{12,x} = m_2 a \end{align} Without Newton's 3rd law, we can conclude, at most, that \begin{align} F + F_{21,x} + F_{12,x} = (m_1 + m_2)a\\ \end{align} so \begin{align} a = \frac{F + F_{12,x} + F_{21,x}}{m_1 + m_2}\\ \end{align} In other words, we have no way of calculating the acceleration of the system unless the forces $\vec{F}_{12}$ and $\vec{F}_{21}$ are specified. It is only once we use Newton's 3rd, which tells us that $\vec{F}_{12} = -\vec{F}_{21}$, that we can say \begin{align} a = \frac{F}{m_1 + m_2}\\ \end{align} as you have.

You might argue that since we observe the acceleration of the two masses to be $F/(m_1 + m_2)$, your first equation has to be true. But this is not something we get from Newton's 2nd law. It's an additional piece of experimental evidence, one which we can use to argue in favor of Newton's 3rd law $\vec{F}_{12} = -\vec{F}_{21}$ holding true.

Improve this answer
$\endgroup$
1
  • $\begingroup$ But Newton argues that the force is proportional to the rate of change of motion or F=ma, so cant' we just use the idea of the inverse proportionality of mass and acceleration to predict the acceleration for compound bodies? Because won't we otherwise have to start talking about fundamental particles then? But was the second law actually stated in terms of fundamental particles? $\endgroup$
    – Zamil Hoque Siddique
    Commented Apr 14 at 10:29
3
$\begingroup$

Your argument works through one case where you can demonstrate that the forces must be equal. Newton's law states that all cases must satisfy this property.

Consider a case where the objects do not all move in a straight line. Perhaps the circles are not exactly in line, such that one of them is going to veer upwards and one will veer downwards when the force is applied. Now we can no longer assume that $a$ is the same for both bodies, so the reasoning you had for your special case will not be the same as the reasoning for this new special case.

Newton's 3rd law states that forces always come in equal and opposite pairs. This is necessary to predict the motion of systems with arbitrary configurations, including your example and my example.

Improve this answer
$\endgroup$
3
  • $\begingroup$ In a situation as yours where the spheres are not in line, we can still derive that the forces will be equal and opposite by using the idea of rotational symmetry if velocities are equal. If the velocities are different, we can change our perspective to make the velocities equal (the observer in this case has a veloctiy). Then, we use the idea that velocities can be treated as vectors and the observer's velocity can be added to the observed velocities of the particles to get the actual ones. A bit of geometry will then show that the changes in velocity are equal and opposite in direction. $\endgroup$
    – Zamil Hoque Siddique
    Commented Apr 14 at 10:47
  • $\begingroup$ Since the time is the same for both, the forces are equal. But this only works for equal masses. $\endgroup$
    – Zamil Hoque Siddique
    Commented Apr 14 at 10:48
  • 1
    $\begingroup$ @ZamilHoqueSiddique If we go down that path, we lose one of the insights of Newtonian physics, which is that all inertial reference frames are equal in stature - there is no privileged frame. And, we can always make the situation more interesting by adding a 3rd sphere. There will be an observer that makes all velocities equal only in very special cases. $\endgroup$
    – Cort Ammon
    Commented Apr 14 at 20:53
0
$\begingroup$

About Newton's third law:

Instead of making use of the historical formulations of Newton's laws of motion I want to make a fresh start, laying down assumptions to be granted.

Law of motion 1.
Space and time are euclidean, position vectors, velocity vectors and acceleration vectors add according to the euclidean metric (that is, according to Pythagoras' theorem), inertia is uniform throughout space, an object that is not subject to a net force will in equal intervals of time cover equal intervals of distance.

Law of motion 2.
$F=ma$


So 'Law of motion 1.' is a lot of words here, but there is a concise way of stating it:

Space, time, and inertia are according to the euclidean metric.



The concise version:
Law of motion 1: space, time, and inertia are according to the euclidean metric.
Law of motion 2: $F=ma$

The reasons for proposing this form:

Ever since the introduction of relativistic physics we know that even though we still start with teaching newtonian mechanics, we need the ability to transition to relativistic physics.

We have that in terms of special relativity the metric of spacetime-and-inertia is the Minkowski metric. That is, in terms of special relativity the 'Law of motion 1.' is: Minkowski metric.

In terms of pre-relativistic theory of motion: the 'Law of motion 1.' is: euclidean metric, and we need to assert that space and time stand in a relation to each other: an object in inertial motion will in equal intervals of time cover equal intervals of distance.


Now a thought experiment:
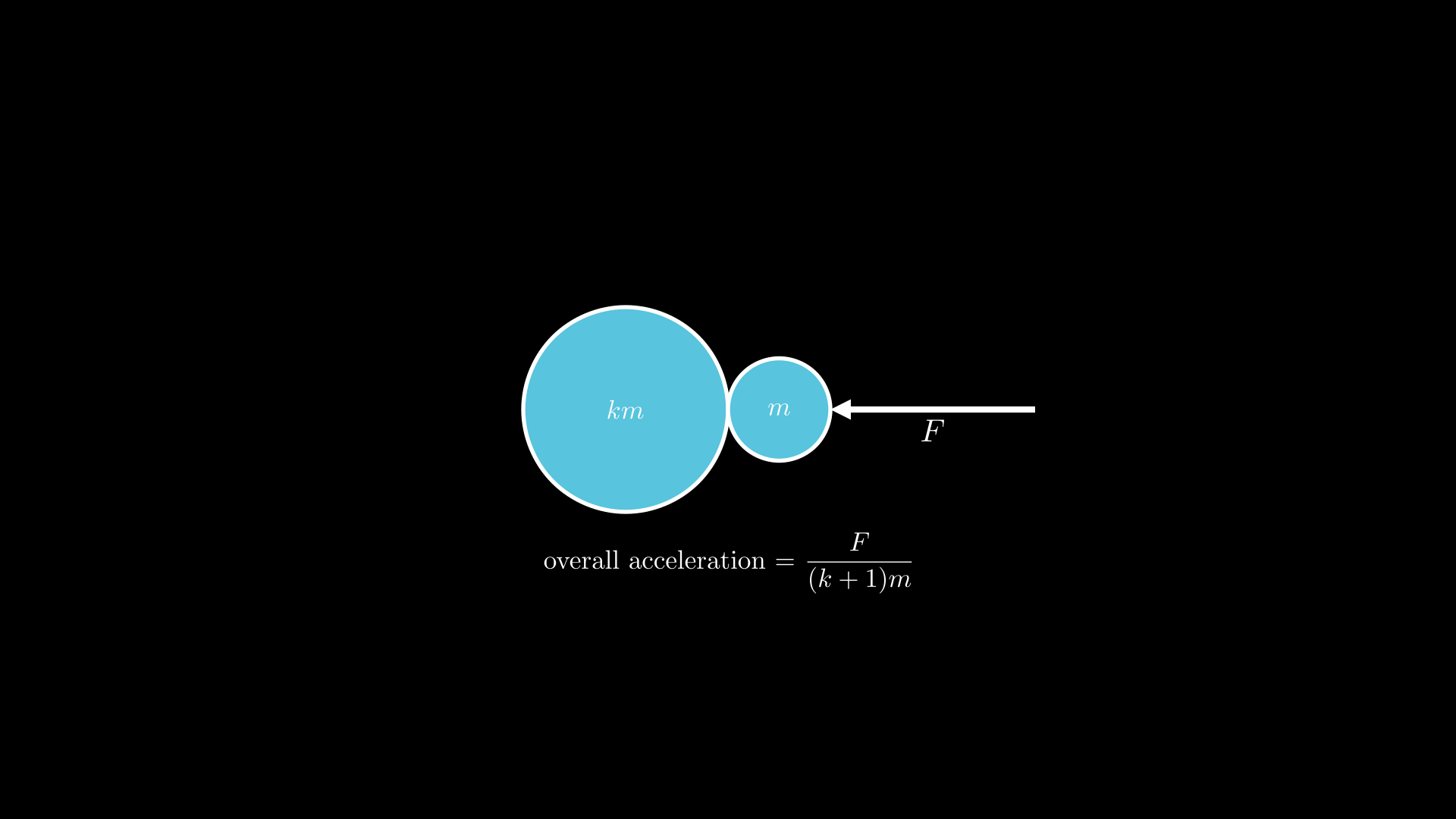
Imaging a fleet of spaceships, initially none of the spaceships accelerates relative to the fleet. Two of the spaceships, let's call them A and B, are connected by a cable, for simplicity we make that cable massless. One of the spaceships starts reeling in the cable.

We know what the result will be: the two spaceships will start moving towards each other. The ratio of the masses of A and B determines the ratio of velocities, in accordance with $F=ma$.

When observed from afar the following three scenarios are indistinguishable:
-Spaceship A is the only craft reeling in the cable.
-Spacechips A and B are both reeling in.
-Spaceship B is the only craft reeling in the cable.

The three scenarios above are indistinguishable in the sense that in all three cases the resulting distribution of acceleration is the same.


In physics there is the following rule of thumb: if some purported distinction cannot be demonstrated with any experiment then you should not allow that distinction to enter your theory. A physics theory should contain only elements that can be experimentally verified.

Example: physics used to have a supposition of a luminiferous Aether. The problem is: in order to make it do what they needed it to do the aetherists had to allow that no experiment can detect this luminiferous Aether. The physics community decided: there is no point in supposition of an entity that inherently cannot be detected. In your theory you should not rely on it in any way.


Back to exertion of force.
Take for example the Coulomb force. When two particles interact, changing each other's momentum, is there any way of setting up an experiment that will tell you whether only one particle is doing the tugging, or whether the two particles are mutually tugging?

In both cases the resulting acceleration distribution is the same, so there is no point in asking the above question; it is not accessible to experiment.


In retrospect it is unfortunate that Newton stated his third law as a property of forces.

Newton formulated his third law as a property of reciprocity, a mutualness. In retrospect we see that things fall into place when we think about it in terms of symmetry. The reciprocity expressed by Newton's third law arises from the uniformity of inertia.

That is why it is worthwhile to assert uniformity of inertia within 'Law of motion 1.'

If you do that then the set of 'Law of motion 1.' and 'Law of motion 2. is sufficient.



It is nowadays quite common for physics textbook authors to associate Newton's third law with the principle of conservation of momentum. When two object interact, changing each other's momentum, the center of mass of the two objects remains in inertial motion.

As we know, the principle of conservation of momentum correlates with a symmetry: in order to formulate a theory of motion we assume inertia is uniform, everywhere.

Improve this answer
$\endgroup$
0
$\begingroup$

This was a fascinating topic for me too, when I was a kid in high school and early college. Yes, you smelled that something is redundant in the three laws. Good job! It's true.

The second law just says/defines what force is. It's the change of momentum of a body in time (or less generally, mass times acceleration):

$F = dp/dt$

It just defines how to call that - we call it force. We don't actually need it, it's just a naming convention. If momentum of a body changes, we say a force acted on it.

If mass doesn't change (and using the definition of momentum $p = mv$, and acceleration $a = dv/dt$), it breaks down into the more commonly known

$F = d(mv)/dt = m \cdot dv/dt = m a$

The true thing behind Newton's laws is the conservation of momentum, and understanding the math behind it.

Law 3 follows easily from the conservation of momentum, and the definition of the force from the 2nd law. Law 1 follows easily out of law 3, if you remove one body.

So, all in all, there's just 1 law here: conservation of momentum.

Let's check how law 3 follows from conservation of momentum. Let's say a system consists of two bodies - their total momentum doesn't change due to conservation:

$d(p_1 + p_2)/dt = 0$

$dp_1/dt + dp_2/dt = 0$

$dp_1/dt = - dp_2/dt$

From the definition of force from the 2nd law and the previous line:

$F_1 = - F_2$

That's it.

Now, we can take the same line and just say the 2nd body didn't exist, and if the mass of the first body doesn't change we have:

$dp_1/dt = - dp_2/dt$

$dp_1/dt = 0$

$dp_1/dt = d(m v_1)/dt = m \cdot dv_1/dt = 0$

If we divide by mass, it disappears from the equation and we get:

$dv_1/dt = 0$

the velocity doesn't change, and so, it's constant:

$v_1 = const.$

If it was 0, it will stay 0 (and the body will stay at rest), if it was not zero, it still won't change (and the body will continue moving with a constant velocity).

Also, the direction doesn't change either because of the same equations. Everything holds even if we were using vectors to indicate direction, from the beginning.

And that's the first law, from the third law.

Even further, the law of conservation of momentum also isn't fundamental, it comes from the homogeneity of space - the symmetry of space in regards to translation and how the laws of physics don't change if a body shifts in space.

This is known from Noether's theorem (along with conservation of energy from the translational symmetry of time; there's other symmetries that give laws of conservation too).

We can go deeper along different paths, but I'll stop there, as it quickly gets increasingly complex.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.