is it possible to find to resistance without knowing the length?
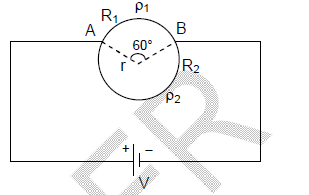
i am tryigng to solve a problem that requires me to find the resistance.I cant seem do so without knowing the length of the circuit which is not given.Is there something that shows resistance without knowing the length or am i just looking at problem all wrong?other details include: resistivity of the upper resistance=4ohm metre,resistivity of the lower resistance=2ohm metre,battery emf V=10pi ohm, radius of the circle=1m,angle between A and B is 60.is there a special relation between resistances in parallel?
$\begingroup$
$\endgroup$
7
-
$\begingroup$ If all you know is the resistivity then you need to know the length because resistance = resitivity * length/area. However this is a somewhat special case. If you edit your question to give more details of the problem, and the ways in which you've attempted to solve it, then I'll have a look. $\endgroup$– John RennieCommented Oct 16, 2013 at 7:46
-
$\begingroup$ other details include: rho of the upper resistance=4ohm metre,resistivity of the lower resistance=2ohm metre,battery emf V=10ohm, radius of the circle=1m,angle between A and B is 60. $\endgroup$– Keith RebelloCommented Oct 16, 2013 at 7:55
-
1$\begingroup$ Here's a hint: what do you know about the relationship between the radius of a circle and its diameter? $\endgroup$– N. VirgoCommented Oct 16, 2013 at 8:15
-
$\begingroup$ Using the arc-sector relationship for a circle. $\endgroup$– LugoCommented Oct 16, 2013 at 8:40
-
$\begingroup$ See physics.stackexchange.com/q/78771 $\endgroup$– John RennieCommented Oct 16, 2013 at 9:27
|
Show 2 more comments
2 Answers
$\begingroup$
$\endgroup$
1
Some good things to remember for basic circuits are that parallel pathways have the effect of increasing the area available to current flow, and because of this always lower resistance -- at least in the basic circuits I know about.
Another thing I remember is adding inverses always amounts to the product over the sum: (1/R1 + 1/R2 + 1/R3 + ...)^-1 = R1R2R3.../R1+R2+R3... } this is a relationship seen in many areas of physics, most notably (imo) for the reduced mass of a system, which can make many calculations much simpler. Since other people have basically already answered your question (the angle reveals the length), I thought I would give my two cents, I hope it moves your studying along a bit faster.
Also, unless I missed something too, I think the lower length should be 5 pi/3. If the path from A to B is a circle, then depending on how you write your fractions, the sum should be 6 pi / 3 or 2 pi. Does this also suggest how, simple as it may be, one of my suggestions might speed things up?
-
$\begingroup$ i tried and i too got 5pi/3 as the lower length.It has helped me. thank you $\endgroup$ Commented Oct 16, 2013 at 11:06
$\begingroup$
$\endgroup$
Hint
Length of the upper half is,
$$\pi /3*r$$
And the length of the lower half is
$$2\pi/3*r$$
$R_1$ and $R_2$ are in parallel.
