Gas and Vapour liquefaction

Question

I am a bit confuse in the first statement.

I think the statement is implying vapour as 'former' and gas as 'latter'. Am I correct here?

But gas can be liqufied with pressure alone below the critical temperature;

and as for vapour, isnt vapour only depend on temperature? Am i wrong here?

So is there a mistake in the statement and by latter it actually means vapour and by former it actually means gas?

Note- I searched liquefaction of vapour but I couldn't find anything regarding it and only found liquefaction of gas and in which,most website call gas as an equivalent term with vapour (which I think is wrong because vapour and gas are different like I dont know how they are different but I think they are different)

Also note- I don't have much clear understanding exactly what is vapour? Is it a transition state between liquid and gas state?

Thank you so much in advance.

Answer 1

Like much JEE/NEET preparation material, the text states somewhat idiosyncratic definitions as universal fact and isn't quite right (and isn't proofread—see "liqufied").

I believe the intended distinction the text aims to make is to define "vapor" as the state of matter with no surface tension and temperature lower than the critical temperature. (The critical temperature is the temperature where the surface tension of the liquid phase drops to zero.) This corresponds to the tan region below, using the phase diagram of water as an example.

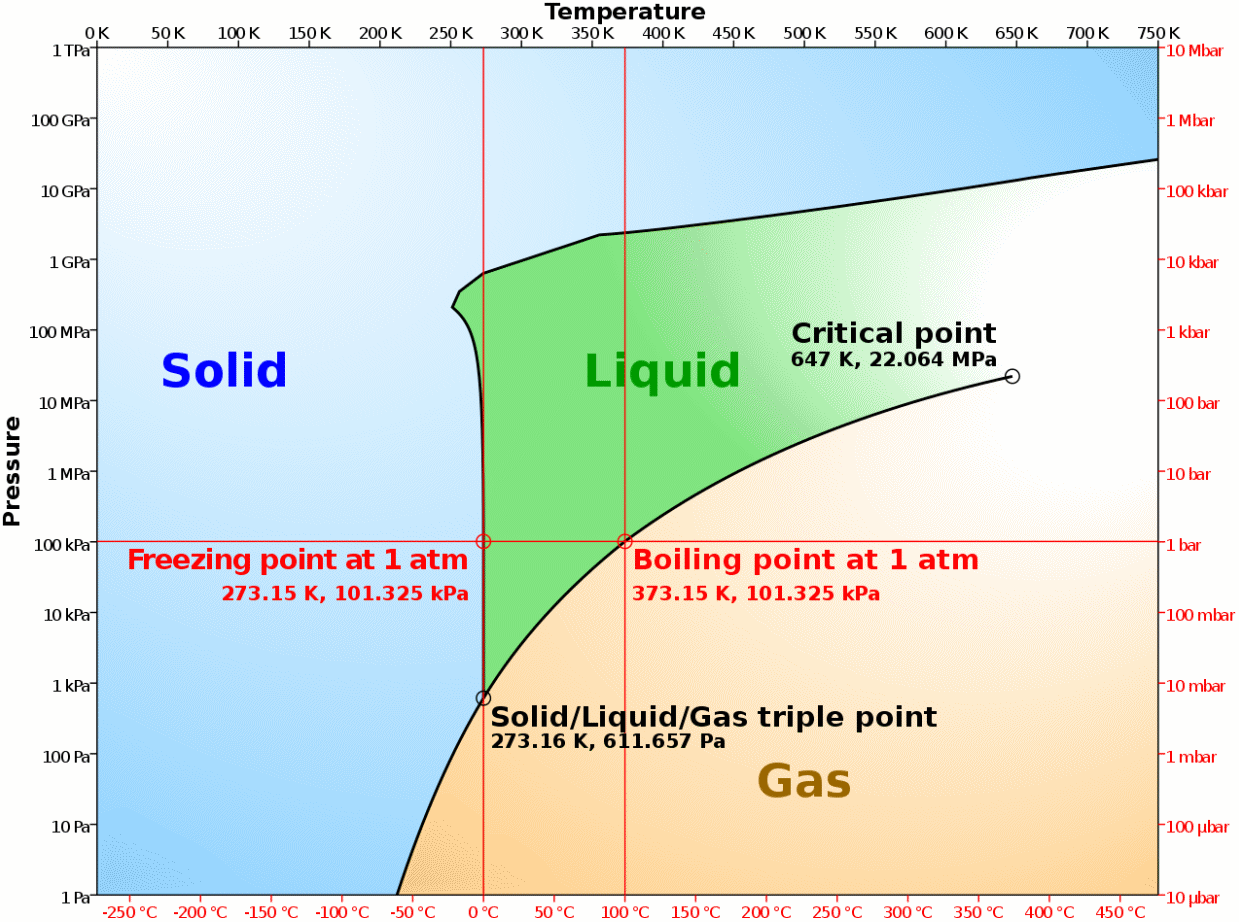
Observe that condensation into the liquid phase—the green region—becomes spontaneous if the vapor pressure is increased to the level of the black separation line.

In contrast, "gas" appears to be defined in the text as the state of matter with no surface tension and temperature above the critical temperature, corresponding to the blended region to the right of the green and tan regions. Here, no line of demarcation can be drawn between the liquid and gas phases because the properties change smoothly (from 0 Pa to about 10 GPa above 647 K, for example). In other words, "gas" can't be condensed into a liquid in well-defined phase transition simply by applying pressure; for that to occur, the gas must first be cooled below the critical point, at which point it's called "vapor," again according to the text.

To repeat, these are somewhat idiosyncratic definitions. Others would define the entire region of no positive surface tension as a gas (subcritical or supercritical, with a supercritical liquid blending into a supercritical gas in terms of material properties), and distinguish vapor specifically as gas in equilibrium with the condensed phase. (So humidity in air would correspond to gaseous water, or alternatively water vapor that at one time evaporated from liquid water or ice.) To imply—as the text does—that vapor isn't gas would elicit quizzical looks from most physicists, I think.

The text is also incorrect that the vapor pressure is independent of the external pressure. The dependence is small but not zero.

Answer 2

I think the statement is implying vapour as 'former' and gas as 'latter'. Am I correct here?

Yes it would appear so, but additional context would be helpful.

Also note- I don't have much clear understanding exactly what is vapour? Is it a transition state between liquid and gas state

Basically yes. But the statement "A vapor differs from a gas" is a bit misleading because saturated vapor (the point where all of the liquid has been converted to a gas at the saturation pressure and temperature) is a gas at the verge of becoming a liquid if any heat is extracted.

Vapor is a mixture of a liquid and gas at its saturation temperature and pressure (boiling point). Any maintained increase in pressure increases the saturation temperature (boiling point), causing all of the vapor to become liquid.

With the exception of saturated vapor, a gas must first be cooled to have its temperature lowered (governed by its specific heat) at a given pressure to reach its boiling point to first become a saturated vapor. The saturated vapor can then begin to be converted to a vapor (mixture of liquid and gas) by withdrawing heat at constant temperature and pressure (governed by the latent heat of vaporization).

Hope this helps.