If we know forces and torques applied to one point of a rigid body, how do we calculate them for another point of a rigid body

Question

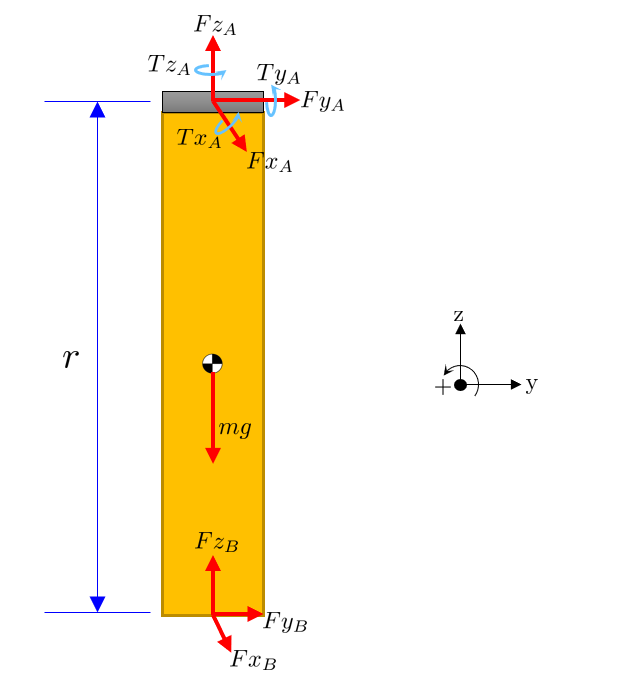
Suppose we have a rigid body with known length $r$ that is fixed at point $A$. We also have a force-torque sensor at point $A$. The body is fixed such that it doesn't move. We apply forces to point $B$ and get the values from sensor at point $A$. How can we translate the f-t values at $A$ to f-t values at $B$?

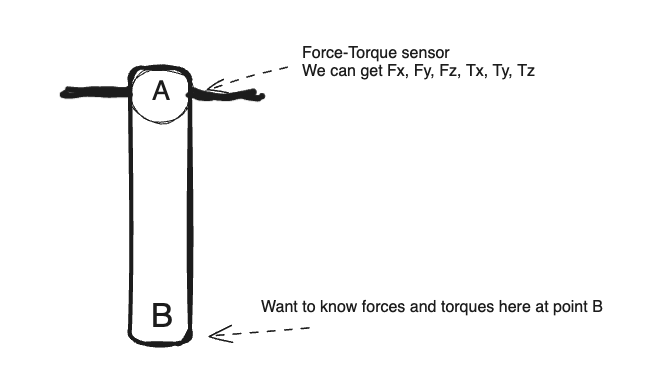
UPD: Decided to write balancing equations to make it a bit more clear:

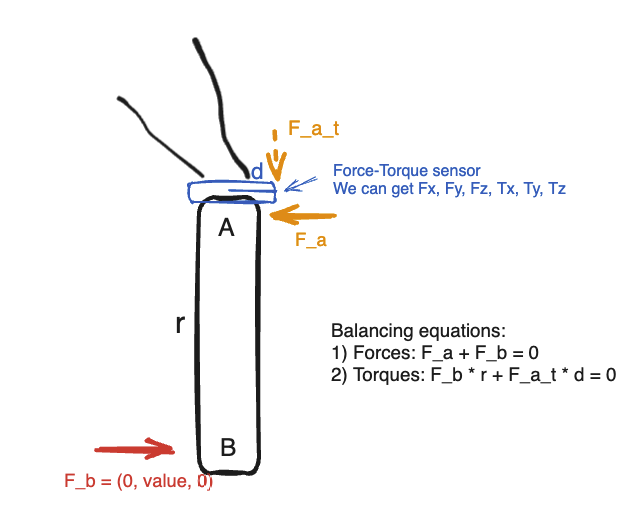
The rigid body is in the equilibrium and we press with some force $F_b$ at point $B$. We can see the values of the f-t sensor at $A$.

Thus:

There is one balancing force at $A$: $F_a$, and one balancing torque, which will be something like $F_{a_{t}}$ $\times$$d$ (depending on the design of the f-t sensor).

$$F_b = -F_a.$$

If $F_a = (0, value, 0)$ then opposing force $F_b$ will be equal to $(0, -value, 0).$

And if we can get values from the force-torque sensor at $B$, then we know the value of $F_a$ at $A$, which is equal to $(0, -F_y, 0)$.

Does this look correct?

Answer 1

Your notation is confusing. Each component needs to indicate both the direction that it corresponds to, and the location it is associated with.

And using the equipollent torque $$ \vec{T}_A = \vec{r}_{\rm B/A} \times \vec{F}_B$$

you have the individual components of force

$$ \vec{F}_A = \pmatrix{ Fx_B \\ Fy_B \\ Fz_B - m g } $$

and torque

$$ \vec{T}_A = \pmatrix{ r\,Fy_B \\ \mbox{-} r\, Fx_B \\ 0 } $$

For the special case where $\vec{F}_B = (0,Fy_B,0)$ then $Tx_A = r\,Fy_B$ is the only surviving term.

Note that the weight of the part does not impart a torque on the support because the line of action of gravity goes through the support.