Fundamental principles for simple radiative heat transfer problems

Question

The picture below illustrates what is intended to be a very simple one-dimensional textbook-style radiative heat transfer problem. It is meant to be a pedagogical tool for explaining the greenhouse effect. The idea is that the gray plate is transparent to all of the incoming radiation from the distant hot source but is otherwise well-approximated as a blackbody. The hot source is at such a distance that it delivers 240 W/m$^2$ to the top surface of the brown plate which is black, $\epsilon = 1$. The bottom surface of the brown plate is perfectly insulated such that no energy escapes through that side. The plates are intended to be parallel and closely spaced such that radiation out from the edges can be neglected, i.e. large in comparison to the spacing between them but still small in comparison to the distance from the source. All objects are surrounded by vacuum at zero Kelvin.

Without the gray plate in place the problem solution for the temperature of the brown plate is 255 K since that is the temperature where it emits 240 W/m$^2$, and the heat in is balanced by the heat out. However, the argument put forth is, since the gray plate is never going to be at a higher temperature than the brown plate it does not transfer any heat to the brown plate and thus cannot raise the temperature of the brown plate.

Is this correct?

Answer 1

Incropera and DeWitt's Fundamentals of Heat and Mass Transfer is a useful resource for radiative and other heat transfer problems.

The total hemispherical emissivity of the bottom (black) plate is $\varepsilon_\mathrm{black}=1$.

The total hemispherical emissivity of the top (gray) plate is $\varepsilon_\mathrm{gray}$, where

$$\varepsilon_\mathrm{gray}=\int_{\lambda_0T_\mathrm{gray}}^\infty \frac{2\pi hc^2}{\sigma T^5\lambda^5\left[\exp(hc/\lambda k_\mathrm{B}T-1\right]}d(\lambda T)$$

with threshold absorption wavelength $\lambda_0$ (here, 1 µm), gray plate temperature $T_\mathrm{gray}$, Planck's constant $h$, speed of light $c$, Stefan–Boltzmann constant $\sigma$, temperature $T$, wavelength $\lambda$, and Boltzmann's constant $k_\mathrm{B}$. This is $1-F_{0\to\lambda_0 T_\mathrm{gray}}$, where $F$ is the so-called blackbody radiation function, a tabulated value dependent on $\lambda_0 T_\mathrm{gray}$ ($\lambda T$ in general) that captures areas under the Planck distribution of spectral intensity.

At steady state, the net heat transfer (which is assumed here to be entirely radiative) for each plate is zero.

For the bottom (black) plate, the total input power (in W/m²) corresponds to the source's $P_\mathrm{source}=240\,\mathrm{W}\,\mathrm{m}^{-2}$ and $\varepsilon_\mathrm{gray}\sigma T_\mathrm{gray}^4$ from the upper (gray) plate. The total output power is $\varepsilon_\mathrm{black}\sigma T_\mathrm{black}^4=\sigma T_\mathrm{black}^4$.

For the upper (gray) plate, the total input power is $\varepsilon_\mathrm{gray}\sigma T_\mathrm{black}^4$ from the bottom (black) plate. (That is, the impinging blackbody radiation from the black plate is modulated by the gray plate's absorptivity, which equals its emissivity.) The total output power (both sides) is $2\varepsilon_\mathrm{gray}\sigma T_\mathrm{gray}^4$.

So the two equations in two unknowns that need to be solved are

$$240\,\mathrm{W}\,\mathrm{m}^{-2}+\varepsilon_\mathrm{gray}\sigma T_\mathrm{gray}^4=\sigma T_\mathrm{black}^4;$$

$$ T_\mathrm{black}^4=2 T_\mathrm{gray}^4.$$

The solution is $T_\mathrm{gray}=255\,\mathrm{K}$, $T_\mathrm{black}=303\,\mathrm{K}$. ($F_{0\to\lambda_0 T_\mathrm{gray}}$ ends up being essentially 0 because the upper (gray) plate isn't hot enough to radiate substantially at shorter wavelengths; thus, we're essentially just solving $\frac{240\,\mathrm{W}\,\mathrm{m}^{-2}}{\sigma}= T_\mathrm{gray}^4$.)

Answer 2

The first thing to note is that the properties of the gray plate are such that it is for all intents and purposes a blackbody with respect to its absorption from the brown plate and its emission to space, but it is completely transparent to the radiation from the hot source. For example for a temperature of 300K a blackbody emits $\sigma (300^4) = 459.3 W/m^2$, while the gray plate emits $\sigma (300^4) - 1.2 \times 10^{-14} W/m^2$ when it is at 300K, where the small number being subtracted is the amount of radiation a blackbody emits in the wavelengths from 0 to 1 $\mu m$.

Next, in order to track all of the heat inflows and outflows to the plates, let’s make the lumped thermal capacity approximation for the plates and assume that they are each at a uniform temperature. Let’s also write everything as per unit area of the plates. The rate form for the first law of thermodynamics (under the assumption of local thermodynamic equilibrium) is,

$$\frac{dU}{dt}=\dot Q + \dot W$$

where $U$ is the internal energy, $\dot Q$ is the net rate of heat inflow to the system $\dot Q=\dot Q_{in}-\dot Q_{out}$, and $\dot W$ is the rate that work is done by the surroundings on the system. For this problem it is clear that $\dot W=0$.

The lumped thermal capacitance approximation then uses,

$$\frac{dU}{dt} = C \frac{dT}{dt}$$

where $C$ is the total thermal capacity of the plate, which is just the mass per unit area of the plate times its specific heat.

The heat influx to the brown plate per unit area is simply the heat influx from the hot distant source $\dot Q_{in} = Q_0=240 W/m^2$, and the heat outflux per unit area is given by the Stefan-Boltzmann equation for the radiative heat flux between two closely spaced parallel plates, $\dot{Q}_{out} = \sigma (T_{b}^{4}-T_{g}^{4})$ where $b$ is for the brown plate and $g$ is for the gray plate. This allows us to write a governing equation for the temperature evolution of the brown plate as,

$$C_b \frac{dT_b}{dt} = 240 - \sigma (T_{b}^{4}-T_{g}^{4})$$

For the gray plate the heat influx is simply the heat outflux from the brown plate, $\dot{Q}_{in} = \sigma (T_{b}^{4}-T_{g}^{4})$, and the heat outflux is the radiation emitted to space, $\dot{Q}_{out} = \sigma T_{g}^{4}$. This allows us to write a governing equation for the temperature evolution of the gray plate as,

$$C_g \frac{dT_g}{dt} = \sigma (T_{b}^{4}-T_{g}^{4})-\sigma T_{g}^{4} =\sigma (T_{b}^{4}-2 T_{g}^{4}) $$

These equations can be cast in non-dimensional form with the following definitions,

$$T_0 =(Q_0/\sigma)^{1/4}, \bar{T} = \frac{T}{T_0},t_0 = \frac{C_b}{\sigma T_0^{3}}\text{, and } \bar{t} = \frac{t}{t_0}$$

The equations are then,

$$\frac{d\bar{T_b}}{d\bar{t}} = 1 - \bar{T}_{b}^{4}+\bar{T}_{g}^{4}$$

and

$$\frac{C_g}{C_b} \frac{d\bar{T_g}}{d\bar{t}} = \bar{T}_{b}^{4}-2 \bar{T}_{g}^{4} $$

This set of two ordinary differential equations for $\bar{T_b}$ and $\bar{T_g}$ have a single parameter $C_g/C_b$ that must be specified along with initial temperatures for each plate. Let’s consider the case where the gray plate is gone and the brown plate has reached its steady state temperature of $\bar{T_b}=1$, and at time $\bar{t}=0$ the gray plate is introduced at a temperature of $\bar{T_g}=0$. Let’s also take $C_g/C_b = 1$. For this case the time evolution of the temperatures of the brown and gray plates is shown below. Note that the steady state temperatures for the plates are $T_b=2^{1/4} T_0$ and $T_g = T_0$.

So how is it that the brown plate increases in temperature if the gray plate is always colder and there is never any heat flux from the gray to the brown plate?

The answer lies in the fact that the net heat outflux from the brown plate changes upon the introduction of the gray plate. Prior to the introduction of the gray pate the brown plate was emitting radiation to 0K space, which is the maximum amount of heat that it can lose per second via radiation. After the gray plate is introduced it warms due to the heat it receives from the brown plate and thus the brown plate is losing less heat per second than when it was when it was emitting to space. Since it is still receiving 240 $W/m^2$ from the source every second it must warm up until it is transferring 240 $W/m^2$ to the gray plate. In turn, the gray plate must emit 240 $W/m^2$ to space at steady state and will be at 255K to do so.

The graph below illustrates this behavior showing the heat influx to the brown plate from the source and the heat outflux to the gray plate. The net is of course positive during the transient, which causes the temperature of the brown plate to increase until the new steady state is achieved. This is in spite of the fact that no heat is ever transferred from the colder gray plate to the hotter brown plate.

Answer 3

Look Squirrel: Just substitute some numbers. If the rate of energy input and output is (a = 240 W/m^2) w/o the intermediate plate & assuming radiation the only method of energy transfer the temperature of the brown/lower plate is 255K.

If the second plate is introduced and intercepts all the energy radiated from the bottom the amt radiated from the bottom one has to double. The temp of the bottom plate is then

Tb = 2^(1/4)*(255K) = 303K

Answer 4

This is actually a fairly simple problem that can be stripped down to make the point.

The rate at which energy is transferred from the external source to the bottom plate is a and the bottom plate transfers energy to the top one at a rate of b

That has to equal the energy emitted to the outside (space) from the top plate, call that c which is also (by symmetry) the rate at which the top plate sends energy to the bottom one

Now by conservation of energy a = c

and a + c = b or by substitution b = 2a

If you only had one plate, for that situation b = a Thus, the bottom plate has to get rid of energy at twice the rate once you introduce the top one (convection, sensible heat, radiation, mb even a little conduction)

Thus the green plate effect.

ADDITION: Since Eli as a newbie does not have 50 reputation points comments are not yet allowed, but editing is, so this is going to be a bit long

For those who wanted more detail

Just substitute some numbers. If the rate of energy input and output is (a = 240 W/m^2) w/o the intermediate plate & assuming radiation the only method of energy transfer the temperature of the brown/lower plate is 255K.

If the second plate is introduced and intercepts all the energy radiated from the bottom the amount radiated from the bottom one has to double. The temp of the bottom plate is then

Tb = 2^(1/4)*(255K) = 303K

The key physics here is that the top plate has to emit the same amount of thermal energy/heat to space as is absorbed by the bottom plate.

To do the problem numerically you have to specify two things

First: how thermal energy is transferred to outside the system, here Eli assumes that it is emission of radiation into a vacuum. That sets the temperature of the top plate as 255K

Second: the nature of the transfer of thermal energy between top and bottom plates. That will set the temperature of the bottom plate. The simplest thing is to assume the space between the plates is a vacuum and the mechanism is again radiation. That means (using the solution above) that the temperature of the bottom plate has to be 303K.

In short to maintain the equilibrium the bottom plate's temperature increases upon the introduction of the second plate. As Clausius said

"The principle assumed by the author as the ground of the second main principle, viz. that heat cannot of itself, or without compensation, pass from a colder to a hotter body, corresponds to everyday experience in certain very simple cases of the exchange of heat. To this class belongs the conduction of heat, which always takes place in such a way that heat passes from hotter bodies or parts of bodies to colder bodies or parts of bodies. Again, as regards the ordinary radiation of heat, it is of course well known that not only do hot bodies radiate to cold, but also cold bodies conversely to hot; nevertheless the general result of this simultaneous double exchange of heat always consists, as is established by experience, in an increase of the heat in the colder body at the expense of the hotter."

In this case 480 W/m^2 goes from the hotter bottom plate up to the cooler top plate and 240 W/m^2 goes in the other direction, so the net is 240 W/m^2 going from the hotter to the colder and the second law is obeyed

Answer 5

The problem is all about the conservation of energy. In steady state, heat flow into and out of any subset of the system has to balance.

The bottom plate has 240 W/m$^2$ going in and only 120 W/m$^2$ coming out, so by the conservation of energy it can't be in a steady state. It's going to warm up, until the output equals the input.

The top plate has 120 W/m$^2$ going in and 120 W/m$^2$ coming out, so on the face of it that would be OK, but when we have corrected the other problems it won't be.

Thirdly, there is radiation being emitted upwards from the top plate, but not downwards. The problem description says nothing about it having a reflective bottom, as it does for the lower plate.

Fourthly, I note the two plates are at different temperatures, but emitting the same 120 W/m$^2$ black body radiation. That's inconsistent.

It's not relevant to answering your question, but I would seriously question the pedagogy here. This is NOT how a greenhouse works; neither the one in the buildings made of glass, nor the famous one in the atmosphere. If you use a transparent pane of rock salt (which is transparent to IR) instead of glass on your greenhouse, you get virtually the same rise in temperature, because greenhouses work by suppressing convection, not by re-emitting IR. And the one in the atmosphere works by raising the average altitude of IR emission to space combined with the adiabatic lapse rate between that altitude and the surface. The altitude radiating to space equilibrates at a temperature that matches input to output. The adiabatic lapse rate is caused by the temperature changes from the compression/expansion of gases as they rise/fall in the atmosphere.

It's fine as an abstract problem in radiative thermodynamics, but connecting it to the greenhouse effect is only going to lead to confusion and dispute when the gaping holes in the theory are pointed out. (Principally, that the atmosphere is obviously not a vacuum at 0K. It convects, preventing the build up of too great a temperature gradient. It's the same reason the oceans don't boil, despite liquid water also being transparent to visible light and opaque to IR.)

Answer 6

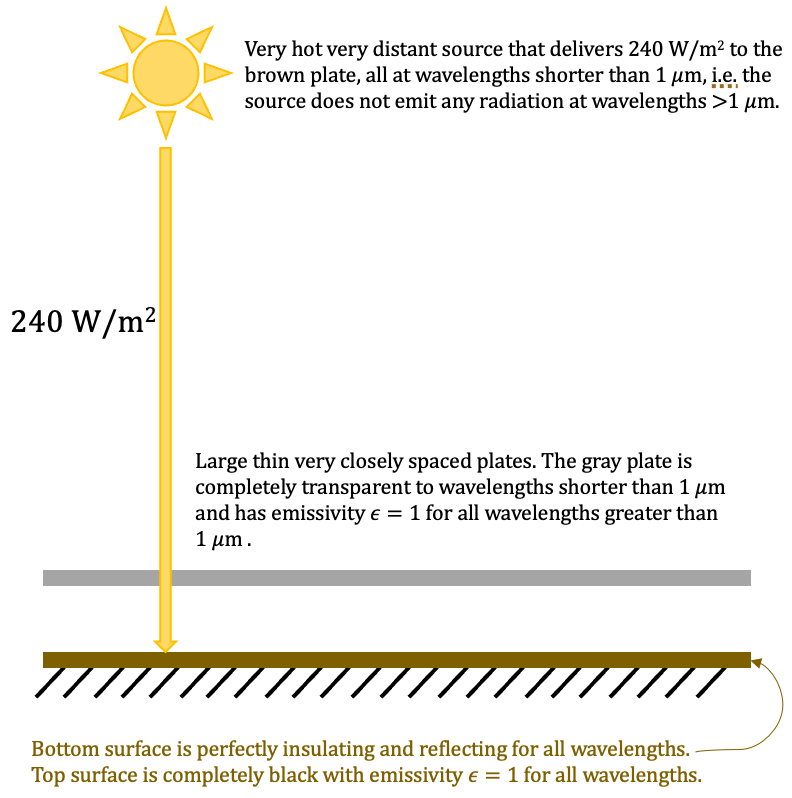
To answer this question we must of course utilize the laws of thermodynamics. It also deals with radiation and so the plane-parallel nature of the problem makes the math more tractable.

First Law:

$dU = Q + W = m*Cp*dT$ (etc.)

There is no work involved, and so we can simplify to:

$dU = Q = m*Cp*dT$

Thus, the first law states that to increase a body's temperature (+dT), one requires heat (Q), and this is an increase in a body's internal energy (+dU).

It is important to note that the the First Law and dU corresponds to a specific body, and this is consistent with the equation for heat, Q, for this plane-parallel scenario as a general equation:

$Q_{hot->cool} = σ(T_{hot}^4 - T_{cool}^4)$

Q is the heat which the specific body receives, and so the specific body is usually the 2nd term in the above equation. Then, in relation to the first term in the heat equation, if the result for Q is positive, then it means that the specific body of the 2nd term is receiving heat; otherwise if Q is negative then it means the 2nd body term is not being heated, which is to say, it is not having its temperature raised, by the body of the first term, which implies that it must be the 2nd term which in fact has higher temperature. We should keep in mind that heat is a transient action, much like work is a transient action, and they are both in general something which one body performs upon another.

Initially, the heat equation for the bottom surface would look like:

$Q_{Sun->bottom} = F_{Sun} - σT_{bottom}^4$

Thermal equilibrium for a given body is established when that body has no change in internal energy (dU = 0), which means that its temperature doesn't change via the First Law (dT = 0). Thus, if we are seeking when dU = dT = 0, then via the First Law for this scenario also requires Q = 0.

$dU = Q_{Sun->bottom} = F_{Sun} - σT_{bottom}^4 = 0$

And then the bottom surface emits the same energy as the input

$F_{Sun} = 240W/m^2 = σT_{bottom}^4$

so that

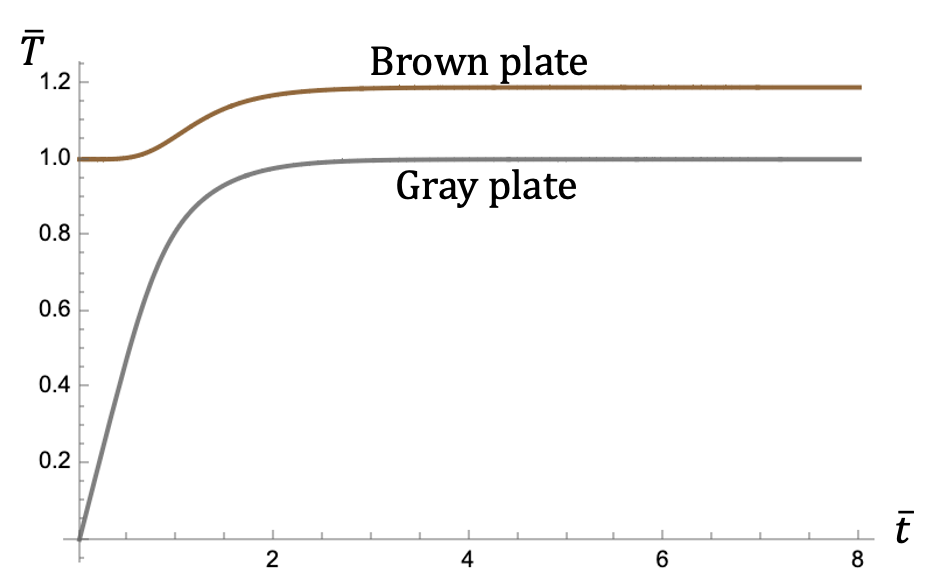
$T_{bottom} = 255K$ (approx)

The question is: what happens with this second plate involved?

The first thing about that is that the Sun does not interact with it, and so there's no heat from the Sun for it.

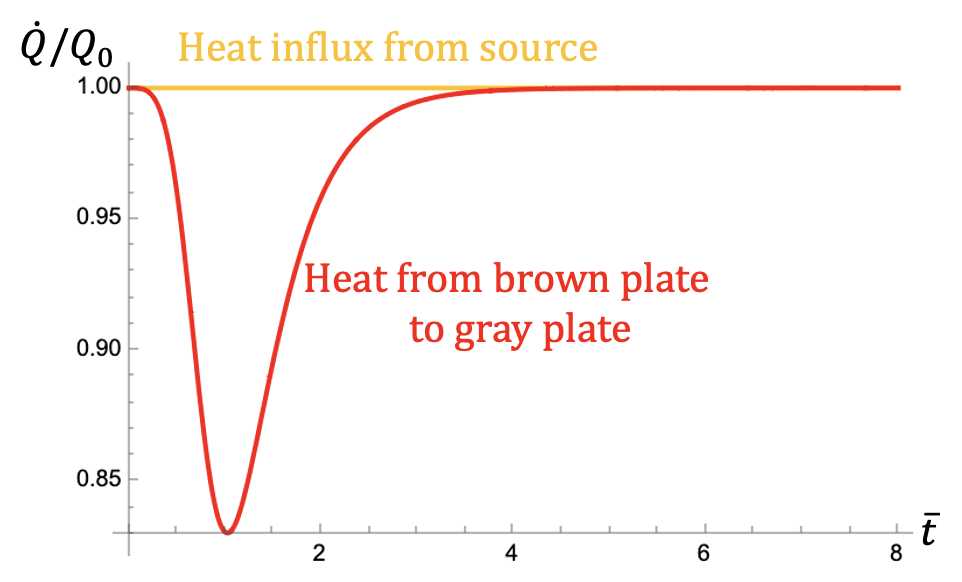
Second, there is definitely heat from the bottom surface to the second (grey) plate, and in general the equation is going to be:

$Q_{bottom->grey} = σ(T_{bottom}^4 - T_{grey}^4)$

Since heat first acts on the bottom plate, or, if we considered that the grey plate is inserted after the bottom plate came to equilibrium, either way, the bottom plate always has a higher initial temperature than the grey plate. Therefore, Q is always from the bottom plate acting on the grey plate.

With thermal equilibrium defined as Q = 0, then the above equation results in thermal equilibrium where

$T_{bottom} = T_{grey}$

which is 255K.

Of course, some solutions suggest that the radiation from the grey plate must add with the radiation from the Sun and act as additional heating forcing on the bottom plate. Again, it is extremely important to understand that the First Law applies to a given body, not a system of bodies, and likewise, the heat equations apply to pairs of bodies, not systems of bodies. If it needs further explanation to understand, consider temperature, and that fact that temperature is part of the first law, as we saw in the first equation. The point here being that temperature is an intrinsic, in-situ property of A BODY, i.e., an object, not a system of spatially-separated objects; in fact one could even say, given that heat is a transient phenomenon which acts at the surface of a body, that for all intents and purposes, temperature is measured at the surface of a body, and this makes sense whether you measure temperature at the surface via radiative means, or, with a thermometer stuck inside a body (there is a new surface created between the thermometer and the body being measured - the interface between the body and the thermometer).

And so, sure, if you proceed with summing all energies, thinking that the solar energy can be summed with the energy from the grey plate, then indeed you would think that the bottom plate should go higher in temperature.

However, the First Law is about directing us to the correct way to solve this problem, and it is really does not tell us to sum all energy, but instead, to consider a body by itself and then the heat exchange it has with each other body it forms pairs with independently, not in sum. The reason you have to do this is because of view factors.