2
$\begingroup$

I think my confusion stems from this: if a book is resting on a table I understand that the force of gravity acts on the book and as it is in equilibrium, the table exerts a force equal in magnitude on the book so that there is no net force. Is it correct to say there is an electrostatic force that the book applies on the table equal to its weight that the table then exerts back, but why would it be equal to its weight?

So now, if a car is accelerating so has a force acting on it, if it were to collide with a wall a force would be exerted on the wall and on the car, but where does it come from - is it the same as the book on the table?

Improve this question
$\endgroup$
8
  • $\begingroup$ You should be careful about where the conclusions come from. Newton's Second Law, $\sum \vec{F} = m \vec{a}$, is about the sum of forces on one object. Newton's Third Law, $\vec{F}_\text{A on B} = - \vec{F}_\text{B on A}$ is about forces on two different objects (a FBD, of forces on one object, will never contain a N-III pair). For the book: gravity acts downward (because it has mass); normal acts upward (because of contact); assuming $a=0$ yields $F_g = F_N$. Note that N-III was not involved here. The book also exerts an NIII paired $F_N$ on the table, but that's a force on the table. $\endgroup$
    – Ben H
    Commented Mar 16 at 11:32
  • $\begingroup$ But why is the force exerted by the book on the table NII paired not NIII, if the table exerts an equal and opposite force on the book? $\endgroup$
    – lemonmeringue
    Commented Mar 16 at 11:36
  • 1
    $\begingroup$ I had a typo in my comment, it is a N-III pair: $\vec{F}_\text{$N$, table on book} = - \vec{F}_\text{$N$, book on table}$. These pairs will always be of the same type of force. I was saying be careful because, for example, your statement that "force that the book applies on the table [is] equal to its weight" is a combination of three conclusions: $\sum \vec{F}_\text{on book} = m \vec{a} = 0 \; \rightarrow \; F_g = F_N$; $\vec{F}_\text{$N$, table on book} = - \vec{F}_\text{$N$, book on table} \; \rightarrow \; F_\text{$N$, ToB} = F_\text{$N$, BoT}$; and therefore $F_\text{$N$, BoT} = F_g$. $\endgroup$
    – Ben H
    Commented Mar 16 at 11:42
  • $\begingroup$ I see, so then in other interactions involving movement like pushing a small box with your hand, the force from the box on the hand is not equal to the force acting on the hand making it accelerate, but will be equal to the force the hand exerts? And so the box moves and the hand keeps moving? $\endgroup$
    – lemonmeringue
    Commented Mar 16 at 11:50
  • $\begingroup$ Almost. Let's completely separate the use of Newton II from Newton III. When pushing a box, there are forces on the box: $\vec{F}_\text{$N$, hand on box}$, $\vec{F}_g$, $\vec{F}_\text{$N$, ground on box}$, $\vec{F}_\text{$fk$, ground on box}$. Note that all of these forces are "on box". Newton's Second Law says that their (vector) sum is equal to whatever the acceleration of the box is, times its mass. Separately, the force of the "box on the hand", is the pair to the first of those forces. It is a normal force equal to the normal force of the hand on the box. So, they are equal. $\endgroup$
    – Ben H
    Commented Mar 16 at 11:55

2 Answers 2

Reset to default
1
$\begingroup$

Electrostatic sounds good. The table (wall) has atomic orbitals on its surface atoms, as does the book (car). So when they come in contact, the electrons are now trying to occupy the same space.

Now I have heard this attributed the Pauli Exclusion Principle....by PhDs on YouTube even, but I don't think that's right. If it were, the repulsion would begin when the density approaches electron-degenerate matter, e.g., white dwarf density, and that is clearly not happening.

So...I'd go with the orbitals bump up against each other, and the change in shape increases their energy, which makes a repulsive force. Push hard enough a materials break (which I presume is a well studied phenomenon).

I don't know how much the orbitals are affected, but just remember: electricity is 30+ orders of magnitude stronger than gravity. A gram (1 mole) of protons in the core of the Sun--even at Earth densities-- has enough electrostatic potential energy to graviationally unbind the whole star.

Improve this answer
$\endgroup$
1
  • 1
    $\begingroup$ Electron degeneracy in white dwarf matter pushes electrons up to near relativistic energies, but is present in ordinary condensed matter. "the orbitals bump up against each other" is a way of describing the Pauli force. $\endgroup$
    – John Doty
    Commented Mar 16 at 12:45
1
$\begingroup$

I think my confusion stems from this: if a book is resting on a table I understand that the force of gravity acts on the book and as it is in equilibrium, the table exerts a force equal in magnitude on the book so that there is no net force.

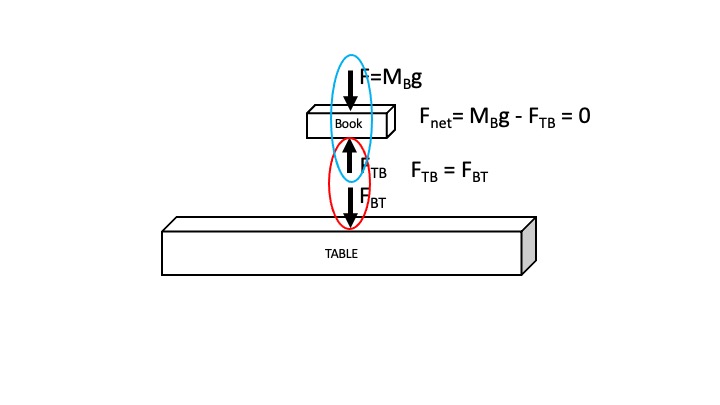
That is correct. The relevant pair of forces are circled in blue in the figure below.

Is it correct to say there is an electrostatic force that the book applies on the table equal to its weight that the table then exerts back, but why would it be equal to its weight?

The contact forces between the table and book are circled in red in the figure below. The force the table exerts on the book is equal and opposite to the force the book exerts on the table, per Newton's 3rd law. What keeps the force of gravity from pushing the book through the table are the repulsive electrostatic forces between the molecules of the table and book. The mechanical analog is to imagine the molecules interconnected with one another by springs that get compressed due to force the book exerts on the table and the table exerts on the book. Overall the compression of the springs (deformation of the book and table) is so small as not to be visible to the eye.

So now, if a car is accelerating so has a force acting on it, if it were to collide with a wall a force would be exerted on the wall and on the car, but where does it come from - is it the same as the book on the table?

The force on the car comes from the rate of change in its momentum brought about by the wall bringing it to a stop. The net force on an car equals its rate of change in momentum, or

$$\vec F_{net}=\frac{d\vec p}{dt}=m\frac{d\vec v}{dt}$$

The force exerted on the wall by the car is equal and opposite to the force the wall exerts on the car, per Newton's 3rd law. The nature of the forces is different from the interaction between the book and table as energy is dissipated in permanently deforming the materials of the car and/or wall in the form of heat and sound.

Hope this helps.

enter image description here

Improve this answer
$\endgroup$
4
  • $\begingroup$ I've heard that we don't really touch when we touch. Is that true? $\endgroup$
    – PinkAura
    Commented Mar 16 at 18:57
  • $\begingroup$ @PinkAura How do you define "touch"? $\endgroup$
    – Bob D
    Commented Mar 16 at 19:04
  • $\begingroup$ I meant there remains a gap between the finger and the surface due to electrostatic force? I might be wrong... this might be off topic, just confirming $\endgroup$
    – PinkAura
    Commented Mar 16 at 19:07
  • 1
    $\begingroup$ @PinkAura You might find this interesting: wtamu.edu/~cbaird/sq/2013/04/16/…. $\endgroup$
    – Bob D
    Commented Mar 17 at 21:08

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.