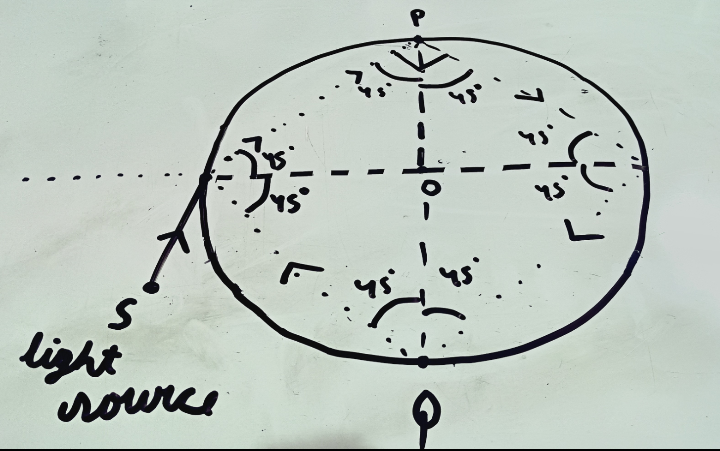
Light is passed through a circular prism in the form of a disc (by refraction) in a way so that the the angle made by the refracted ray with the normal is 45 degrees.Assuming that the prism is of glass and the surrounding medium is air and the critical angle for the glass air interface is less than 45 degrees, the light ray suffers total internal reflection inside the prism. If total internal reflection doesn't involve the loss of light energy, does the light get trapped in the prism?
-
5$\begingroup$ If it can enter, then it will be able to exit when it gets to a similar point the other way. $\endgroup$– naturallyInconsistentCommented Mar 6 at 5:56
-
3$\begingroup$ I'm trying to tell you that if you have a pretty symmetric situation like this ellipse, then if it can enter by refraction, then it can also exit by refraction. $\endgroup$– naturallyInconsistentCommented Mar 6 at 8:54
-
1$\begingroup$ Look at your Snell's Law. If it can refract into the thing and move at 45 degrees, then it must also be able to leave at 45 degrees. $\endgroup$– naturallyInconsistentCommented Mar 6 at 9:23
-
2$\begingroup$ Yes, if you considered Snell's Law in enough detail, you will realise that either the light can enter and leave at those angles, or it will neither enter nor leave at those angles. You cannot create a scenario whereby the light gets trapped like how you think it can. $\endgroup$– naturallyInconsistentCommented Mar 6 at 11:41
-
2$\begingroup$ Optics are entirely reversible. The "one-way mirror" does not exist. If a ray can enter at some angle, then by simply reversing the direciton, is can also exit by that same angle. $\endgroup$– MichaelKCommented Mar 6 at 12:07
4 Answers
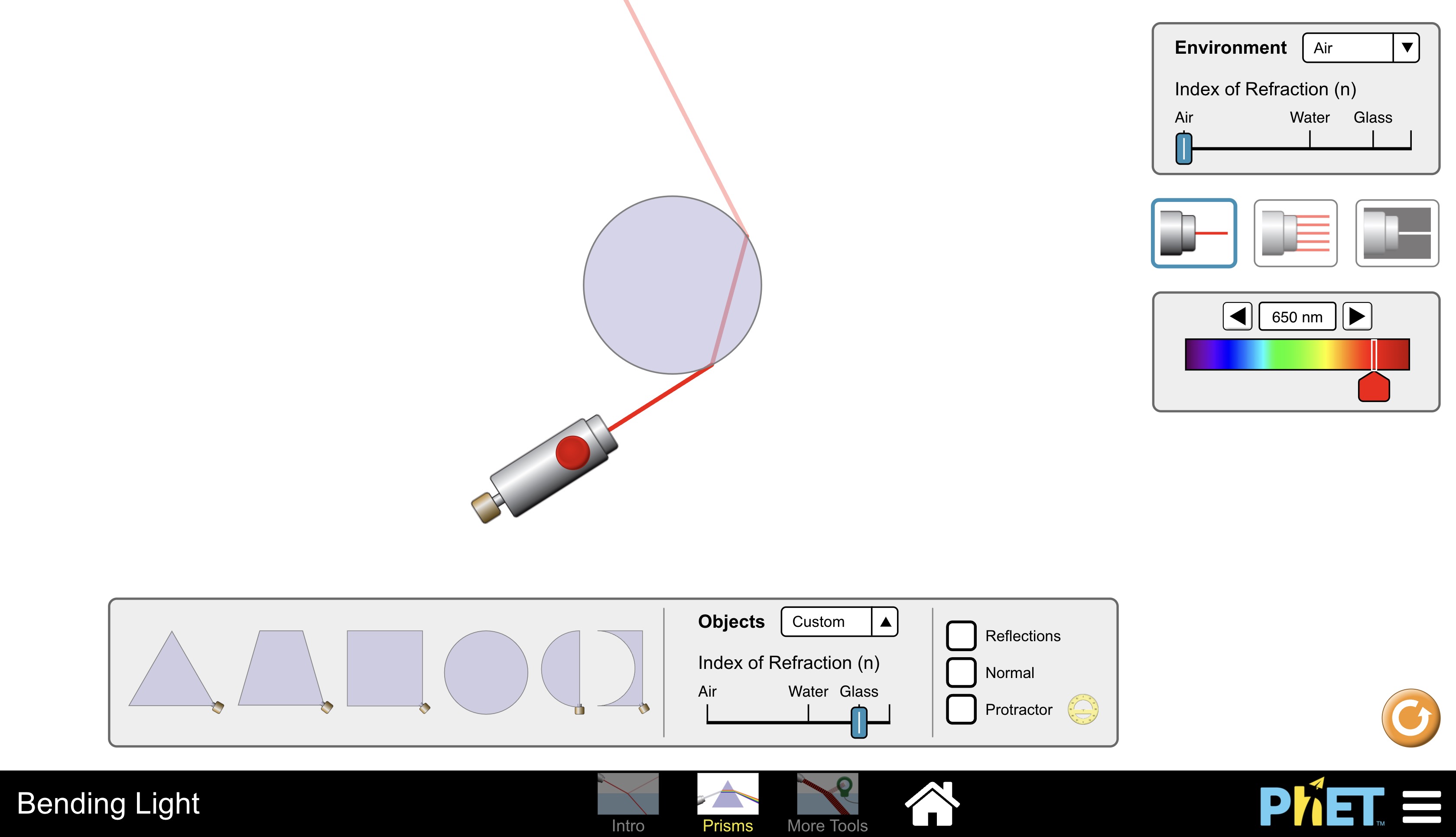
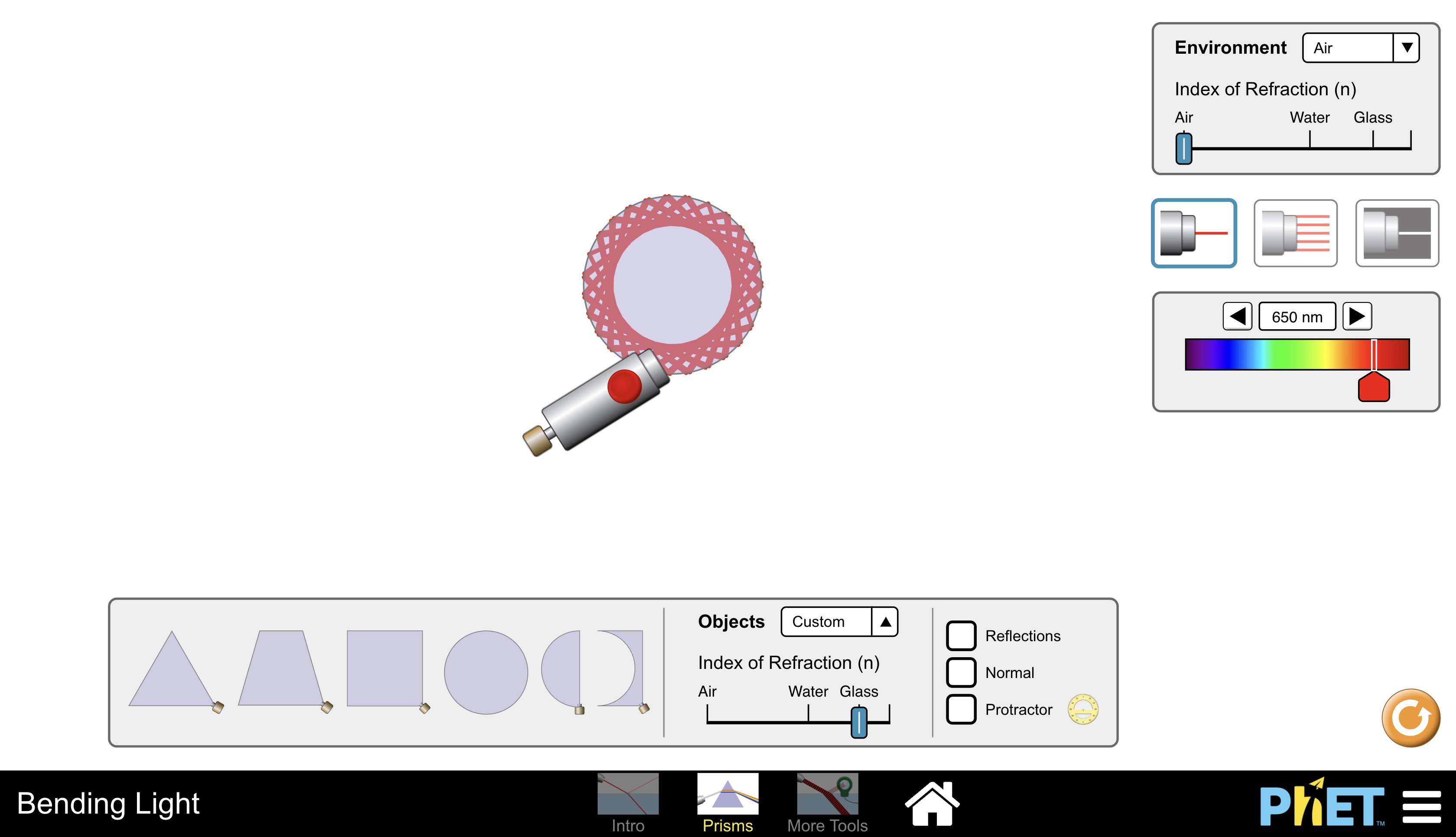
Heading over to PhET's Bending Light Simulator, you can try out this experiment yourself. As it turns out, you cannot get the light to be infinitely contained in the glass if you shine light from the outside. But it is possible if the light source is within the glass, as is shown below.
But why is this true?
To answer this question, let us first try to find out the necessary conditions for the light to have an angle of incidence equal to the critical angle at its second incidence (when it goes from glass to air).
[I’ll be using “the first incidence of light” to refer to when the light goes from the air to the glass, and “the second incidence of light” to refer to when it goes from the glass to the air.]
Have a look at the following image.
First you must understand that the normals drawn at points B and C have the radii of the circle as their part, i.e., if extended, they intersect at the centre always. Why is this true? Because the normals are by definition perpendicular to the tangents to the circular glass drawn at those points — B and C. But it is known that the radius of a circle is the line segment that is perpendicular to a tangent drawn at any point on the circumference. Therefore, the normals must be extensions of the radii of the circle.
If you didn’t get the above part, you may ignore it; just know that OB and OC are radii of the circle, hence they are equal. This would imply that triangle BOC is isosceles, and so ∠OBC = ∠OCB.
Now we know by Snell’s law that $\sin{i} \propto \sin{r}$. In other words, more the angle of incidence, more the angle of refraction, and vice versa, because the sine function increases from $0°$ to $90°$, and those are the only angles we are considering here. Thus, if we look at the first incidence, to maximise $r_{1}$ (∠OBC), we need to maximise $i_{1}$ (∠EBA).
But $i_{1}$ cannot be any bigger than $90°$, because if it were greater, the light would basically have its source within the glass, and we know that the light does get infinitely contained if its source is in the glass.
Now what is the angle of refraction $r_{1}$ corresponding to a $90°$ $i_{1}$? Let’s find that out using Snell’s law! Assuming $1.5$ to be the refractive index ($\mu$) of glass,
\begin{align} & \frac{sin(i)}{sin(r)} = \mu \\ & \implies \frac{sin(90°)}{sin(r_{1})} = 1.5 \\ & \implies \frac{1}{sin(r_{1})} = 1.5 \\ & \implies r_{1} = sin^{-1}(1/1.5) \\ & \implies r_{1} \approx 41.8103149° \end{align}
[Which is exactly equal to the critical angle for glass and air, in accordance with the principle of reversibility of light.]
$\therefore ∠OBC = ∠OCB \approx 41.81°$
But this wasn’t our true objective was it? We wanted the light to get totally internally reflected, but for that, $r_{2}$ must be greater than $90°$. Hence $i_{2}$ must be greater than $41.81°$ (because $i \propto r$, by Snell's law), which implies that $r_{1}$ must be greater than $41.81°$ (because $r_{1}$ = $r_{2}$), which means that $i_{1}$ must be greater than $90°$ (again, because $i \propto r$). That is impossible, unless the source be in the glass itself, thus proving our observations.
I would need to go through the details but I guess something like this is possible. It is a simple type of ring cavity. In practice the number of round trips that light makes in this cavity (the cavity finesse) will be limited by two things.
- Energy lost to absorption in the glass and
- If there are any surface impurities (scratches etc.) where the light hits the surface of the glass then some fraction of light will scatter and transmit out of the glass at those points.
The light ray, under the conditions mentioned by you, will not be able to get refracted such that the angle of refraction is $45°$.
You say that the critical angle is less than $45°$. Let the refractive index of glass be represented by $U$. Then, $U = \csc{C}$, where $C$ is the critical angle
If $C < 45°$, then the Refractive Index will surely be greater than $\sqrt{2}$ or $1.41$.
We know by Snell's law that the ratio between the sine of the angle of incidence and the sine of the angle of refraction equals the Refractive Index for the given pair of media.
In such a case where $\sin{i} = U × \sin{r}$ (where i is the angle of incidence and r is the angle of refraction), if $\sin{r} = 1/\sqrt{2}$ and U is anything greater than $\sqrt{2}$, $\sin{i}$ becomes greater than $1$ which is not possible for any value of $i$.
Thus light can't be trapped in the circular prism. Consider the limiting condition when the light is incident tangent to the circular disc when the refractive index is $U = \sqrt{2}$ and the critical angle is equal to $45°$.
Clearly the light leaves the prism without getting reflected as the critical angle equals the angle of incidence inside the prism.
-
$\begingroup$ Correct me if I am terribly mistaken, but I think you are wrong in considering the value of the refractive index. Since the light is moving from the glass to air, its RI will always be lesser than (or equal to) 1. Velocity of light in glass / velocity of light in air < 1 (and hence < √2). $\endgroup$– HerrAlvéCommented Mar 6 at 16:15
-
1$\begingroup$ In both the formulas I used (Snell's law and. U=cosec C), the refractive index used is the absolute refractive index of the denser medium with respect to the rarer one which is called the refractive index of the medium (here glass) (it is the velocity of light in the air divided by the velocity in glass). The RI is for light travelling from air to glass in both the formulas. $\endgroup$ Commented Mar 6 at 17:15
-
1$\begingroup$ As it’s currently written, your answer is unclear. Please edit to add additional details that will help others understand how this addresses the question asked. You can find more information on how to write good answers in the help center. $\endgroup$– Community BotCommented Mar 6 at 18:09
I believe that is impossible for two reasons: 1, there would be energy loss due to heat, which would just turn the photons into thermal energy, and reason 2, to prevent light escaping from the disk, you would have to close where you got the light in from faster than the speed of light.
-
2$\begingroup$ The OP has commented that "It (the light) enters the prism by refraction and not through a hole." Moreover, if the light got in through a hole instead of refracting in, reason 2 would still not make it impossible, because the glass could be made with its circumference equal to a light-hour, suppose, which would be more than sufficient time to cover up the hole. Is it practical? Absolutely not. But is it impossible? No. Also, "turn the photons into thermal energy" might need a rephrasing. $\endgroup$– HerrAlvéCommented Mar 7 at 11:06