4
$\begingroup$

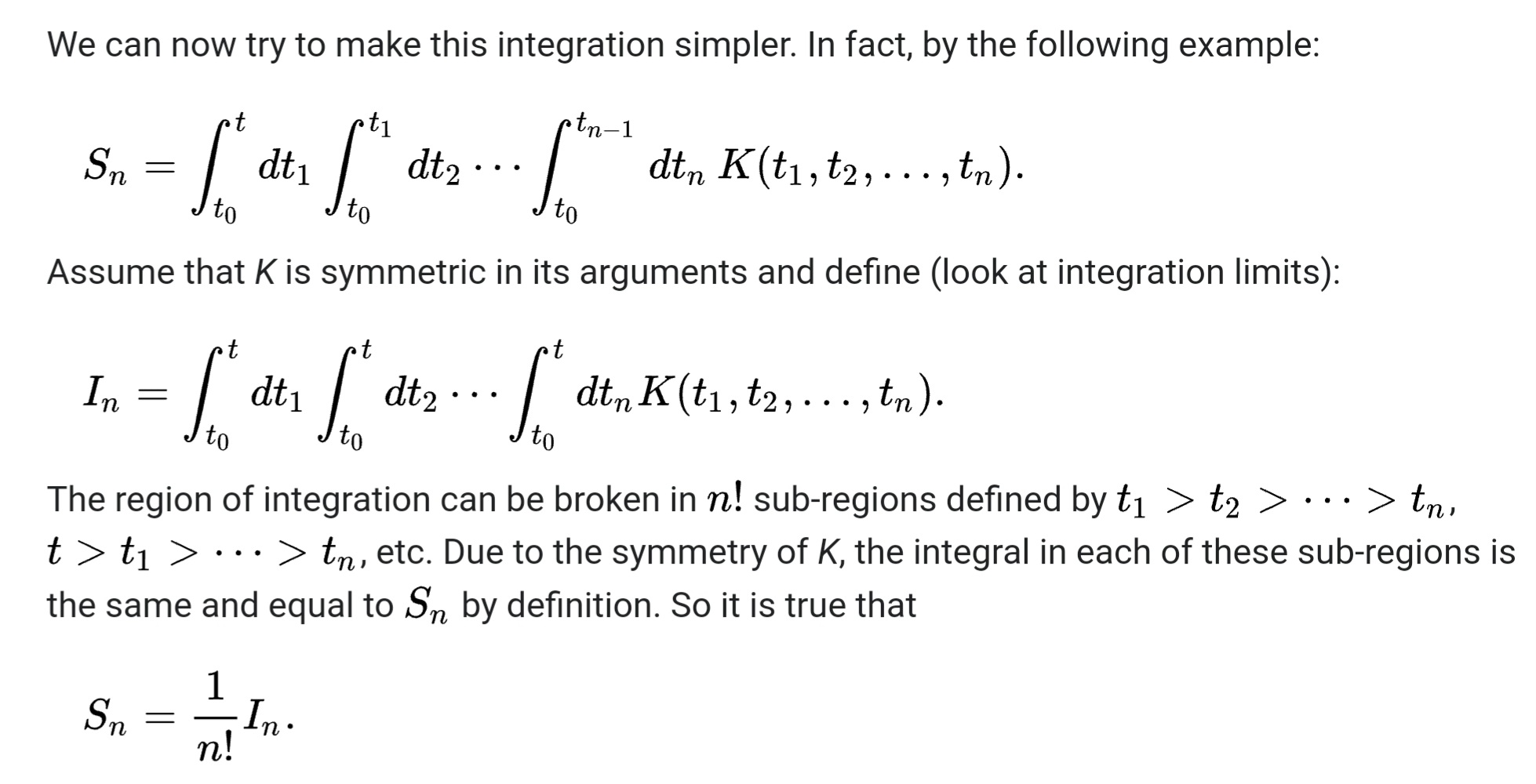
This is the explanation from Wikipedia: enter image description here

Is there a more rigorous proof or explanation of how reducing the integration region to these sub-regions introduces a $\frac{1}{n!}$ factor? I am confused about why the number of these sub-regions is $n!$ in the first place. They way the sub-regions are defined it would seem as if there are $n$ of them.

The $\frac{1}{n!}$ factor and the fact that $K$ has to be symmetric suggests that the proof involves looking at different permutations of the time boundaries $t_1, t_2,$ etc. However, the proof given here (and other proofs I've found) never show this step explicitly.

Improve this question
$\endgroup$

1 Answer 1

Reset to default
10
$\begingroup$

There are $n$ time variables. Each way of ordering the times is a permutation of the $n$ variables. There are $n!$ permutations of $n$ time variables ($n$ ways to choose the highest time, $n-1$ ways to choose the second highest time, etc.).

Improve this answer
$\endgroup$
5
  • $\begingroup$ I see. Now I understand it intuitively, but how do we rigorously prove that the n! number of such integrals S_n is equal to I_n? $\endgroup$
    – pll04
    Commented Feb 27 at 16:14
  • $\begingroup$ @pll04 Have you done it for $n=2$? $\endgroup$
    – Tobias Fünke
    Commented Feb 27 at 16:31
  • $\begingroup$ @TobiasFünke Yes, by setting K(t_1,t_2) = 1. But I couldn't work out a proof for general n, nor for integral with a general symmetric K. $\endgroup$
    – pll04
    Commented Feb 27 at 16:45
  • 1
    $\begingroup$ @pll04 Since you've done it for $n=2$, repeat for $n=3$. That'll give you a feel for using induction to do $m=n+1$, and then you're set. $\endgroup$
    – rob ♦
    Commented Feb 27 at 17:10
  • $\begingroup$ @rob I see, thanks. But how can we extend this to general cases where K isn't 1? $\endgroup$
    – pll04
    Commented Feb 27 at 17:19

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.