Dynamics
The object of mass $m$, and mass movement of inertia (sphere) of ${\rm I} = \tfrac{2}{5} m R^2$ has the following momentum values
$$\begin{aligned}
\vec{p} & = m \vec{v} & & \text{linear momentum} \\
\vec{L} & = {\rm I}\, \vec{\omega} & & \text{angular momentum} \\
\end{aligned} \tag{1}$$
where $\vec{v}$ is the velocity vector of the center of mass, and $\vec{\omega}$ is the rotational velocity vector of the body.
Note for a general shape $\rm I$ is a 3×3 matrix, and ${\rm I}^{-1}$ the matrix inverse.
Since Newton's 2nd law connects external forces/torques applied to a body, to the changes in its momentum with
$$ \begin{aligned}
\vec{F} & = \tfrac{\rm d}{{\rm d}t} \vec{p} & & \text{net force} \\
\vec{\tau} & = \tfrac{\rm d}{{\rm d}t} \vec{L} & & \text{net torque} \\
\end{aligned} \tag{2}$$
we track the body's momentum state through time, and we extract the velocity by reversing (1) as
$$\begin{aligned}
\vec{v} & = \tfrac{1}{m} \vec{p} & & \text{velocity} \\
\vec{\omega} & = {\rm I}^{-1} \vec{L} & & \text{rotation}\\
\end{aligned} \tag{3}$$
Kinematics
Suppose the ball is sliding on a horizontal surface with normal direction vector $\hat{n}$ pointing upwards against gravity. In that case, the ball's velocity at the contact point is restricted to be in-plane only.
$$ \hat{n} \cdot ( \vec{v} + R \hat{n} \times \vec{\omega}) = 0 $$
simplified to
$$ \hat{n} \cdot \vec{v} = 0 \tag{4}$$
which is intuitive as the velocity of the center of mass must be in-plane also.
Friction
Sliding friction acts in a direction opposite of the relative slipping of the contact, and in this case
$$ \vec{F} = -\mu m g \frac{\vec{v}+R\hat{n}\times\vec{\omega}}{\|\vec{v}+R\hat{n}\times\vec{\omega}\|} $$
and corresponding equipollent torque
$$ \vec{\tau} = \vec{F} \times \hat{n} R $$
At this point, it might be worth establishing some direction vectors, and looking at these relationships in terms of the vector components along these directions.
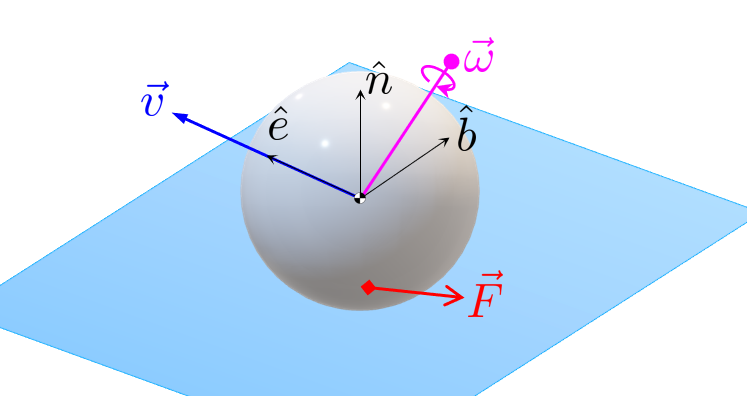
At any time frame, the direction of travel is designated $\hat{e}$ and the perpendicular direction is $\hat{b}$. By definition $\vec{v} = v \hat{e}$ at all times. Decompose the angular velocity vector into three components, denoting the roll, spin, and yaw as
$$ \vec{\omega} = \omega_{\rm spin} \hat{e} + \omega_{\rm roll} \hat{b} + \omega_{\rm yaw} \hat{n} $$
and calculate the velocity of the contact point
$$ \vec{v} + R \hat{n} \times \vec{\omega} = ( v + R \omega_{\rm roll}) \hat{e} - (R \omega_{\rm spin}) \hat{b} \tag{5}$$
This makes the sliding frictional force equal to
$$ \vec{F} = -\mu m g \frac{ ( v + R \omega_{\rm roll}) \hat{e} - (R \omega_{\rm spin}) \hat{b}}{\sqrt{ ( v + R \omega_{\rm roll})^2 + (R \omega_{\rm spin})^2}} \tag{6}$$
This illustrates the difficulty here because the direction of $\vec{F}$ depends on $\omega_{\rm spin}$. We see this by calculating the torque due to friction
$$ \vec{\tau} = -\left( \frac{\mu m g R^2 \omega_{\rm spin}}{\sqrt{ ( v + R \omega_{\rm roll})^2 + (R \omega_{\rm spin})^2}} \right) \hat{e} - \left(\frac{\mu m g R ( v + \omega_{\rm roll}R)}{\sqrt{ ( v + R \omega_{\rm roll})^2 + (R \omega_{\rm spin})^2}} \right) \hat{b} \tag{7}$$
and since $\vec{\tau} = {\rm I} \tfrac{\rm d}{{\rm d}t} \vec{\omega}$ we can find the change in rotation as
$$\begin{aligned}
\tfrac{\rm d}{{\rm d}t} \omega_{\rm roll} & = - \frac{5 \mu m g (v+R \omega_{\rm roll})}{2 R \sqrt{ ( v + R \omega_{\rm roll})^2 + (R \omega_{\rm spin})^2}} \\
\tfrac{\rm d}{{\rm d}t} \omega_{\rm spin} & = - \frac{5 \mu m g \omega_{\rm spin}}{2 \sqrt{ ( v + R \omega_{\rm roll})^2 + (R \omega_{\rm spin})^2}} \\
\tfrac{\rm d}{{\rm d}t} \omega_{\rm yaw} & = 0
\end{aligned}$$
In summary, due to the nature of friction, it is not possible to integrate the above system into a later time frame and predict analytically the motion of the ball.

