If a passing star can jostle comets in the Oort Cloud, why doesn't the Moon disrupt the orbits of high-flying satellites?
Or does it? Maybe the satellites need periodic course corrections?
$\begingroup$
$\endgroup$
4
-
6$\begingroup$ It's usually recommended to wait a while upon receiving an answer, to see if a better one comes along. While Thomas' numbers are correct, his conclusion is not as accurate as Matt's $\endgroup$– Cort AmmonCommented Feb 19 at 0:50
-
1$\begingroup$ see also: space.stackexchange.com/questions/12528/… The effect of Sun and Moon can be clearly seen in satellites that have been inactive for a decade or more. $\endgroup$– asdfexCommented Feb 19 at 12:23
-
2$\begingroup$ A pro tip for your future questions: a question which presupposes a falsehood is hard to answer. The question "does the Moon significantly affect orbits of man-made satellites?" has a yes or no answer, so ask that question. $\endgroup$– Eric LippertCommented Feb 20 at 3:21
-
$\begingroup$ "If a passing star can jostle comets in the Oort Cloud" - is this in reference to the nemesis theory en.wikipedia.org/wiki/Nemesis_(hypothetical_star)? Just in case it is, although many of us were taught this in school as an exciting and very likely hypothesis, in the words of a NASA news release, "recent scientific analysis no longer supports the idea that extinctions on Earth happen at regular, repeating intervals, and thus, the Nemesis hypothesis is no longer needed." That's in addition to a wide range of sizes, temperatures, and distances for Nemesis being ruled out in searches. $\endgroup$– AXensenCommented Feb 20 at 4:47
Add a comment
|
4 Answers
$\begingroup$
$\endgroup$
1
The moon absolutely disrupts the orbit of a geostationary satellite. Just as the moon can cause tides on the Earth's surface, the moon's gravitational pull on a satellite will cause it to begin to drift slightly north/south in its orbit. Geostationary satellites must actively counteract this if they wish to remain geostationary.
See this page for more info https://www.satsig.net//satellite/inclined-orbit-operation.htm
-
$\begingroup$ Satellites are (usually) spacecrafts! $\endgroup$ Commented Feb 28 at 17:35
$\begingroup$
$\endgroup$
6
The moon will always "perturb" the orbit to some extent, but to "disrupt" an orbit is to kick the satellite out of orbit. And this will only happen if the orbit is very wide, approximately when it reaches the Lagrange point L1, at about 85% the Moon's distance! Here's a drawing clearly showing stable and disrupted orbits: (replace Sun-Earth system with the Earth-Moon)
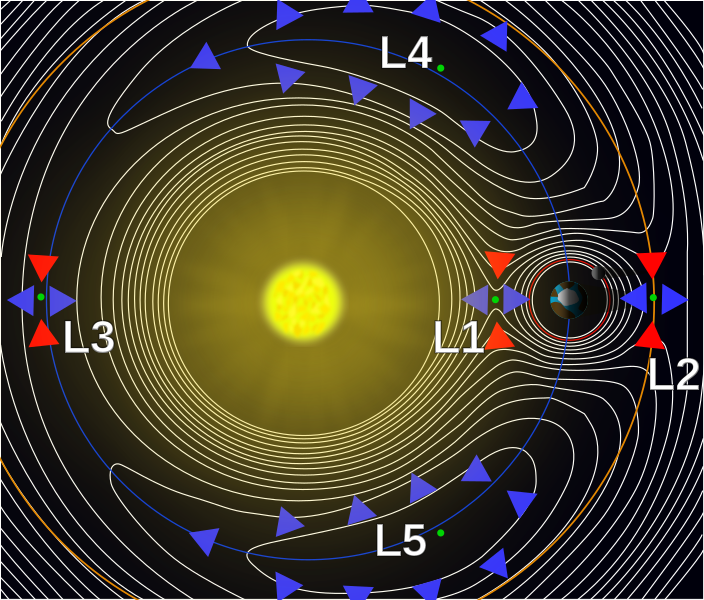https://en.wikipedia.org/wiki/Lagrange_point#/media/File:Lagrange_points2.svg
The drawing also shows that things are much less favorable for orbits around the smaller mass, i.e. a satellite around the Moon instead of Earth. More precisely, the region around the Moon where those orbits would still be stable is the so-called Hill sphere: $$ R_H= (e-1) \ a \ \left(\frac{m_2}{m_1+m_2}\right)^{1/3} $$ but with masses $m_1$ and $m_2$ for the Earth-Moon system (and $e$ and $a$ the exentricity and semi-major axis) that gives almost the same result as just using the L1 position.
answered Feb 19 at 9:05
-
3$\begingroup$ These stability considerations are nearly irrelevant to the question at hand. First, note that geostationary orbit is 35Mm, and the moon is 240Mm from the earth. So we're not talking about satellites sitting in the Lagrange points between the earth and the moon. Their orbits are, to a mathematician, "unstable" but only in the sense that the frequency changes with distance so small perturbations in distance will grow over time. And the hill sphere is another entirely irrelevant consideration. All of these satellites are well within the influence of the earth and not within the Moon's orbit. $\endgroup$– AXensenCommented Feb 20 at 4:40
-
3$\begingroup$ The question was "why doesn't the Moon disrupt the orbits" and the reason is indeed (as you correctly claim) that geostationary orbit is something entirely different than a far-out orbit which can be disrupted. In my view the answer explained just that! In your view the answer is irrelevant. How can we explain this paradox?! $\endgroup$ Commented Feb 20 at 8:01
-
1$\begingroup$ I guess my issue is that you chose to define "distrupt" as the specific kind of instability we get at L1,2,3. It would be like if someone asked "is the earth's orbit perfectly circular" and I responded "it isn't hyperbolic so yes it is circular" instead of "it is elliptical with eccentricity 0.02." The most upvoted answer points out that satellites do need course corrections because of the moon's influence, which is how the question defined "disrupt." So your answer seems to amount to "well it isn't at L1,2,3, so it's not that bad." Which says very little about the actual effect the moon has $\endgroup$– AXensenCommented Feb 20 at 19:51
-
$\begingroup$ No, I absolutely did not "choose to define" disruption as the kind of instability we shoulld talk about. That choice was made by OP, it is even in the title: "Why doesn't the Moon disrupt the ..." So you really are complaining to the wrong person... $\endgroup$ Commented Feb 20 at 23:56
-
2$\begingroup$ OP gave two descriptions of "disruption." The first is the perturbations to oort cloud comets from passing stars, which is unrelated to Lagrange points (the sun and passing stars are not in an orbit) . The second is "needing course corrections." You talked Lagrange point instability, which is not useful for understanding the actual effect the moon has on satellites. You answer is "well here's one kind of disruption that doesn't happen in this case." So I stand by my comments. But I can see that this conversation is not being productive, and you don't see my criticism as correct. $\endgroup$– AXensenCommented Feb 21 at 0:53
$\begingroup$
$\endgroup$
6
The highest-flying satellites orbit the earth at a distance of $42000$ km (the geostationary orbit) away from the center of the earth.
Remember the gravitational force is $F\propto \frac{M}{r^2}$.
Thus the force of the moon (mass $M=7.3\cdot 10^{22}$ kg, distance $r=380000$ km) on the satellite is very much smaller than the force of the earth (mass $M=6.0\cdot 10^{24}$ kg, distance $r=42000$ km) on the satellite. Using these numbers you find the force by the moon is around $10^{-4}$ times smaller than the force by the earth. So the effect of the moon is too small to significantly disrupt the satellite orbit around the earth.
The situation is different when a star moves through the Oort cloud. The star and the sun have similar masses, and they are similar distances away from the comets. Hence the forces by star and sun acting on a comet would be of similar size.
answered Feb 18 at 22:23
-
3$\begingroup$ " is too small to disrupt" maybe since it is the chosen answer you should qualify this with "significantly" ? "easily corrected" ? $\endgroup$– anna vCommented Feb 19 at 6:51
-
12$\begingroup$ I think this answer glosses a bit over the fact that in space there is no friction (or very little friction), so small accelerations can add up over time. Maybe $10^{-4}$ is enough to cause some deviations to the orbit $\endgroup$ Commented Feb 19 at 8:38
-
19$\begingroup$ After 1000 rotations, roughly three years, a $10^{-4}$ correction to the force would have a cumulative effect of 10%. Surely not negligible. $\endgroup$– JavierCommented Feb 19 at 13:18
-
9$\begingroup$ This answer is wrong about the significance of the forces and about the distances involved in Oort cloud interactions. Stars do not "move through" the Oort cloud. Proxima Centauri is the closest star to the sun at 4.25 light years away while the maximum extent of the Oort cloud is <0.08 light years. $\endgroup$ Commented Feb 19 at 16:24
-
2$\begingroup$ @DougLipinski The question and my answer is not about present-day neighbor stars (like Proxima Centauri), but about hypothetical stars actually passing the Oort cloud, like for example Scholz’s Star Disturbed Oort Cloud Objects 70,000 Years Ago. $\endgroup$ Commented Feb 19 at 19:05
$\begingroup$
$\endgroup$
3
The moon bounces around all over the place--it never hits the same apogee or perigee twice. What you see published are only averages, and maxima and minima figures derive from computer simulations that can predict (ahead and backward) about 5ky. In spite of this chaos LLR (lunar laser ranging) purports to measure the rate at which the moon is receding due to tidal drag--this must require considerable averaging and modeling over many years.
The point being, it's a lot easier to simulate lunar attraction on satellites than to measure the rate of lunar recession.
-
$\begingroup$ Welcome! I've removed a "signature" from the end of your answer. Your username appears under each of your posts; we discourage additional signatures or taglines. $\endgroup$– rob ♦Commented Feb 21 at 4:40
-
2$\begingroup$ Note that state-of-the-art lunar laser ranging measures the distance to the various lunar retroreflectors to sub-centimeter precision. There are corrections to the Moon's orbit due to general relativity of order 10 meters. Observing an orbital drift of several centimeters per year requires less averaging than you seem to think. $\endgroup$– rob ♦Commented Feb 21 at 4:45
-
2$\begingroup$ This doesn't seem to address the question. $\endgroup$– SchwernCommented Feb 21 at 22:53