1
$\begingroup$

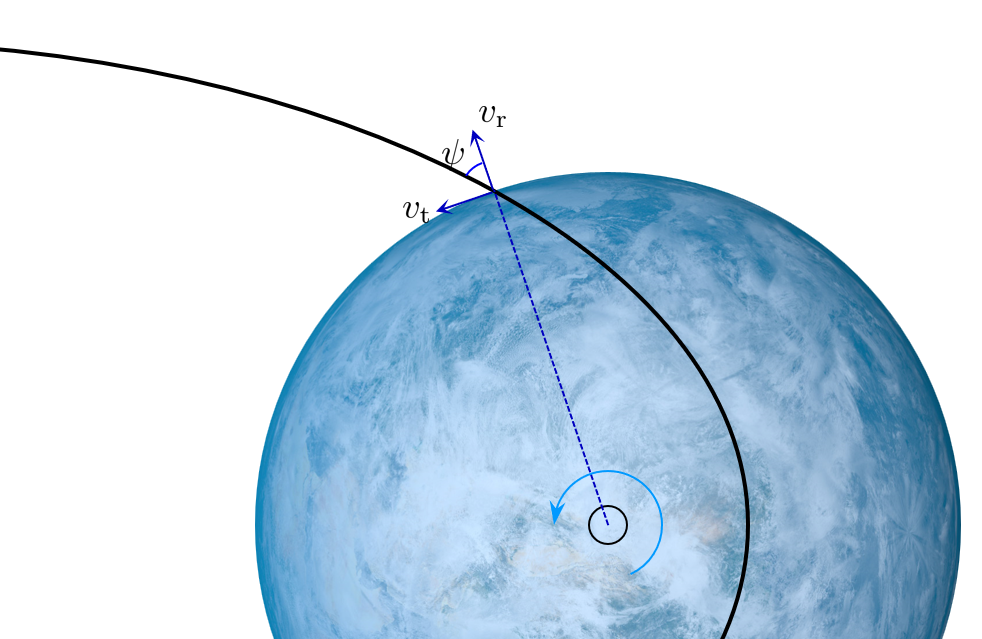
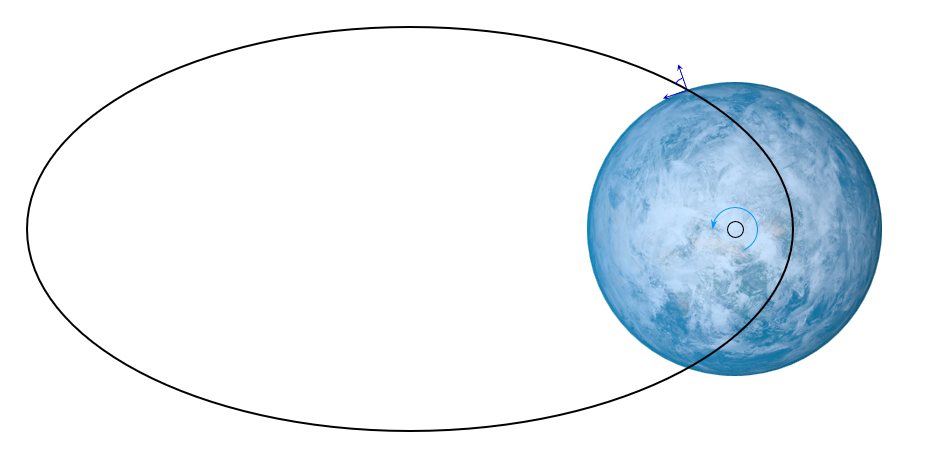
Last night I couldn't sleep for some time because of thinking this problem. The starting point of this problem was actually "If we were to jump perfectly vertically on earth, would we land on the same spot as we jumped?". However, I would like to ask a simplified version:

Suppose that there is a spherical rotating body of mass, which I assume it to be a planet, and an object on the surface of planet. Now, at some point this object is thrown vertically outwards the planet (90° angle between velocity vector and the planet surface) with some initial velocity.

I would like to know the distance between the thrower and the thrown object when it lands on this planet after it is thrown. If there is a minimum velocity which this object does not land on the planet after thrown, it would be awesome as well!

Note: My major is not in physics and its been a while since I have worked on these types of problems, so it would be helpful if you can explain the path to equations, derivations in more detail.

Improve this question
$\endgroup$
4
  • $\begingroup$ There is an assumption that the mass of the planet is much bigger than the object, and thus the planet does not move when the object is thrown upwards. Is this correct? $\endgroup$
    – John Alexiou
    Commented Feb 12 at 18:13
  • 1
    $\begingroup$ Commenting because when I saw this post, I had a textbook already in front of me open to a page declaring: "One of the Aristotelian arguments against a rotating Earth was that objects on the Earth's surface would be flung off it. Galileo argued that this conclusion was not valid, because the Earth's 'gravity' overwhelmed this centrifugal tendency!" (Analytical Mechanics, Cassiday & Fowles) Just throwing out here that the question keeping you from sleeping has kept other minds from sleeping over the centuries! There is a good answer to it; you can see Cassiday & Fowles' book, e.g. $\endgroup$
    – David C.
    Commented Feb 12 at 18:15
  • $\begingroup$ @JohnAlexiou Yes, we can say that. Similarly, effect of Newton's third law on the planet is neglected when throwing the object. $\endgroup$
    – CuriousCrypto
    Commented Feb 12 at 18:49
  • $\begingroup$ @DavidC. So wait another thing is... if we were to keep all things constant of a planet and gradually increase the angular velocity (as well as linear velocity, as an effect of this), eventually gravity will not be able to "overwhelm this centrifugal tendency"? So does this also mean that a maximum linear velocity of a planet in space is a constant maximum in terms of its other attributes? $\endgroup$
    – CuriousCrypto
    Commented Feb 12 at 20:27

1 Answer 1

Reset to default
0
$\begingroup$

So the orbit of a free object under the influence of gravity from a planet is that of an ellipse. It is a requirement that one of the focal points of the ellipse is at the center of the planet.

Now one of the features of an ellipse is that it always loops back on itself, so any ellipse that starts from the surface of the planet will intersect the planet at some point in the future always. So no you can't shoot yourself into space and become a satellite from level ground without any additional thrust like from a rocket.

From an observer far away, the moment you leave the ground, you have some defined radial velocity (upwards) depending on how much you jump, and a defined tangential velocity (sideways) depending on the rotation rate and radius of the planet.

This is the reason they prefer to launch rockets near the equator since it requires less fuel to get to orbit as the spin of the earth help in gaining the required tangential velocity.

See to be in orbit, you don't just need to go up, but also to go sideways by a very large velocity. Something like 18 km/s maybe.

Consider the example below where the launch angle is approximately 45° (radial velocity ≈ tangential velocity)

fig1

when you zoom out, you will see how the orbit is going to crash back down to the planet

fig2

I used the actual focal point of the ellipse and placed the planet center there.

Improve this answer
$\endgroup$
1
  • $\begingroup$ Do they hold for a planet with any rotational velocity or mostly for Earth? As "..to be in orbit, you don't just need to go up, but also to go sideways by a very large velocity. Something like 18 km/s maybe..." seems to suggest that it would be possible if the new linear velocity is "18 km/s + linear_velocity" given all other attributes of the said planet is kept constant. $\endgroup$
    – CuriousCrypto
    Commented Feb 12 at 20:17

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.