I am trying to understand momentum is conserved after collision between a point mass and a rotating object. I understand that during a collision between two point mass, momentum is conserved. Also that when a collision occurs with rotating objects (for example when a still cylindre is dropped on a rotating cylindre) angular momentum is conserved. But I don't understand what happen when the momentum of a point mass is converted into angular moment.
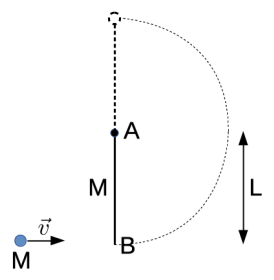
For example, let's take a point mass moving towards a still pendulum. After a perfectly inelastic collision, both the point mass and pendulum rotate around A with an angular velocity $\omega$. In this case, I don't understand if the angular momentum is conserved or if it is the linear momentum. Apparently, not both as when I compute both, I get a different equation.
Here I consider linear momentum conservation, computing the linear momentums of the mass centers after the collision :
$$M_{point} v = M_{point} \omega L + M_{bar} \omega \frac{L}{2}$$ $$=>$$ $$M_{point} v = L \omega \left(M_{point} + \frac{1}{2}M_{bar}\right)$$
And if I try with angular momentum conservation, computing an initial angular momentum as if the point mass is rotating around A instantaniously before the collision.:
$$M_{point} L^2 \frac{V}{L} = \left(M_{point} L^2 + \frac{1}{12} M_{bar} L^2 + M_{bar} \left(\frac{L}{4}\right)^2\right) \omega$$ $$=>$$ $$M_{point} v = L \omega \left(M_{point}+\frac{1}{3} M_{bar}\right)$$
So which is right (if any) ? And why ?