Work done by friction on an incline surface of random geometry

Question

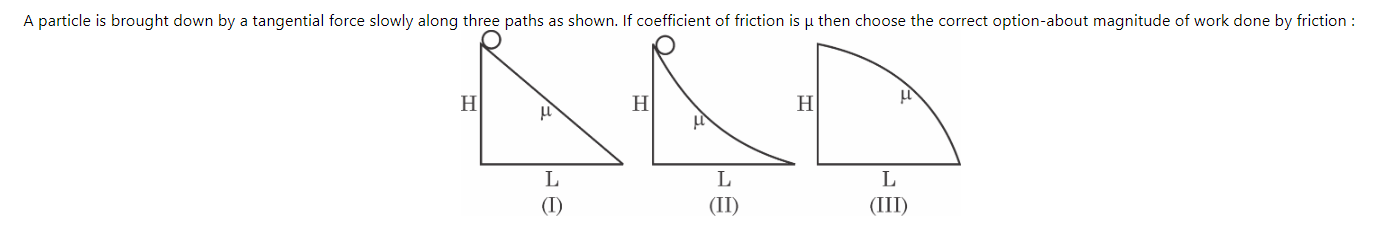
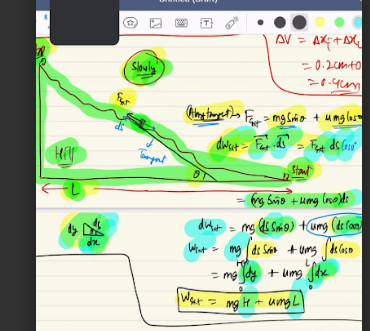
And, here's the solution my teacher gave: read from top to bottom (and note that the angle $θ$ is the instantaneous slope at that point) and ignore the green highlighting, I apologize for the poor picture quality.

According to his answer, no matter what the path of the particle is, as long as it is acted upon by an external agent that continuously applies a force tangential to the local curvature of the incline without changing the kinetic energy of the particle, the work done by friction remains constant. In this sense, friction almost behaves like a conservative force since its work only depends on the height and the length of the distance covered by the incline, irrespective of the path taken.

Firstly, I would like to verify whether this solution is correct because when I looked at similar problems, the answer only accounts for the potential energy change of $mgH$ and does not have the extra term $μmgL$ in some places, whereas in other problems, the work done by friction appears to be proportional to the actual distance the particle traverses. Can these answers be correct as well? If not, then how would the question have to be modified to make these answers correct?

My second broader question is based on the conservative behavior of friction in this scenario;

Is there perhaps a category of forces that are "pseudo-conservative" i.e. behave conservatively under special circumstances, and if so, can you give an example? Alternatively, are all non-conservative forces capable of behaving conservatively under the correct set of circumstances? Could you again give an example, perhaps with regard to the magnetic force?

Answer 1

The work done by friction will be constant given that the gravitational acceleration is constant for the height given. Actually in this case it is constant because it is a special case where the two paths are somewhat identical and symmetric. The first path is straight so we need not concern about it. The second path is a smooth curve symmetric about it's mid-point. The third path is nothing but just the second path turned inside out. We will take three points on all the three paths.

$(1)$ (The topmost point) The particle is present at the topmost point. In the first path, the normal force which will cause friction is $mgcos(\theta)$ where $\theta$ is the angle of inclination. For the second path, the tangent is very less inclined with vertical, so the normal force will be quite less and also friction will be very less. For the third path, we see that the tangent is inclined heavily on the horizontal which makes the normal force larger and hence also the friction that is acting.

$(2)$ (The mid point) Given that the curves are symmetric, we see that the directions of the tangents to both the curves is the same as the inclination of the straight path. This means same normal is acting along the three paths and hence the same frictional force.

$(3)$ (The bottom point) This becomes the exact opposite of the topmost point in the sense that the tangent of the second curve becomes equal to what the tangent of the third curve was at the top point. Similarly, the tangent here of the third curve is the same as that of tangent of the second curve at the top point.

The conclusion is that the force of friction the manner in which the force of friction varies from minimum to maximum in second path is the exact opposite of how it varies from maximum to minimum in the third path while remaining constant for the straight line path. So after integration with respect to $dr$ all over the paths, we find the work done by friction to be the same. An important condition here is to have contact with the surface all the time.

For your second question, there is nothing as pseudo-conservative. If, on a horizontal line, the coefficient of friction and normal reaction is maintained constant, the the work done by friction will appear to be dependent on displacement. But this does not make it a conservative force (or some imaginary pseudo-conservative). It is just the right set of conditions that make it apparent. While conservative forces are always conservative under any set of conditions.