My confusion is that the force the book experiences due to gravity is
the same force experienced by the table due to push from the book,
same as in its the same force, not just the magnitude. So there are
only three forces acting. There isn't action reaction pair for the
normal force.
They are not the same force. See the free body diagrams (FBD) for the book and table below.
The force the book experiences due to gravity is $F_1$. It is not a contact force. In Newtonian mechanics it's a force experienced due to a field, in this case the local gravitational field. The force experienced by the table due to push from the book is $F_3$. It is a contact force (the result of electrical forces between atoms). The two forces happen to have the same magnitude only because the book is in equilibrium.
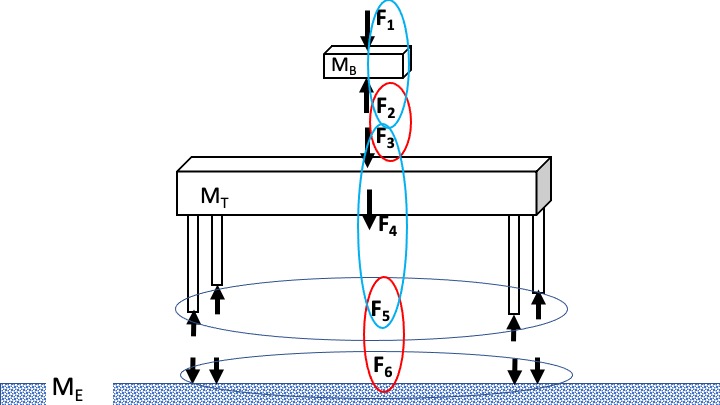
The forces shown in the FBD's are as follows:
$F_1$ = the force of gravity on the book = $M_{B}g$
$F_2$ = the normal force of the table on the book which, since the book is in equilibrium, = $M_{B}g$
$F_3$ = the normal force of the book on the table = $M_{B}g$
$F_4$ = the force of gravity on the table = $M_{T}g$
$F_5$ = the total normal force of the Earth on the table which, since the table is in equilibrium = $F_{3}+F_{4}=M_{B}g+M_{T}g$
$F_6$ = the total normal force of the table on the Earth = $F_{3}+F_{4}=M_{B}g+M_{T}g$
Not shown is the gravitational force that exists between the book and table. This force is infinitesimal compared to all the other forces.
The two Newton 3rd law equal and opposite forces pairs are $F_{2},F_{3}$ and $F_{5},F_{6}$, circled in red.
The forces acting on the book and the table for the application of Newton's 2nd law forces are circled in blue. The equilibrium requirements for the book and table are, respectively
$F_{1}+F_{2}=-M_{B}g+M_{B}g=0$
$F_{3}+F_{4}+F_{5}=-M_{B}g-M_{T}g+(M_{B}g+M_{T}g)=0$
Hope this helps.