I tried to answer the following homework problem:
I managed to answer the problem after some thinking, but I want to know the FBD of the pulleys. In a typical Atwood's Machine Problem, the two strings would be parallel to a vertical axis, and there would be two tensions exerted on the pulley at the two points of contact on either side. However, how does this change with a pulley system such as this, where the strings are perpendicular to each other.
At the moment shown in the picture, there are 4 forces on the pulley and so the FBD looks like this (for the leftmost pulleys of each pair). If you assume the pulley to be massless, you can get rid of the weight ${\bf W}$. T is the magnitude of the tension which is equal everywhere for an ideal string. The $\textbf{T2}$ is the tension due to the rope holding onto the pulley.
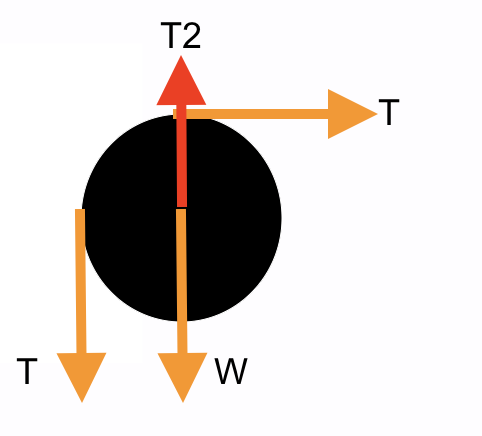
This means that there will be a net acceleration to the right (or left for the right pulleys) and the pulley pairs will come together pretty quickly and the direction of ${\bf T2}$ will change as that happens.
Sidenote: When I drew this diagram I didn't have the size of the arrows in mind but if you draw the forces to scale (as some do) then the size of the arrows for ${\bf W}$ and for ${\bf T}$ should add to that of ${\bf T2}$ here (because ${\bf T} + {\bf T2} +{\bf W} = 0$).
Answer to Lingering Question:
Thank you so much! This was a very concise and detailed response. If I may, could I just ask why the tension forces acting on the pulley point in that direction?
A rope can only pull.
If I remember correctly, there is a tension force exerted in both directions on every point of the string (if not it would accelerate). Thank you
